So I have this network in the figure below (100 MVA base) that I need to model with DC power flow line reactances and etc.
Im attempting to set up the estimation matrix and have the following, but not sure what the value for M3 and M2 would be.
M21 = 10*Theta2 - 10*Theta1 M23 = 4*Theta2 M32 = -4*Theta2
I'm thinking: M2 = M12 + M23 (should one be negative even though both are flowing out of bus 2?) M3 = M32 (do we consider M23 here at all?)
Any help is great appreciated, thank you!
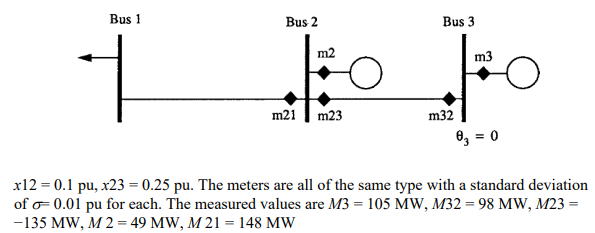
Let the (directed) active power flow at a line connecting buses \$k\$ and \$m\$ be:
$$ p_{km}=\frac{\theta_k-\theta_m}{x_{km}}$$
and denote the power injection at bus \$k\$ by \$p_k\$.
So: $$ \begin{align} m_{21} \approx& \frac{\theta_2-\theta_1}{x_{12}} =& \frac{-1}{x_{12}}\theta_1 + \frac{1}{x_{12}}\theta_2 + 0 \theta_3\\ m_{23} \approx& \frac{\theta_2-\theta_3}{x_{23}} =& 0\theta_1 + \frac{1}{x_{23}}\theta_2 + \frac{1}{x_{23}}\theta_3\\ m_{32} \approx& \frac{\theta_2-\theta_3}{x_{23}} =& 0\theta_1 + \frac{-1}{x_{23}}\theta_2 + \frac{1}{x_{23}}\theta_3 \end{align}$$ $$\begin{align} m_2 \approx& p_2 =& \frac{\theta_2-\theta_1}{x_{12}}+\frac{\theta_2-\theta_3}{x_{23}} =& \frac{-1}{x_{12}}\theta_1 +\left(\frac{1}{x_{12}}+\frac{1}{x_{23}}\right)\theta_2 + \frac{-1}{x_{23}}\theta_3\\ m_3 \approx& p_3 =& \frac{\theta_3-\theta_2}{x_{23}} =& 0\theta_1 +\frac{-1}{x_{23}}\theta_2 + \frac{1}{x_{23}}\theta_3 \end{align}$$ Then the numbers multiplying the voltage angles are the elements of your matrix so that $$\begin{bmatrix} m_{21}\\m_{23}\\m_{32}\\m_2\\m_3 \end{bmatrix} \approx B \begin{bmatrix} \theta_1\\\theta_2\\\theta_3 \end{bmatrix} $$
You should also specify a reference bus \$s\$ to have \$\theta_s=0\$, then you can remove the corresponding column of \$B\$.
I'm thinking: M2 = M12 + M23 (should one be negative even though both are flowing out of bus 2?)
I think you are making a mistake by thinking this in terms of measurements. The external injection into bus 2 (which should match the measurement M2) equals the network flows out of bus 2. So it would be better to write \$p_2=p_{21}+p_{23}\$. Note that I wrote \$p_{21}\$, not \$p_{12}\$, so net power into (or out of) the bus is zero.
M3 = M32 (do we consider M23 here at all?)
\$p_3\$ does equal \$p_{32}\$ from nodal power balance. I'm not sure what you mean by "do we consider M23 here at all?", as this is a lossless model where the flow \$p_{km}=-p_{mk}\$. You are probably asking this because you are not making a proper distinction of measurements and actual flows due to voltage angles.
