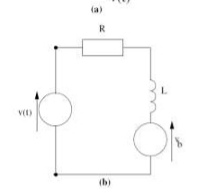
Using Faraday's law of induction, we can write:
$$-\text{V}\left(t\right)+\text{V}_\text{d}\left(t\right)+\text{R}\cdot\text{I}\left(t\right)=-\text{L}\cdot\text{I}'\left(t\right)\tag1$$
Where \$\text{I}\left(t\right)\$ is the input current (which is the same trough all the components because it is a series circuit).
Using Laplace transform we can write:
$$-\text{v}\left(\text{s}\right)+\text{v}_\text{d}\left(\text{s}\right)+\text{R}\cdot\text{i}\left(\text{s}\right)=-\text{L}\cdot\left(\text{s}\cdot\text{i}\left(\text{s}\right)-\text{I}\left(0\right)\right)\tag2$$