Finding the current trough resistor in a closed mesh

Question

In a closed mesh we have:

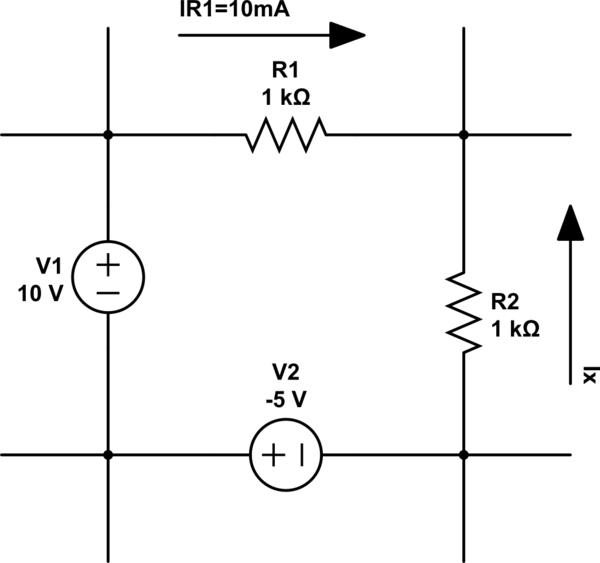
^{simulate this circuit – Schematic created using CircuitLab}

The question is to determine the current through the 2nd resistor \$\text{I}_x\$.

---

My work:

I think of two methodes:

1. Because the currents that flow into a node must add up to zero, we know that:

$$\text{I}_{\text{R}_1}+\text{I}_x=0=10\cdot10^{-3}+\text{I}_x\space\Longleftrightarrow\space\text{I}_x=-10\cdot10^{-3}\space\text{A}\tag1$$

2. The voltage across the two resistors must add up to \$10+\left(-5\right)=5\space\text{V}\$, and I lose \$10\cdot10^{-3}\cdot1000=10\space\text{V}\$ across \$\text{R}_1\$ so the thing I've left is \$5-10=-5\space\text{V}\$, so \$\text{I}_x=-\frac{-5}{1000}=\frac{1}{200}=5\space\text{mA}\$

Which of my methods is right?

Answer 1

There may be currents entering/leaving the nodes via the spurs. This doesn't matter, you don't need to find these, just solve the isolated mesh with the information given.

1. \$\small R_2\$ has \$\small 10\: V\$ across it, with + on the left and - on the right.
2. The \$\small 5\:V\$ source has + on the right and - on the left, since its voltage is -5V.
3. The voltage across \$\small R_2\$ is \$\small I_x R_2\$ with + at the bottom and - at the top.

Now you can apply KVL to find \$\small I_x\$.

Don't expect KCL to work for this mesh - you have no way of knowing what currents are flowing through the spurs.

You have the correct answer: \$\small I_x = 5\:mA\$

Answer 2

Neither of your answers is correct. If \$I_x\$ is -10mA then the total voltage drop across the two resistors is 20V and KVL is violated. If \$I_x\$ is 5mA then KCL is violated at the junction between the two resistors.

Your problem is nonsensical as shown.