I have a collection of piezoelectric transducers that I have got from old devices.
No print stamp on them, and I couldn't get a schematic of the device that could help me to find a datasheet. I wonder if I could find the model through this procedure:
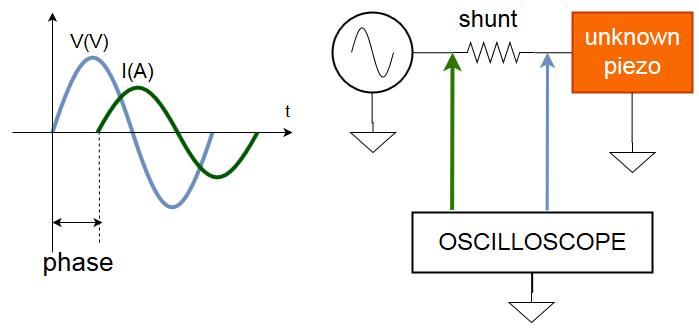
The idea is basically exciting the piezo with a known electrical signal (sine) and measuring the current and voltage in the load. By taking note of the voltage in the shunt resistor I get the current amplitude and phase.
I could say that the piezo impedance equals R+jX, where X is the reactive component.
Is there any pitfall in this idea that I should be aware of? This is the first time I try to model this piezo.
EDIT: i want the model to build a matching network EDIT: The piezos I know, usually work at a simple frequency,so I think that I just need to sample at the same frequency (no freq sweep).