I did the following calculations: Max current = 1.2A, Max voltage = 12V Then power = 14.4 watts = 0.019hp
Rpm = HP * 5252 ÷ torque I need a torque to 2kgf-cm = 0.144 lb-ft Gives rpm= 698.
Thus this means if I put load(mechanical) on the motor, it will run at 698 rpm and increase its torque to 2kg-cm?
(The motor is a high rpm motor, i.e 12000)
In a permanent magnet or shunt-wound DC motor torque is directly proportional to current, and the torque constant (Kt) is the inverse of the velocity constant (Kv). If your motor does 12000rpm at 12V unloaded, then (assuming no-load current is 'small') Kv = 1000rpm/V or 105rad/s/V. The inverse of that is 1/105 = 0.0095N-m/A or 97g-cm/A. At 1.2A it would produce 1.2*97 = 116g-cm torque.
Max current = 1.2A, Max voltage = 12V Then power = 14.4 watts = 0.019hp
That is input power, not all of which is converted to output power (rotational speed x torque). Knowing Kt and current we can calculate torque, but speed is dependent on the motor's internal resistance - which we do not know.
The generic graph below shows the relationships between power, speed, current, torque, and efficiency. As loading is increased the motor draws more current, which increases torque but reduces rpm (the blue line) due to voltage drop across its internal resistance. Peak output power occurs at 50% of no-load speed, but at that point the motor is dissipating half the input power and efficiency is only 50%.
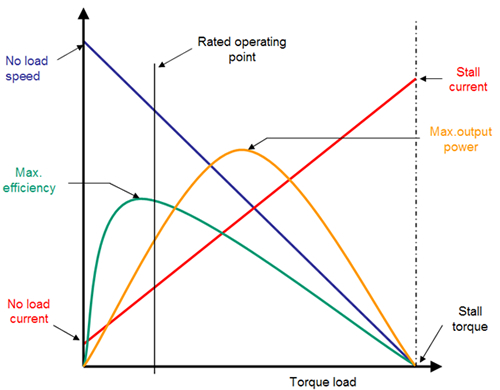
Most motors are rated for the maximum power they can run at without overheating, which is usually less than peak output power. Therefore we can assume that at rated voltage and current the output power will be at least 7.2W and the speed will be 6000rpm or higher.
But what if you increased loading beyond the motor's rating? If we assume that at rated power it is doing 6000rpm then at 0rpm it would draw twice the current and produce twice the torque, ie. 232g-cm - still way less than what you want.
However, if you gear the motor down by a ratio a 17.2:1 then 6000rpm becomes 349rpm and torque becomes 2kg-cm! (assuming 100% gearbox efficiency). Note that your motor could do significantly better than this if its rated output is at higher than 6000rpm, since it will have higher efficiency and be producing more output power.
No, of course not. The torque that a motor can generate is related to the strength of the internal magnetic fields and their geometry — especially the radius of the rotor.
The magnetic field is proportional to the current, and the max current also puts a limit on the max torque, regardless of RPM.
If you want to trade off RPM for torque without overloading the motor, you need to use a gearbox. Or pick a motor that was designed for that speed and torque combination to begin with.
The only type of motor that allows more torque at reduced speed without drawing way too much current is a DC motor with a series-connected field. All other types of motor have greatly increased current and losses as the load torque increases above the rated load torque. Even a series motor will not allow loading to anywhere near that much speed reduction.
