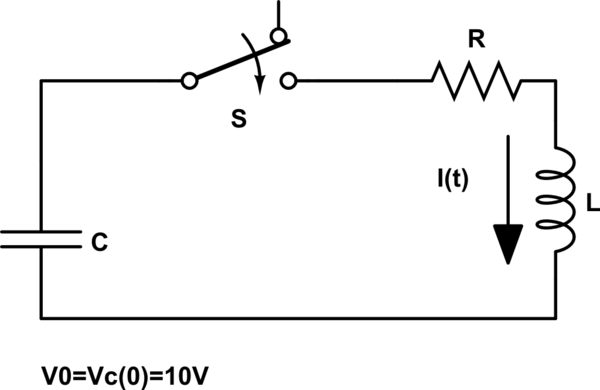
simulate this circuit – Schematic created using CircuitLab
From my book
Assume the capacitor is charged to a voltage V0, and then at t = 0, the switch is closed. \begin{gather*} \frac{1}{C}\int Idt+IR+L\frac{dI}{dt} =0\\ \frac{d^{2} I}{dt^{2}} +\frac{R}{L}\frac{dI}{dt} +\frac{1}{LC} I=0\\ \end{gather*} select
\begin{gather*} I=I_{0} e^{\alpha t}\\ \end{gather*}
substitute into the differential equation gives. \begin{gather*} \alpha ^{2} +\frac{R}{L} \alpha +\frac{1}{LC} =0\\ \alpha _{1} =-\frac{R}{2L} +\sqrt{\frac{R^{2}}{4L^{2}} -\frac{1}{LC}}\\ \alpha _{2} =-\frac{R}{2L} -\sqrt{\frac{R^{2}}{4L^{2}} -\frac{1}{LC}}\\ I=I_{1} e^{\alpha _{1} t} +I_{2} e^{\alpha _{2} t}\\ \end{gather*} In this case the constants can be evaluated from a knowledge of I(0) and dI/dt(0). Since the current in the inductor was zero for t < 0, and since it cannot change abruptly, we know that: \begin{gather*} I( 0) =0\\ \end{gather*}
The initial voltage across the inductor is the same as across the capacitor, so that:
\begin{gather*} I_{1} =-I_{2} =\frac{V_{0}}{( \alpha _{1} +\alpha _{2}) L}\\ \end{gather*} The solution for the current in the series RLC circuit is thus:
\begin{gather*} I=\frac{V_{0}}{( \alpha _{1} -\alpha _{2}) L}\left( e^{\alpha _{1} t} -e^{\alpha _{2} t}\right)\\\end{gather*} Case 1: Overdamped For R2 > 4L/C, the quantity under the square root is positive, and both values of α are negative with | α2| > |α1|. The solution is the sum of a slowly decaying positive term and a more rapidly decaying negative term of equal initial magnitude. An important limiting case is the one in which R2 >> 4L/C. In that limit, the square root can be approximated as:
\begin{gather*} \sqrt{\frac{R^{2}}{4L^{2}} -\frac{1}{LC}} =\frac{R}{2L}\sqrt{1-\frac{4L}{R^{2} C}} \approx \frac{R}{2L} -\frac{1}{RC}\\ \alpha _{1} =-\frac{1}{RC}, \alpha _{2} =-\frac{R}{L}\\ I\approx \frac{V_{0}}{R}\left( e^{\frac{-t}{RC}} -e^{\frac{-Rt}{L}}\right) \end{gather*}
How does this equation is estimated?
\begin{gather*} \frac{R}{2L}\sqrt{1-\frac{4L}{R^{2} C}} \approx \frac{R}{2L} -\frac{1}{RC}\\ \end{gather*} I think it should be right? \begin{gather*} \alpha _{2} =-\frac{R}{L} +\frac{1}{RC}\\ \end{gather*}
But if
\begin{gather*} \alpha _{2} =-\frac{R}{L}\\ \end{gather*}
Current should be \begin{gather*} I\approx \frac{V_{0} RC}{-L+R^{2} C}\left( e^{\frac{-t}{RC}} -e^{\frac{-Rt}{L}}\right)\\\end{gather*}
