0
\$\begingroup\$

The task says:

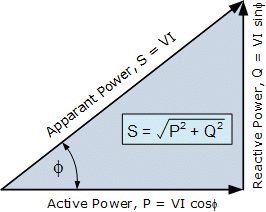
Two impedance's are added together in parallel. \$ Z_{1} = 2 - j5 (Ω) \$ ,\$ Z_{2} = 1 + j (Ω)\$ Power on \$ Z_1 = 20 W\$. Determine the reactive power.

I tried this \$P = U * I\$ => \$ P = \frac{U^2}{Z_1} \$ and that would give me the absolute value of U = 10.38 V ,after that using voltage I would find the current that goes through both Impedance's. Following that logic I would then find the current of the source and multiply that with Impedance's ( \$ 6.82\angle{-29.91} * 1.52\angle{29.93} = 61.33 - 35.25j \$ ) and that would give me the wrong answer.

Is there any tip, I think my understanding of this whole concept of power in a circuit is a bit loose, so help about what I did wrong is greatly appreciated.

\$\endgroup\$
3
  • \$\begingroup\$ Remember load is a pythagoras vector aka complex impedance and Power is the i real axis for R and j is the reactive axis for X. So use Trig. to find X \$\endgroup\$
    – D.A.S.
    Commented Jul 28, 2019 at 15:21
  • \$\begingroup\$ Maybe I was not clear sorry about that, In my language power means apparent power, real power means real power and reactive power well reactive power \$\endgroup\$
    – Gustav Robert Kirchhoff
    Commented Jul 28, 2019 at 15:28
  • \$\begingroup\$ Multiplying polar quantities means multiply the magnitudes and add the phase angles. 6.82 L-29.91 x 1.52 L29.93 = 10.37 L0.02 \$\endgroup\$
    – Chu
    Commented Jul 28, 2019 at 17:29

1 Answer 1

Reset to default
0
\$\begingroup\$

Is there any tip

If you know the power on Z1 then you know that that is dissipated by the "2" part of 2 - j5 and this leads on to be able to state the current through that 2 ohms: -

$$20 = I^2 \cdot 2$$

Hence current is \$\sqrt{10}\$ = 3.162 amps.

From that you can calculate the line voltage using Z1's impedance. That impedance is \$\sqrt{2^2+5^2}\$ = 5.285 ohms. So you have the line voltage of 17.03 volts.

Can you take it from here?

In engineering, power is real (or active) power, apparent power is volts x amps and, reactive power is \$\sqrt{(V\cdot I)^2 - (watts)^2}\$: -

enter image description here

\$\endgroup\$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.