(It has been a while since I took digital logic classes, but ...)
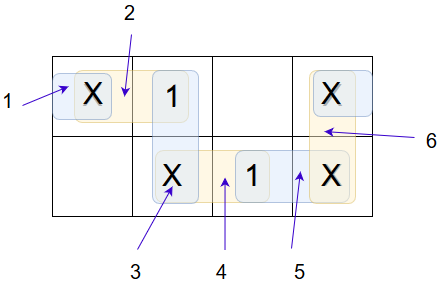
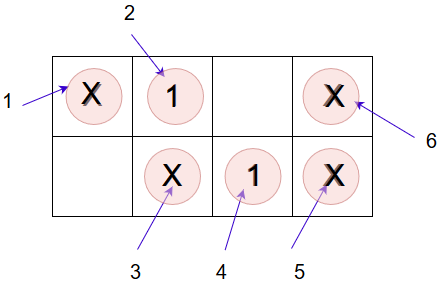
Considering that the Xs are don't-cares they do not need to be included in the minimized logic expression and are there to be used only if needed. Also, prime implicants are supposed to maximize the number of cells (1s and X) they include, that being in order to reduce the expression. I would not count the groups 1 and 6, because they only link Xs, and the single cells such as groups 2 and 4 of your first picture, are not prime implicants because they don't maximize the number of cells they group. So, counting possible implicants (though not prime implicants) I guess you cound count the two lone 1s in the first picture and the groups 2, 3, 4 and 5 of the second picture posted.