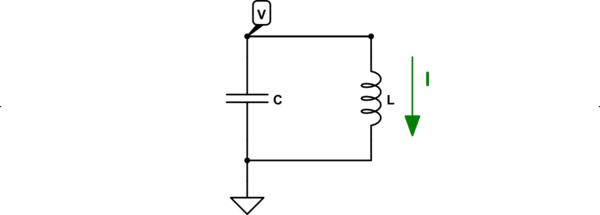
I am having some trouble understanding the behaviour of electrons inside an RC circuit. Let me explain:
- One capacitor plate accumulates a lot of electrons, which pushes electrons away into the negative/ground from the other plate.
- I disconnect the charged capacitor.
- I insert it into the LC circuit.
- Because of the attraction of the electrons on the one plate to the electron-deprived protons on the other plate, they start to "travel" in that direction through the wire.
- The electrons hit the inductor, which creates a magnetic field due to the change in current, which pushes back against the electrons, but lets some pass through.
- Now the discharge of the capacitor has completed and the magnetic field in the inductor collapses and causes electrons from the wire to be pushed towards the (previously) negative plate.
- The plates have now switched and the same amount of electrons that were on the one plate before are now on the other.
Questions:
- Regarding 4) Since there is a lack of electrons on the one plate, won't it simply attract free electrons from the wire (that is connected between the end of the inductor and the "negative" plate) to fill the "holes"?
- Regarding 5) As I understand it, there is always some electrons passing through, it's just the number that changes?
- Regarding 6) The plate that is being charged by the inductor (the initially "negtative" plate) should already have a slightly positive charge from the current that passed through the inductor while it was building the magnetic field. But this would only be true if my assumption about point 4) is true (the first question).
Could somebody try to clarify this, and tell me if my assumptions are correct?
EDIT: My main question is how the overcharge from the one plate can create an overcharge on the other. Since the amount of positive charge on the one plate of the capacitor should match the amount of negative charge on the other, shouldn't they just cancel each other out once the discharge is complete? How does the inductor in between influence the current so that it ends up in a net positive charge? Are the electrons that were displaced by the charge still there to refill the space?