0
\$\begingroup\$

In the three-phase electric power,we will assume the difference of phase between the voltage of three alternating current is 120 degree

I want to ask why do we assume the 120 degree is the best option for the difference of three voltage phase?Can it be proved with math formula ?

\$\endgroup\$
5
  • 1
    \$\begingroup\$ They're evenly spaced which makes it better for most applications. 3 x 120° = ? \$\endgroup\$
    – Spehro 'speff' Pefhany
    Commented Mar 1, 2020 at 4:36
  • \$\begingroup\$ Define what "best option" means to you. \$\endgroup\$
    – Dave Tweed
    Commented Mar 1, 2020 at 4:51
  • \$\begingroup\$ @DaveTweed If 120 degree is not the best option,why don't we use like 90 degree,45 degree? the best option means this \$\endgroup\$
    – shineele
    Commented Mar 1, 2020 at 6:35
  • \$\begingroup\$ @SpehroPefhany 360 degree is like a circle,but why do we want to let the degree become \$\frac{3}{360}\$? why don't we assume the degree become \$\frac{3}{90}\$ or \$\frac{3}{180}\$? \$\endgroup\$
    – shineele
    Commented Mar 1, 2020 at 6:36
  • \$\begingroup\$ Not generally. If you're allowed to assume an ideal system, yes, but unbalanced loads on different phases will modify that phase difference; in the real world, 120 degrees is an approximation not an exact value. \$\endgroup\$
    – user16324
    Commented Mar 1, 2020 at 12:52

1 Answer 1

Reset to default
3
\$\begingroup\$

In the three-phase electric power, we will assume the difference of phase between the voltage of three alternating current is 120 degree.

No, the phase relationship is not assumed, it is designed.

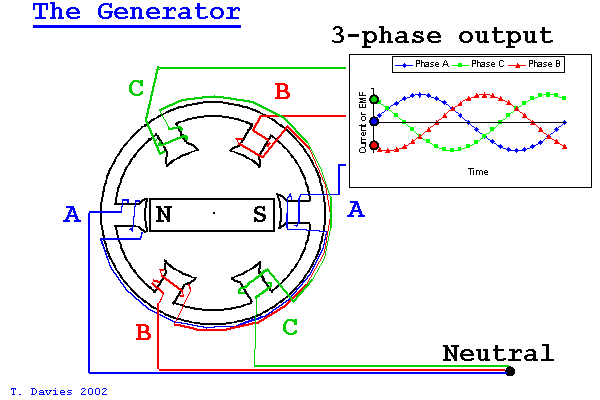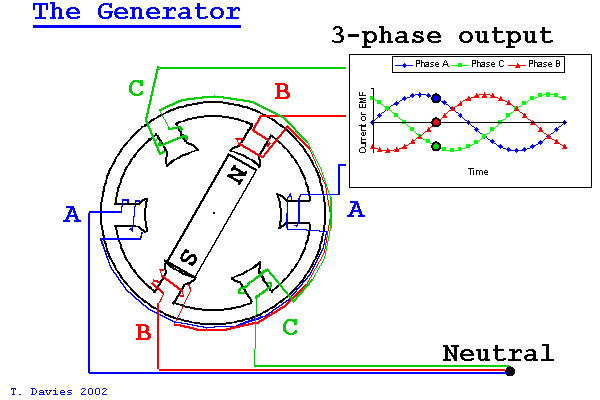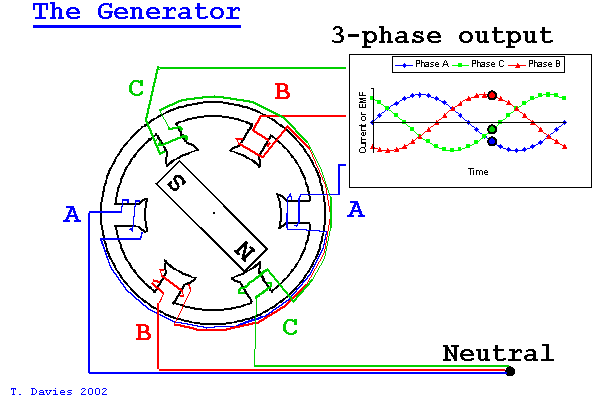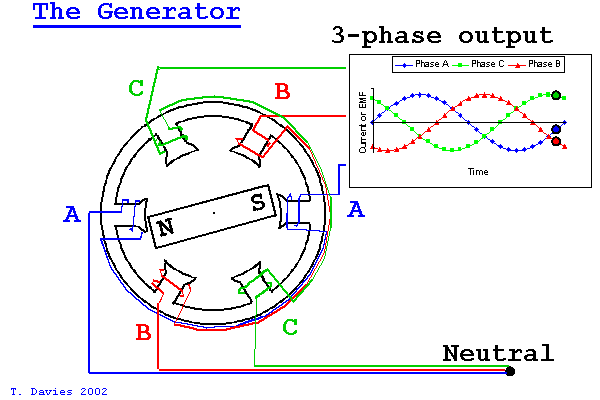
enter image description here

Figure 1. Source: TES. (Original author unknown.)

As shown in Figure 1, the poles are mechanically laid out in a 120° spacing so the voltage outputs are offset by 120°.

I want to ask why do we assume the 120 degree is the best option for the difference of three voltage phase? Can it be proved with math formula ?

The maths should be fairly straight forward. The three phases balance out when set at 120°. If you can prove that \$ sin(a) + sin(a - \frac {2}{3}\pi) + sin(a - \frac {4}{3}\pi) = 0 \$ you will have shown that the phases are perfectly balanced for all values of a. Thus the current in on any one phase is perfectly balanced by the current out on the other two. (Hence we don't require a neutral connection for a balanced load.)

\$\endgroup\$
2
  • \$\begingroup\$ @shineele, thank you for accepting my answer but I recommend that you unaccept for a day or two to give the whole of humanity - some of who are asleep right now - a chance to answer. Different answers may give you different ways of looking at the matter which will help you further. \$\endgroup\$
    – Transistor
    Commented Mar 1, 2020 at 9:35
  • \$\begingroup\$ It's also worth emphasizing that the net magnetic field produced by cables feeding a balanced load from a balanced supply (120 deg spacing) is zero. If the angles were not so then all havoc would happen when laying cables near to other wiring. +1 on the neat gif. \$\endgroup\$
    – Andy aka
    Commented Mar 1, 2020 at 11:24

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.