Is there any logic as to why the power of a signal \$\mathrm{x(t)}\$ is taken as \$\mathrm{x^2(t)}\$? I searched everywhere and there's no clue. My professor told: "It's a standard result so shut up!"
\$\begingroup\$
\$\endgroup\$
12
-
3\$\begingroup\$ It's not always triue. But if the signal is applied to a load ... and that load is a pure resistor ... then what? \$\endgroup\$– user16324Commented Sep 21, 2020 at 13:41
-
3\$\begingroup\$ That "professor" is an idiot! A signal has no power unless the signal itself is power (in the RF world this is common) but then there is always an impedance (resistor) defined into which that power goes. Your "professor" didn't mention that there needs to be a resistor for power to exist and that makes him sloppy in my opinion. \$\endgroup\$– BimpelrekkieCommented Sep 21, 2020 at 13:43
-
3\$\begingroup\$ Pretty sure he does not mean literally the power, but proportional to. \$\endgroup\$– DKNguyenCommented Sep 21, 2020 at 13:47
-
5\$\begingroup\$ @emreiris It is what their professor told them. It is not directed at SE users. OP should have put quotes around it. \$\endgroup\$– AJNCommented Sep 21, 2020 at 13:56
-
1\$\begingroup\$ @Bimpelrekkie "there needs to be a resistor for power to exist" is a bit extreme. While I understand what you mean, in general the concept of power - even in a lumped circuit - does not require any resistor. Ideally charging a capacitor will transfer energy from the ideal source to the capacitor. Is there not power involved in the process? \$\endgroup\$– Sredni VashtarCommented Sep 22, 2020 at 0:59
|
Show 7 more comments
7 Answers
\$\begingroup\$
\$\endgroup\$
1
If the signal is represented as a voltage \$v(t)\$ or a current \$i(t)\$ and it is connected to a (1 ohm) resistor, the power dissipated in the resistor is proportional to \$v^2(t)\$ or \$i^2(t)\$.
Apart from that, defining power as a positive, increasing function of the signal amplitude has useful mathematical properties.
-
2\$\begingroup\$ And if it's a different resistance, that's just a constant factor which we don't care about for signal processing purposes. \$\endgroup\$ Commented Sep 22, 2020 at 8:42
\$\begingroup\$
\$\endgroup\$
2
$$ P = \frac{V^2} R $$
If you're driving a constant resistance then the power is proportional to the square of the voltage.
You can rewrite the equation substituting, from Ohm's Law, \$ V = IR \$:
$$ P = \frac {V^2} R = V \frac V R = VI $$
and again ...
$$ P = VI = (IR)I = I^2R $$
answered Sep 21, 2020 at 13:41
-
\$\begingroup\$ Even though they're all equivalent, IMHO, one would start from \$P=VI\$ (and \$V=IR\$) and from there get to either \$P=\frac {V^2} R\$ OR \$P=RI^2\$. \$\endgroup\$– jcaronCommented Sep 22, 2020 at 14:00
-
\$\begingroup\$ I would have too if the OP hadn't started with V^2 in the question. \$\endgroup\$ Commented Sep 22, 2020 at 14:51
\$\begingroup\$
\$\endgroup\$
The signal power you are describing is not the actual power (in the conventi
There is power and energy. Energy is used to measure the signal content in a signal of FINITE duration. Power is a measure for a signal that has an INFINITE duration (sine wave etc). The signal must be periodic for this calculation to be possible.
Here is an equation to calculate power from one of my textbooks:
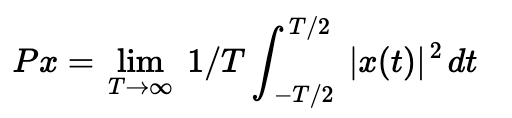
Hopefully it is clear that this is a measure of the average energy of the signal. Also note that this equation requires a period T. Therefore, the power of a signal is equal to the mean of the amplitude squared within one period.
\$\begingroup\$
\$\endgroup\$
It's a convention in signal processing theory to consider the signal being applied to a 1 Ω load, hence the expressions you were given.
My professor told: "It's a standard result..."
Your professor was strictly correct. It would have been more helpful if he or she had explained the 1 Ω convention.
If you are calculating power levels in a practical system you'll need to take account of the actual system impedance.
answered Sep 22, 2020 at 0:24
\$\begingroup\$
\$\endgroup\$
Well, if your source were to double its voltage output while driving the same unchanging load, the current would double (See Ohm's law.)
Double the voltage with double the current would heat up a resistive load by 4X, which is the square of 2. (See definition of electric power in terms of both current and voltage.)
\$\begingroup\$
\$\endgroup\$
0
A point not mentioned in other answers: the term “power” has a meaning, in physics: it means the rate of transfer of energy over time. It’s not just an arbitrarily defined quantity. So from this meaning, and a bit of knowledge of physics, we can work out what the power of a signal must be.
As mentioned in Graham Nye’s answer, there is a useful convention of considering signals as applied to a nominal 1 Ω load. Plugging this in to the equation \$P = I^2/R\$ given in Transistor’s answer for the power used by a current through a resistor, we get that the power is the square of the amplitude of the signal. (Or that the power is proportional to the amplitude of the signal, if we don’t assume the 1Ω load convention.)
\$\begingroup\$
\$\endgroup\$
Power is proportional to the square of amplitude in so many physical situations that it can be considered the normal case.
As many here have mentioned, the power into a load is proportional to the square of voltage and proportional to the square of current.
The power delivered into the air by a speaker (at a given frequency) is proportional to the square of the travel of the cone.
The power transferred from one place to another by a wave in any linear medium is also proportional to the square of the amplitude of the wave -- height for water waves, pressure for sound waves, electric and magnetic fields for radio waves, etc.
If you have some kind of signal, the odds are very good that when you translate it into something real, the power will be proportional to the signal amplitude, at least at constant frequency.
It works out this way so often that it makes sense to refer to the square of a signal's amplitude as "power", even if you don't know what it will be used for.
