If something has infinite resistance wouldn't nothing happen because everything is blocked?
I also don't understand what an "open circuit" really means (to my eye it just looks like a broken circuit that isn't going to do anything). It's all quite horribly confusing to me and all the online guides and answers just repeat the same stuff. Can anyone explain this to me like I am a dummy?
You are getting confused because you are thinking that voltage flows.
Current flows, but voltage does not flow. This means you can block a current so that the current flow is zero. But you can't block voltage, not because voltage is unblockable, but because it doesn't make sense to talk about blocking it since it doesn't flow in the first place.
It is like how you can block a ball from falling off a table, but you can't block it from having \$E=mgh\$ of potential energy while sitting on that table.
I also don't understand what an "open circuit" really means (to my eye it just looks like a broken circuit that isn't going to do anything).
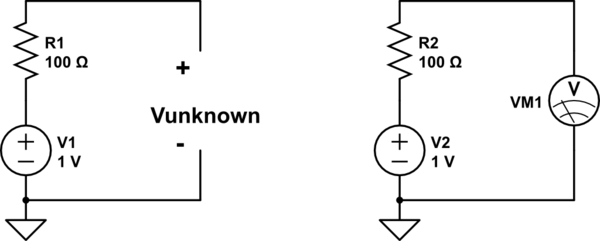
simulate this circuit – Schematic created using CircuitLab
What is the current flow in the circuit on the left? It's an open-circuit which means the circuit path is broken and not closed which means no current can flow. Agreed?
If no current can flow, what is the voltage drop across R1? \$V_{R1} = IR_{1} = 0amps \times 100 \Omega = 0V\$ Agreed?
So if the voltage drop across R1 is 0V, what is the voltage \$V_{unknown}\$? It is V1 because V1 has a voltage of 1V, but R1 has a voltage drop of 0V.
The circuit on the right is the same except we have connected a voltmeter VM1 where \$V_{unknown}\$ is so we can actually measure it. The voltmeter is designed to allow no current to flow through it so the circuit on the right is no different from the circuit on the right. In other words, the voltmeter VM1 can be thought of as an infinite Ohm resistor as far as the circuit is concerned.
Don't even worry about output resistance (and more generally, impedance) of voltage or current sources until you understand the above.
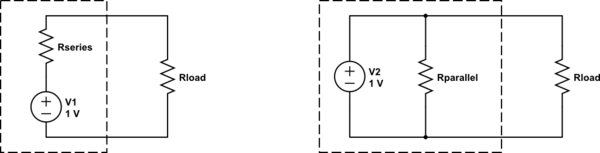
simulate this circuit
These are a non-ideal voltage source and a current source with output resistance. Everything inside the dotted box is part of the non-ideal source.
For the non-ideal voltage source, \$R_{parallel}\$ cannot influence the voltage that the ideal voltage source applies to \$R_{load}\$. The ideal voltage source simply applies its ideal voltage to both \$R_{parallel}\$ and \$R_{load}\$. So we do not talk about internal parallel resistances as an output resistance for a voltage source.
But it should be obvious that \$R_{series}\$ can interfere with the voltage that the ideal voltage source is trying to apply to \$R_{load}\$, and the voltage that \$R_{load}\$ actually gets. If current flows, then some voltage will be lost across \$R_{series}\$ and change the voltage seen by \$R_{load}\$. The more current that it needs to supply, the more the voltage drops below ideal. So when we talk about the output impedance for a voltage source, we are talking about a series resistance.
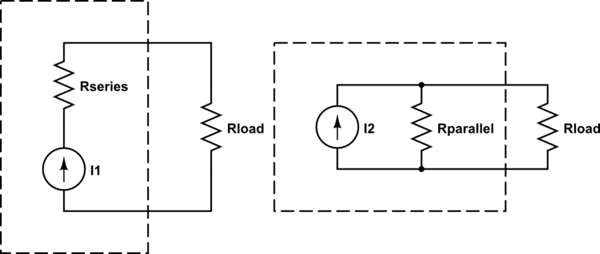
simulate this circuit
For the current source, \$R_{series}\$ cannot influence the output current. It cannot affect the current running through \$R_{load}\$. The ideal current source simply drives whatever current it wants to drive and it goes through both \$R_{series}\$ and \$R_{load}\$. Therefore, we do not talk about series resistances as an output resistance for current sources.
But it should be obvious that \$R_{parallel}\$ can disrupt the current that the ideal current source is trying to push through \$R_{load}\$, and the current that actually flows through \$R_{load}\$. Since the current source outputs a fixed amount of current, some current will be split between \$R_{series}\$ and \$R_{load}\$. That means that as more current is supplied, less and less current reaches \$R_{load}\$. So when we talk about an output resistance for a current source, we are talking about an internal parallel resistance.
Now...what do they mean when they say an IDEAL voltage source has zero output impedance and an IDEAL current source has infinite output impedance? Look at the circuits for V1 and I2.
What does \$R_{series}\$ have to make the circuit for V1 into an ideal voltage source? It needs \$R_{series} = 0 \Omega \$, ergo ideal voltage sources have zero output resistance.
What does \$R_{parallel}\$ have to make the circuit for I2 into an ideal current source? It needs \$R_{parallel} = \infty \Omega \$, ergo ideal voltage sources have zero output resistance.
If something has infinite resistance wouldn't nothing happen because everything is blocked?
So by now, you should have realized that the output resistance for a current source is considered to be in parallel, not in series like it would be for a voltage source. If this parallel resistance is infinite it doesn't block any output current from reach the load, rather it stops output current from leaking away before it reaches the load.



