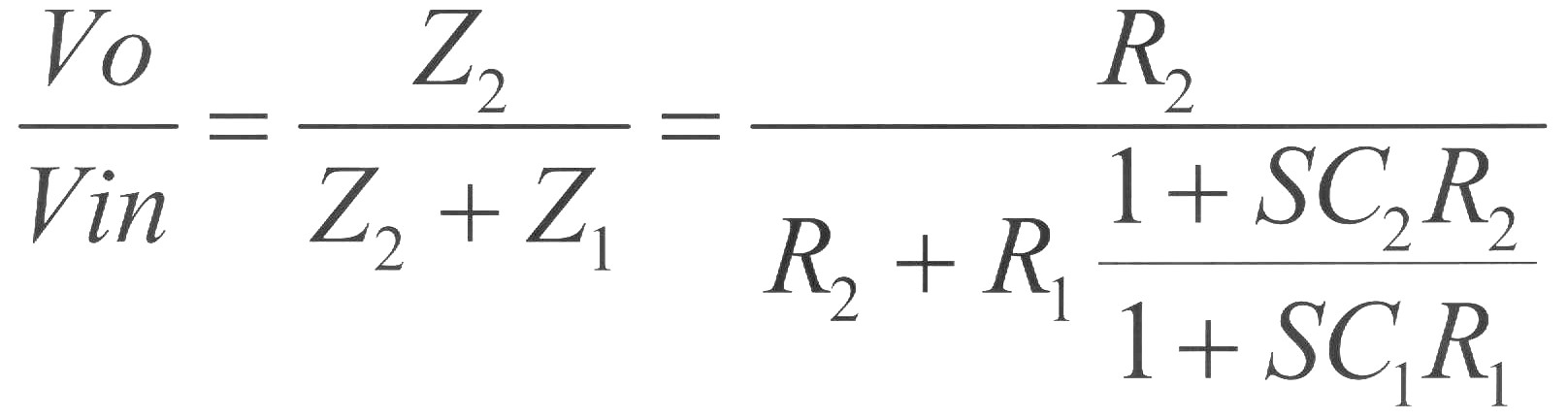Let's analyze this circuit by inspection. We have two capacitors, meaning we expect a second order transfer function. If we're lucky, no poles are complex conjugate, so we can express the transfer function as:
$$ T(s) = \frac{N(s)}{D(s)}= \alpha\frac{(1+st_L)(1+st_H)}{(1+s\tau_L)(1+s\tau_H)}$$
We can first of all derive the poles by inspection. Using a network theorem, we will derive the time constant associated with each capacitor in a superposition of effects and we will approximate the dominant pole as the sum of the two time constants.
Let's start with C2.
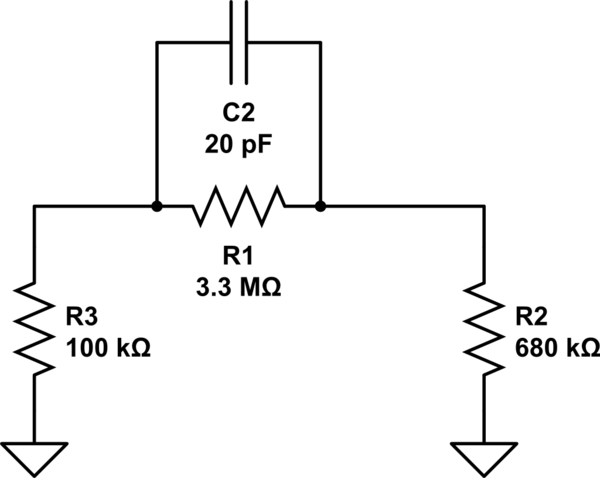
simulate this circuit – Schematic created using CircuitLab
We get that:
$$ \tau_{C_2} = C_2 (R_1 // (R_2 + R_3)) = 12.6 \mu s $$
Now we do the same for C1:
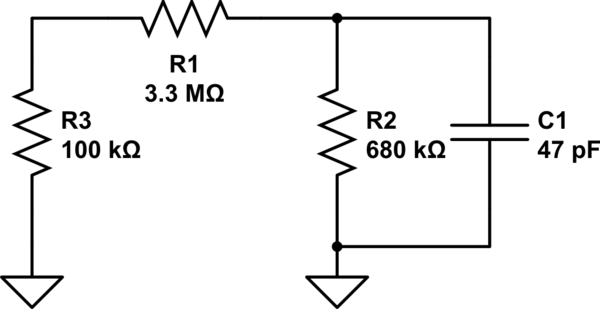
simulate this circuit
$$ \tau_{C_1} = C_1 (R_2 // (R_1 + R_3)) = 26.6 \mu s $$
The two time constants are quite close, so the approximation we're going to do won't be great but it will give us an idea.
It can be demosntrated that, if the two time constant are sufficiently far apart, the low frequency time constant of a circuit like this is:
$$ \tau_L \simeq \tau_{C_1} + \tau_{C_2} = 39.2 \mu s \Rightarrow f_L = \frac{1}{2\pi \tau_L} = 4.08 kHz $$
It also holds that the reciprocal of the higher time constant is the sum of the reciprocals of the time constants calculated with the other capacitors shorted:
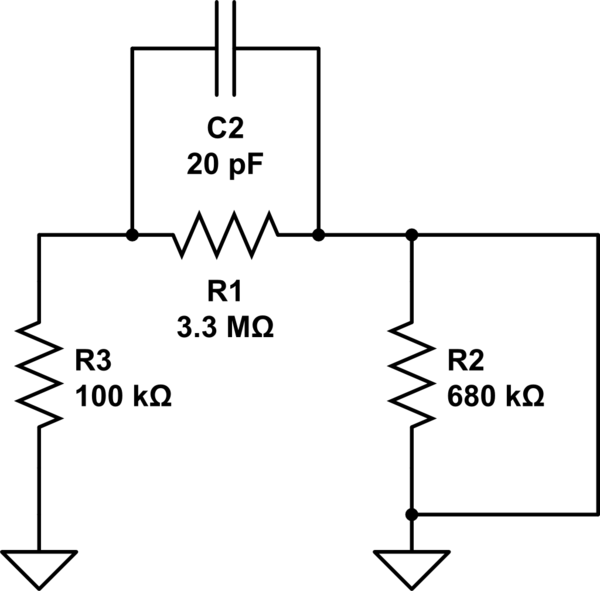
simulate this circuit
$$ \tau_{C_2}^\infty = C_2 (R_1 // R_3) = 2\mu s $$
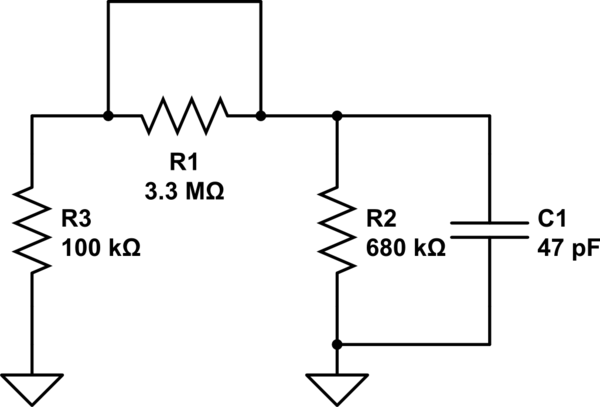
simulate this circuit
$$ \tau_{C_1}^\infty = C_1 (R_3 // R_2) = 1.7\mu s $$
So
$$ \tau_H \simeq \frac{1}{\frac{1}{\tau_{C_1}^\infty }+\frac{1}{\tau_{C_2}^\infty }} = 0.918\mu s \Rightarrow f_H = \frac{1}{2\pi \tau_H} = 173 kHz $$
Now we have both our poles, let's look for the zeros. We have a zero when, at a specific frequency, we don't have any signal at all at the output. Every capacitor introduces a zero in the transfer function and we can study them with the superposition of effects. In fact, C1 introduces a zero (allows no signal at the output) when it's a short. This means that you'll need an infinite frequency to get the zero from C1. So C1 introduces a zero at infinite frequency. Let's analyze C2. At which frequency does C2 block our signal from getting to the output? If $ C2//R1 = \infty $ we get no signal at the output.
$$ \frac{1}{sC_2} // R_1 = \infty = \frac{R_1}{1+sC_2R_1} \Rightarrow 1+sR_1C_2 = 0$$
This happens for:
$$ s = j\omega = -\frac{1}{R_1C_2} \Rightarrow f_z = \frac{1}{2\pi R_1C_2} = 2.4 kHz $$
Lastly, we have to consider our DC gain to calculate alpha. Basically we have a resistive divider that leads us to:
$$ \alpha = \frac{R_2}{R_1+R_2} = 0.17 = -15dB $$
In the end we did get this transfer function:
$$ T(s) = \alpha\frac{(1+st_L)}{(1+s\tau_L)(1+s\tau_H)}$$
So, in the audio band you will start at -15dB, then you will meet your zero at about 2.4 kHz, so your transfer function will rise at 20dB/decade until you meet your pole at about 4 kHz, then it would flatten towards infinity. Since the singularities are really close, your real transfer function will be a lot smoother than this.
Check here the bode plot of the approximation
Hope this helps.