Why must the voltage drop over resistors in parallel be the same?

Question

I am wondering why it is the case the when resistors are connected in parallel the voltage drop over them must be the same?

The reason I have seen arguing for this is that the electrons before the resistors appears in the same place, and the electrons after the resistors appears at the same place. And that means that the electrons that goes through resistor \$R_1\$ and those going through resistor \$R_2\$ are at the state before and after.

But why does this argument hold? Voltage is energy per charge, why couldn't it be the case that even though the electrons are at the same place before and after going through the resistors, the work done on the electrons going through \$R_1\$ is higher than the work done to the electrons going through resistor \$R_2\$?

Answer 1

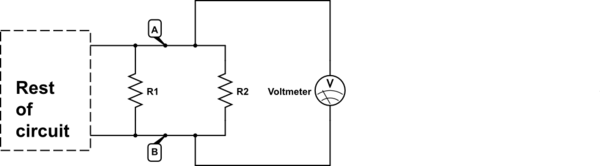
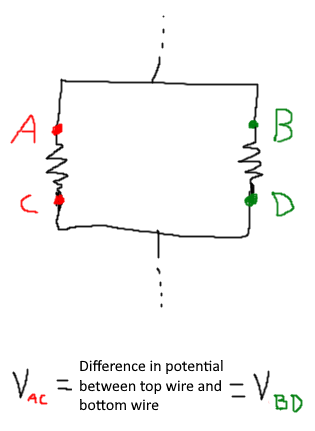
The reason I have seen argumenting for this is that the electrons before the resistors appears in the same place, and the electrons after the resistors appears at the same place. And that means that the electrons that goes through resistor \$R_1\$ and those going through resistor \$R_2\$ are at the state before and after.

Except when one is being precise, that is a fairly good explanation.

But why does this argument hold? Voltage is energy per charge, why couldn't it be the case that even though the electrons are at the same place before and after going through the resistors, the work done on the electrons going through \$R_1\$ is higher than the electrons going through resistor \$R_2\$?

[It sometimes is the case that the work done is different depending upon path. In particular, when there is a time-varying magnetic field involved. We will get to that. But first:]

Faraday's Law states:

$$\nabla \times E = -\frac{1}{c}\frac{\partial B}{\partial t}$$

If there is no time-varying magnetic field, this implies that

$$\nabla \times E = 0$$

which means that E is a conservative field. Being a conservative field implies that E is the gradient of a potential function, (which we call V)

$$E = \nabla V$$

V, being a potential function implies that the work done to move an electron from one point to another does not depend upon the path taken. That is why the voltage across two resistors in parallel are said to be equal. And, this is true whenever there is no time-varying magnetic field present.

[However, if there is a time varying magnetic field affecting the circuit, one needs to model this as if there were a transformer, or voltage source, present in one or both of the paths through the resistors. The voltages induced by these transformers will affect the voltage "dropped" by each of the resistors.]

Answer 2

I think you're over-thinking this.

Here's how the voltage across two resistors in parallel is measured:

^{simulate this circuit – Schematic created using CircuitLab}

Since R1 and R2 are in parallel they are each connected between the same nets, net A and net B. That means both R1 and R2 experience the same voltage, that is the voltage between those nets. The voltmeter also experiences that voltage and displays it as well.

Your misunderstanding could result from misunderstanding the relations between voltage, current and resistance.

I prefer to compare voltage to "pressure", in the case of parallel resistors, the voltage (pressure) on both is the same.

That voltage (pressure) will make charge / electrons flow through a resistor, compare it to how a liquid or a gas flows through a pipe. In electronics we call that flow of electrons (or charge) current and that current is directly a measure of how many electrons flow through a conductor or a resistor.

If the same voltage is applied to two resistors because they're in parallel (see above) and one resistor has a high value while the other resistor has a low value, then the low value resistor makes it "easy" for the electrons to flow through it so more electrons flow (per second) so the current will be higher (for the low value resistor). For the high value resistor it takes more effort so less current flows.

Answer 3

Here's a more elementary explanation:

In an ideal wire with 0 resistance, the electric potential is the same everywhere in the wire. In other words, the voltage between two parts of that wire is 0. This is a direct consequence of Ampere's Law (or alternatively, Kirchoff's Voltage Law).

This is true of both ends of the resistors. Thus, since the two ends of the resistors are the same two wires, both ends have the same difference in potential, aka. the same voltage.