4
\$\begingroup\$

I've been going through the design of a few different EMI filters, mainly for buck converter inputs.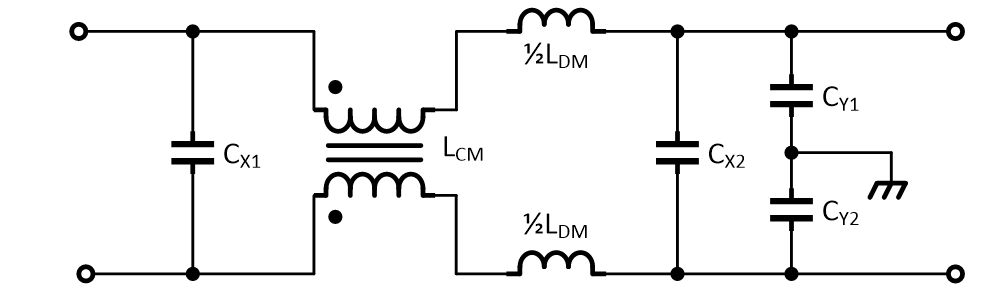Standard EMI filter for both CM and differential noise

In many cases the leakage inductance of the CM choke can provide enough inductance for the differential part of the filter. Although it's not shown in this picture, it's also extremely common to damp the differential filter via a resistor and damping capacitor (5-10 the capacitance of Cx2) in parallel with Cx2. This is natural given the peaking in the bode plot of a LC second order filter.

What I have not seen anywhere online, is a design that dampens the common mode filter with a similar network to the chassis. Why is peaking not a concern for the common mode portion of the filter like it is for the differential? Maybe the shallow impedance slope of a CM choke is a hint?

Usually the CM chokes are in the milli henries, and they have decent attentuation at >1Mhz, Cy1/2 fall around 1uF.

I'm trying to see if I can save space by picking a small CM choke in the micro-henries, and then having a series inductor, so that I can reduce Cdamp. This makes Cy1/2 have much larger capacitance values, and I'm not sure how this will interplay.

\$\endgroup\$
6
  • \$\begingroup\$ Please ask a specific question \$\endgroup\$
    – Voltage Spike ♦
    Commented Mar 21, 2021 at 4:47
  • \$\begingroup\$ Why do common-mode EMI filters lack damping networks? \$\endgroup\$
    – ardarn
    Commented Mar 21, 2021 at 6:50
  • \$\begingroup\$ Please show a simulation example of what you think might be an issue in terms of resonance/peaking and I'll try and show you how to analyse it properly and more realistically. Use in your sim a real model of a CM choke. \$\endgroup\$
    – Andy aka
    Commented Mar 21, 2021 at 10:08
  • \$\begingroup\$ Damping involves energy loss, which you usually want to minimise in a power supply. \$\endgroup\$
    – user16324
    Commented Mar 21, 2021 at 14:14
  • \$\begingroup\$ CM chokes do not see the high-current differential pulses absorbed by the downstream converter, only the differential part (the leakage inductance in case of CM choke) does hence damping for that part only. You can check an APEC seminar I taught on the subject in 2017. \$\endgroup\$
    – Verbal Kint
    Commented Mar 21, 2021 at 21:03

1 Answer 1

Reset to default
4
\$\begingroup\$

You're headed for a regulatory compliance issue

The problem with jacking up Cy is that doing so increases the leakage current from the mains to your appliance's earth grounding lead. Given that the least restrictive condition IEC(UL) 60950 permits for garden-variety devices is 3.5mA of earth leakage, this limits Cy to just under 20nF for a reasonable worst case of 240V, 60Hz power. (If your appliance is stationary and either is hardwired to the mains, plugged in via an IEC 60309 or equivalent industrial-type plug, or has a separate earthing terminal, then you can use the relaxed 5%-of-supply-current limit provisions in IEC 60950, but those aren't intended to be readily applicable to general-purpose equipment.)

\$\endgroup\$
5
  • \$\begingroup\$ Ah, that makes a lot of sense. Would you be concerned in a offline battery application? And regardless of the size of Cy, why commit the damping network to chassis? \$\endgroup\$
    – ardarn
    Commented Mar 21, 2021 at 17:12
  • \$\begingroup\$ @ardarn -- the damping network itself is irrelevant here -- the leakage is 1/2*pifC leakage through Cy, not through any other part, and exists in all mains filters that use Y caps to mains earth \$\endgroup\$
    – ThreePhaseEel
    Commented Mar 21, 2021 at 17:34
  • \$\begingroup\$ @ardarn -- can you clarify what you mean by "offline battery application"? \$\endgroup\$
    – ThreePhaseEel
    Commented Mar 21, 2021 at 17:35
  • \$\begingroup\$ Devices that aren't connected to mains or earth, but have a metal chassis. I understand that the damping network is irrelevant when talking about the leakage aspect of the system, but still, why should we not damp the common mode filter to avoid ringing during a common-mode noise event. \$\endgroup\$
    – ardarn
    Commented Mar 21, 2021 at 19:05
  • \$\begingroup\$ Just following up here @ThreePhaseEel \$\endgroup\$
    – ardarn
    Commented Mar 26, 2022 at 3:17

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.