Suppose we have this circuit:
simulate this circuit – Schematic created using CircuitLab
Z = 4-3j
I = V/Z = 1<0/5<36 = 0.2< -36 A
But when I use Ohm's law on the capacitor i get this which doesn't make any sense:
Vc = IZc = 0.2< -36 -3j = 0.2< -36 *3< -90 = 0.6< -126.
Any help appreciated
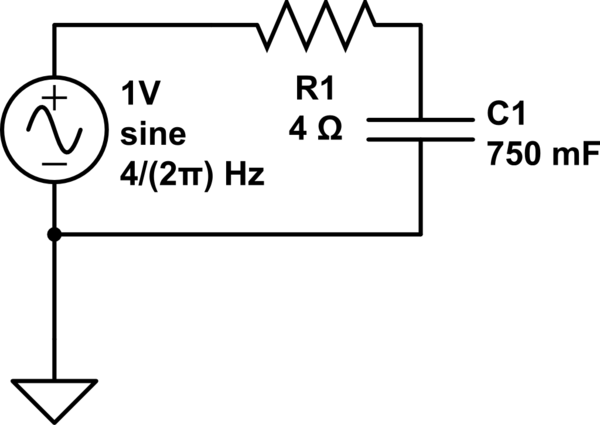
Well, we know that:
$$\underline{\text{V}}_{\space\text{C}}=\frac{1}{\text{j}\omega\text{C}}\cdot\frac{\hat{\text{u}}\exp\left(\theta\text{j}\right)}{\text{R}_1+\frac{1}{\text{j}\omega\text{C}}}\tag1$$
Assuming that the \$1\space\text{V}\$ is the amplitude of the voltage-source, we get:
$$\underline{\text{V}}_{\space\text{C}}=\frac{1}{\text{j}\cdot4\cdot750\cdot10^{-3}}\cdot\frac{1\exp\left(0\text{j}\right)}{1+\frac{1}{\text{j}\cdot4\cdot750\cdot10^{-3}}}\tag2$$
So, the function in the time domain will be:
$$\text{V}_\text{C}\left(t\right)=\left|\underline{\text{V}}_{\space\text{C}}\right|\sin\left(\omega t+\arg\left(\underline{\text{V}}_{\space\text{C}}\right)\right)\tag3$$
And you will find:
$$\left|\underline{\text{V}}_{\space\text{C}}\right|=\frac{1}{\sqrt{10}}\approx0.316228\space\text{V}\space\space\space\wedge\space\space\space\arg\left(\underline{\text{V}}_{\space\text{C}}\right)=-\arctan(3)\approx-1.24905\space\text{rad}\tag4$$
By l = V/Z we get the magnitude and phase angle of the total current in the circuit.
By V(c) = I * Z(c) we get the magnitude and phase angle of the voltage across the capacitor.
By your calculations current in the network leads the voltage across the capacitor by 90 degree.
Completely make sense.
