6 dB in-line 50 ohm attenuator

Question

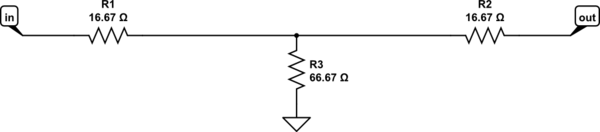
Recently someone posted a question regarding an inline device to attenuate their voltage on a 50 \$\Omega\$ coax by half (i.e. 6 dB). Because the question asked for commercial recommendations, it was closed. However, the question of how to create an attenuator that has the same input and output impedance is a reasonable one, I was not able to find a similar question, although my search was perhaps superficial. If this is a duplicate, and provides no new value, I will gladly delete it.

The easy part of the answer is a circuit that gives 6 dB attenuation with both input and output impedances matching 50 ohms. I will give that answer shortly. A harder part, is how to physically construct such a circuit such that the transition between coaxial cable and circuit and back to coax is not afflicted by reflections due to changing impedance. This difficulty is perhaps part of the reason why commercial in-line attenuators tend to be expensive. Any advice in this area will be a welcome addition.

Answer 1

The following simple circuit provides "6" dB attenuation and 50\$\Omega\$ input impedance, assuming that the load at the output is also 50\$\Omega\$. (To be precise, it is an attenuator with a attenuation factor of 0.5 or 6.021 dB)

^{simulate this circuit – Schematic created using CircuitLab}

The circuit can easily be adjusted for 75\$\Omega\$ lines simply by scaling each resistor by 75/50 = 1.5

Edit:

AndyAka has kindly given an equation which contrains the resistor values for a T-pad attenuator with equal input and output impedances:

$$R_{in} = R_{out} = R_1\sqrt{1 + \frac{2 \cdot R_3}{R_1}}$$

(I have modified it to match the schematic in my answer. In this equation \$R_1\$ is equal to \$R_2\$).

Answer 2

Of course you could just use a calculator specifically designed for that application: Everything RF T-attenuator