Current Division Question

Question

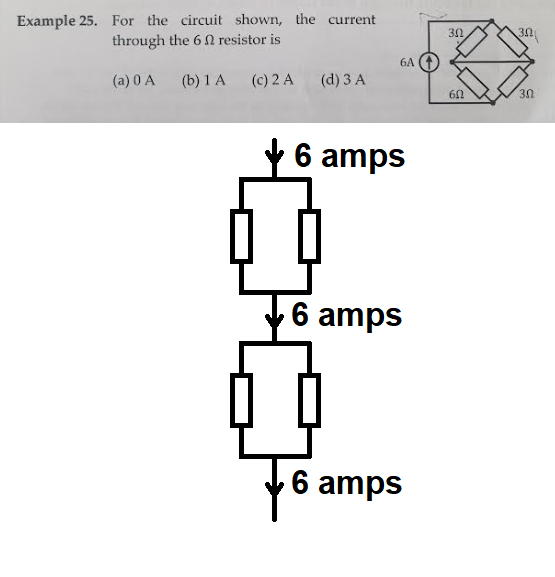
I am trying to solve this current division question and I wanted to check if my work was correct.

I've tried applying equivalent resistance for the two parallel circuits to form 1 series circuit with (1.5 ohms) and (2 ohms) equivalent resistors. This allowed me to find the Voltage at equivalent resistor (2ohms) at 6A*2 = 12V.

As I = V/R and voltage is constant in a parallel circuit 12/6 = 2 A for the 6 ohm resistor. This however, doesn't really utilise the current division rule but the KVL rule instead. How should I approach this question instead?

Answer 1

Forget you ever heard that KVL and KCL were useful for solving problems like this. They're just ways to state the obvious. KVL says the sum of voltage changes around a loop is zero. Of course, it is. otherwise, the voltage at a node could have two or more values. KCL says the sum of the currents entering a node is zero. Again, of course. Otherwise, there would be charge building up at the node. The current division rule, the KVL and the KCL rules are just consequences of Ohm's law. You don't have to memorize anything more.

You were right in saying the 3//3 ohms is 1.5 ohms, and the 3//6 ohms is 2 ohms, but the two 3 ohm resisters can have any value. If one of them was a milliohm and the other a megohm, it wouldn't change the answer. There's 6 amps going through the 6//3 ohms parallel combination. The voltage across them would be 12 volts, but whatever it is, the current through the 6 ohm resistor will be 1/3 the current through the 3 ohm resistor, per ohm's law: 2 amps in this case.

Answer 2

Just think about what I've drawn below your picture: -

I'm not explicitly telling you how to look at this; I'm giving a hint: Is the current through the lower left hand resistor affected by either of the two resistors above it?

Here's another hint; when you see a circuit that might confuse you; redraw it.