I have been trying to find the quality factor of this RLC circuit for some time now. My method is trying to find the equivalent series RLC circuit and calculate the resonant circuit. When I work through the algebra and plug the values in I get values in the order of 10^9, so clearly my working is wrong.
It is an unfamiliar combination as the inductor and capacitor are kind of series and parallel at the same time and it is very confusing.
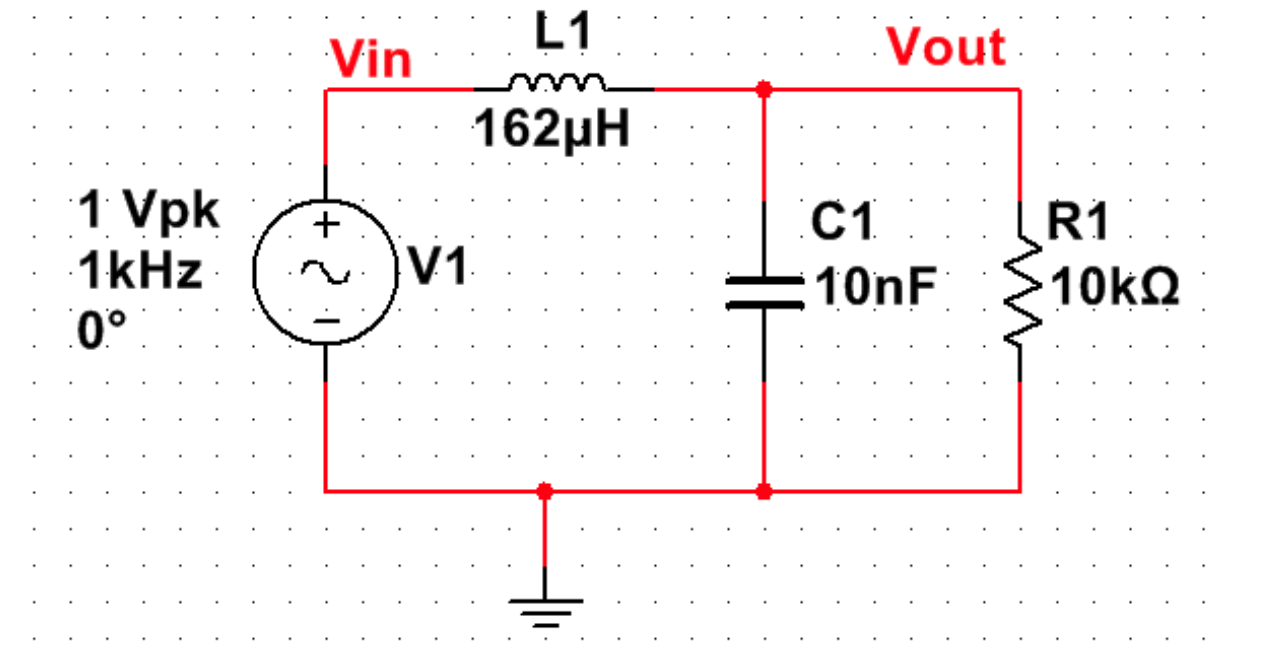
Well, the transfer function of the circuit is given by:
$$\mathscr{H}\left(\text{s}\right):=\frac{\text{V}_\text{o}\left(\text{s}\right)}{\text{V}_\text{i}\left(\text{s}\right)}=\frac{\text{R}\space\text{||}\space\frac{1}{\text{sC}}}{\left(\text{R}\space\text{||}\space\frac{1}{\text{sC}}\right)+\text{sL}}=\frac{\text{R}}{\text{CLRs}^2+\text{Ls}+\text{R}}\tag1$$
Where \$\alpha\space\text{||}\space\beta:=\frac{\alpha\beta}{\alpha+\beta}\$.
Now, when working with sinusoidal signals we can use \$\text{s}:=\text{j}\omega\$ (where \$\text{j}^2=-1\$ and \$\omega=2\pi\text{f}\$ with \$\text{f}\$ is the frequency of the input signal in Hertz). So, we get:
$$\underline{\mathscr{H}}\left(\text{j}\omega\right)=\frac{\text{R}}{\text{CLR}\left(\text{j}\omega\right)^2+\text{L}\text{j}\omega+\text{R}}=\frac{\text{R}}{\text{R}\left(1-\text{CL}\omega^2\right)+\text{L}\omega\text{j}}\tag2$$
So, the absolute value if given by:
$$\left|\underline{\mathscr{H}}\left(\text{j}\omega\right)\right|=\frac{\text{R}}{\sqrt{\left(\text{R}\left(1-\text{CL}\omega^2\right)\right)^2+\left(\text{L}\omega\right)^2}}\tag3$$
The quality factor is given by:
$$\mathcal{Q}:=\frac{\hat{\omega}}{\mathcal{B}}\tag4$$
Where \$\hat{\omega}\$ is the frequency (in rad/sec) where \$\left|\underline{\mathscr{H}}\left(\text{j}\omega\right)\right|\$ is at the maximum and \$\mathcal{B}\$ is the bandwidth and is given by \$\mathcal{B}=\left|\omega_--\omega_+\right|\$ with \$\$ and \$\$ are the upper and lower cut-off frequencies (in rad/sec).
Now, we can solve for the unkowns using your values, we get:
$$\frac{\partial\left|\underline{\mathscr{H}}\left(\text{j}\omega\right)\right|}{\partial\omega}=0\space\Longrightarrow\space\omega:=\hat{\omega}=\frac{5000 \sqrt{1999838}}{9}\tag5$$
And:
$$\left|\underline{\mathscr{H}}\left(\text{j}\omega\right)\right|=\left|\underline{\mathscr{H}}\left(\text{j}\hat{\omega}\right)\right|\cdot\frac{1}{\sqrt{2}}\space\Longrightarrow\space\omega=\frac{5000}{9} \sqrt{2 \left(999919\pm9 \sqrt{1999919}\right)}\tag6$$
So, the quality factor is:
$$\mathcal{Q}=\frac{1}{9} \sqrt{\frac{999919 \left(\sqrt{999676013122}+999919\right)}{3999838}}\approx78.5611\tag7$$
