1
\$\begingroup\$

In texts I see the example for RLC series circuits which is more straightforward, but how can I set the equations for the following circuit to find its response either in time or s domain and reach the response for the inductor current?

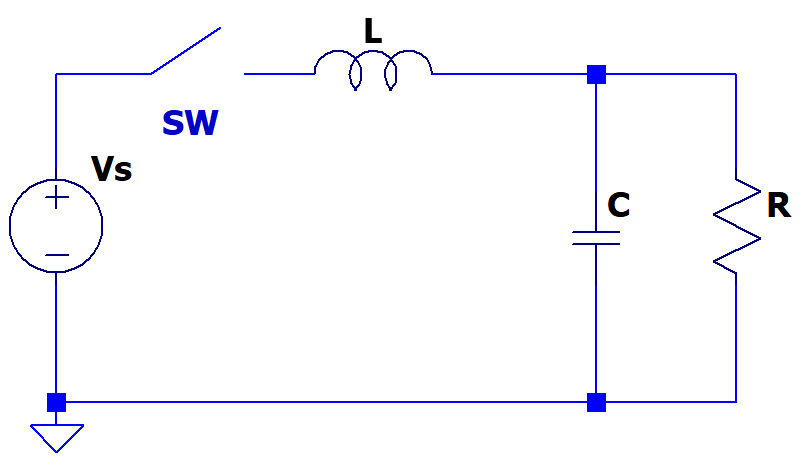
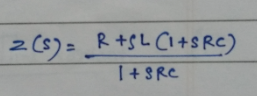enter image description here

If I apply KVL there is two KVL. Should KCL be used here? I'm confused about how to approach this.

\$\endgroup\$
4
  • \$\begingroup\$ The method of solving for this circuit is up to you and whatever you believe is the easiest way to find the solution. You could KVL or KCL or Laplace Domain. Though in my opinion, the Laplace Domain is the easiest way to solve for something, especially when there are reactive elements in the circuit. \$\endgroup\$
    – user103380
    Commented Feb 19, 2018 at 2:41
  • \$\begingroup\$ This will be second order system response but how to set the circuit equation here to begin with? \$\endgroup\$
    – user1999
    Commented Feb 19, 2018 at 2:44
  • \$\begingroup\$ Since this is a second order circuit, you need to check if this circuit is overdamped, underdamped, or critically damped. From there you need to find your eigenvalues and your roots. After that, you can find your general \$i(t)\$ equation. Whether or not \$i_L(t)\$ is equal to \$i(t)\$ is up to find out ;) \$\endgroup\$
    – user103380
    Commented Feb 19, 2018 at 3:10
  • \$\begingroup\$ You will only get one KVL equation, not sure how you are getting two. \$V_s=I(sL+\frac{\frac{1}{sC}×R}{\frac{1}{sC}+R})\$ \$\endgroup\$
    – Harry Svensson
    Commented Feb 19, 2018 at 5:49

1 Answer 1

Reset to default
1
\$\begingroup\$

The best way would be to do it in the Laplace domain and then convert it back to the time domain.

Clearly the current supplied by the source is equal to the inductor current. Find the equivalent impedance and divide it by the supply voltage to get the current equation.

The equivalent impedance comes out to be - 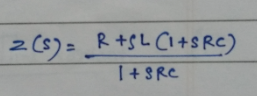enter image description here

The current equation then comes out to be - enter image description here

Now you can take the inverse laplace of this to find the time domain expression.

Method 2 would be, as it has been pointed out in the earlier comments, is to find out the damping ratio and determine the nature of the system, but that would of course require the component values. I have provided you a general method to solve it.

\$\endgroup\$
6
  • \$\begingroup\$ Great answer. But how could you get that denominator with rho? \$\endgroup\$
    – user1999
    Commented Feb 19, 2018 at 10:08
  • \$\begingroup\$ I apologize for that. It's an 's', the laplace variable. Actually, I'm still not accustomed with writing formulas or equations through text so I had to write it down in my notebook. \$\endgroup\$
    – Rohan Singh
    Commented Feb 19, 2018 at 10:27
  • \$\begingroup\$ I see. But if I want to find the damping for the current from this equation, should I convert the equation for I(s) to a specific format? Like nominator is a constant and the denominator is s^2+ax+b ect? I dont know how to achieve this algebrically. \$\endgroup\$
    – user1999
    Commented Feb 19, 2018 at 10:32
  • \$\begingroup\$ If there are non-zero initial conditions, the Laplace transform is not always the best method. \$\endgroup\$
    – Chu
    Commented Feb 19, 2018 at 14:51
  • \$\begingroup\$ @newage2000 - You don't need to consider the numerator, just look for the quadratic term in the denominator and bring it to the form of s^2+as+b. You can easily determine the natural frequency and the damping factor using a and b. Natural frequency is the square root of b and a=2(damping factor)(natural frequency). \$\endgroup\$
    – Rohan Singh
    Commented Feb 19, 2018 at 15:33

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.