Problem in the book "Radio Antennas and Propagation"

Question

I came across a problem in an electronics textbook by William Gosling, Radio Antennas and Propagation.

I am an undergraduate and I sent this to my lecturer to help solve but unfortunately, her answer was incorrect. She came up with a maximum range of 283 000 km whilst the book gives a grossly redacted solution of simply 8000 km. I understood her approach to the problem but then she converted quanta into Joules in a way I did not understand.

I have attempted it myself to no avail.

Edit: My lecturer's attempt

My attempt using matlab:

Answer 1

Interesting question! I took a different approach that gave me the same answer as the book, so I think I'm on the right track.

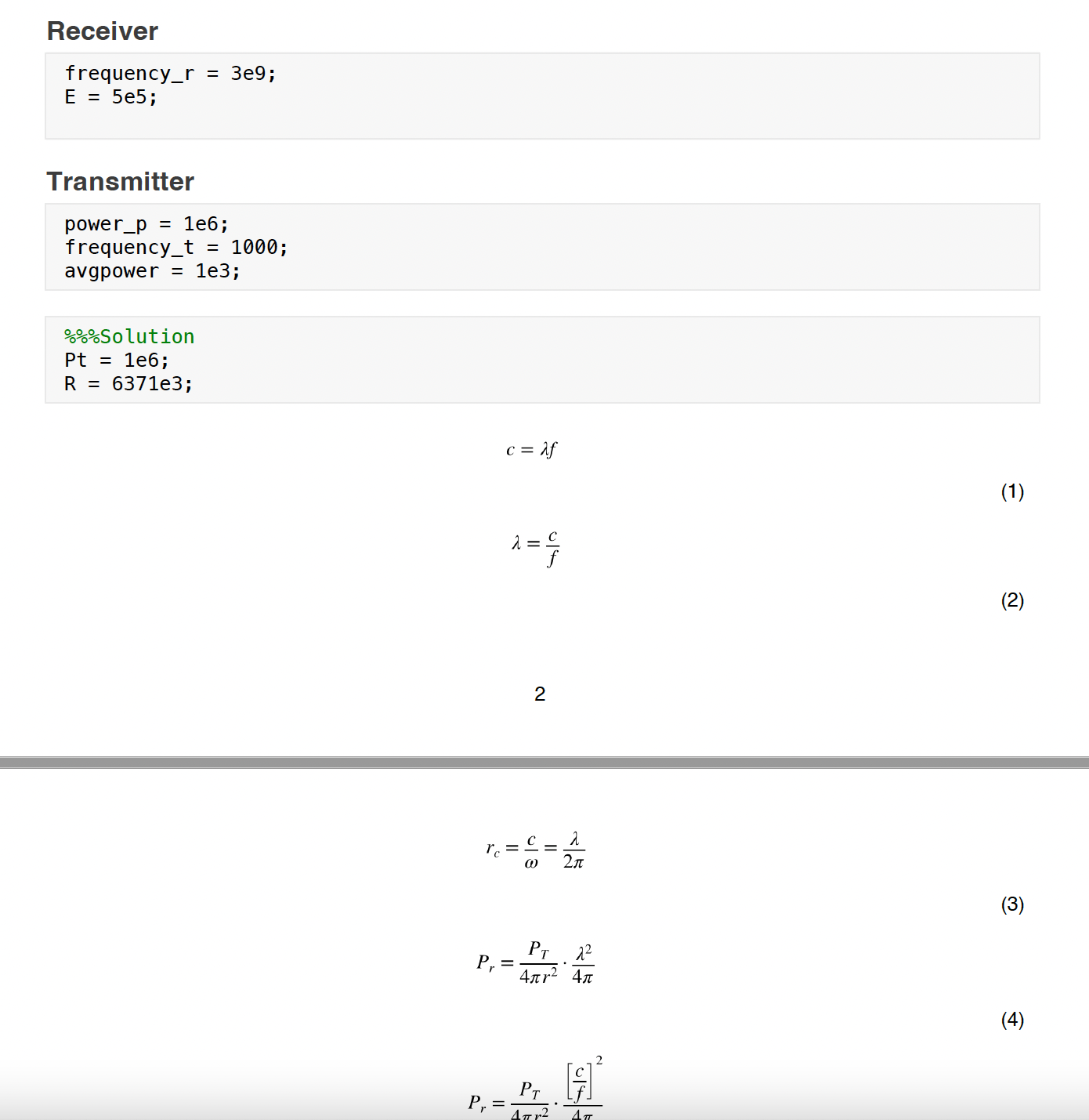
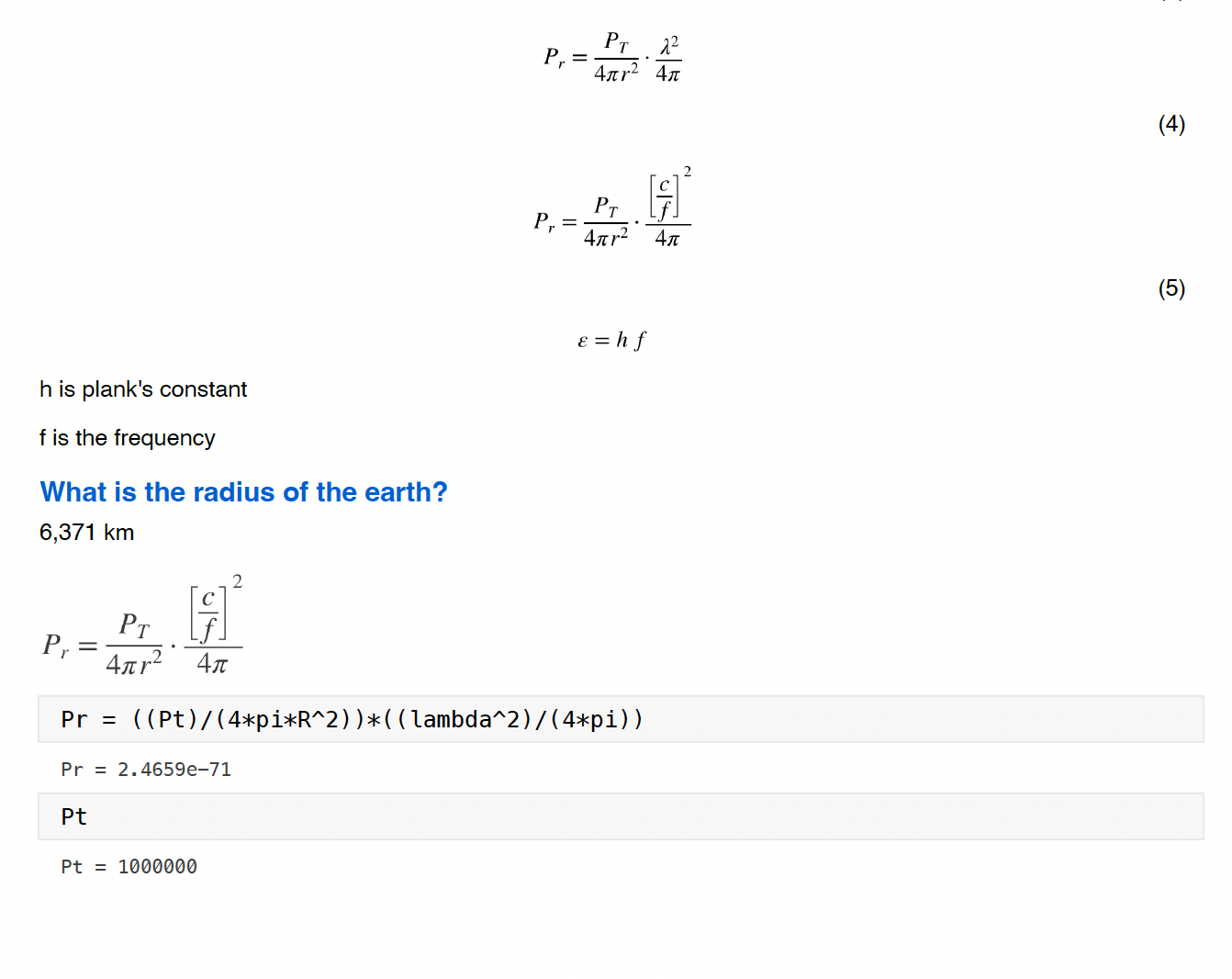
The receiver sensitivity is given in terms of energy, which works out to

$$5\cdot 10^5 \cdot h \cdot f = 5\cdot10^5\cdot 6.626\cdot 10^{-34} \text{ J/Hz}\cdot 3\cdot 10^9\text{ Hz} \approx 1\cdot 10^{-18}\text{ J}$$

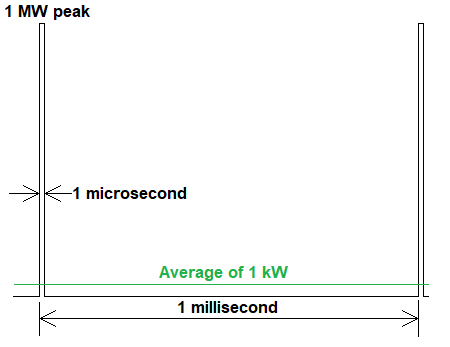
The transmitter outputs 1 J per pulse, so how much of this energy does the receiver intercept? That requires knowing the effective aperture of an isotropic antenna, which is basically \$\lambda^2/4\pi\$. At 3 GHz, that works out to (0.1 m)² / 4π.

The transmitter's energy is distributed evenly over a sphere that has a surface area of 4πr², so the surface energy density is

$$\frac{1\text{ J}}{4\pi r^2}$$

The receiver is going to intercept that density multiplied by its aperture, or

$$\frac{(0.1\text{ m})^2}{4\pi}\cdot\frac{1\text{ J}}{4\pi r^2}$$

We just need to find the value of r for which this equals 10^-18 J. Rearranging terms, we get

$$r^2 \leq \frac{(0.1\text{ m})^2}{(4\pi)^2 10^{-18}}$$

Take the square root of both sides:

$$r \leq \frac{0.1\text{ m}}{4\pi\cdot 10^{-9}}$$

Which works out to approximately 8×10⁶ meters, or 8000 km.

Answer 2

Convert quanta to energy, multiply by number of quanta then convert to power

This is based on \$E = hf\$ where \$f\$ = 3 GHz and \$h\$ is Planck's constant (\$6.6261 × 10^{−34}\$ J*s).

• The energy per quanta is therefore \$1.99 × 10^{-24}\$ joules.

• With \$5 × 10^{+5}\$ quanta that's a total energy of near-enough \$10 × 10^{-19}\$ joules

• For each 1 microsecond pulse\$^1\$, that's a receive power of \$10 × 10^{-13}\$ watts or -120 dBW.

Find the link/path loss

Given that the originating pulse power is 1 MW, that's a link/path loss of 180 dB.

The Friis transmission equation for isotropic antennas in decibels

$$\color{red}{\boxed{\text{Path loss (dB) = 32.45 + } 20log_{10}(f) + 20log_{10}(d)}}$$

Where \$f\$ is in MHz and \$d\$ is in kilometres.

• This means that \$180 = 32.45 + 69.54 + 20 log_{10}(\text{distance})\$

• Therefore, \$78 = 20 log_{10}(\text{distance})\$

Hence, I get distance to be 7,943 km (corrected from earlier).

---

---

\$^1\$ The answer has been edited to fix a brain-fart

• I wrongly calculated the pulse width to be 1 millisecond.
• It's actually 1 μs and this makes all the difference.

A thousand pulses per second means a 1 ms period and, the peak:average being 1000:1 means a 1 μs pulse width: -

I guess it does agree with the book (despite the spelling mistakes).

Answer 3

Let's do orders of magnitude to check whose answer is closer to right:

• A photon has energy \$E=hf\$, \$h\$ being Planck's constant. \$h\approx 7·10^{-34}\,\text{J/Hz}\implies h·3\!·\!10^9\,\text{Hz}\approx 2.1\!·\!10^{-24}\,\text{J}\$.
• Each TX pulse has 1J (don't know what we'd need the peak power for? It's 1000 pulses a second, each second we emit 1 kJ, so one pulse has 1 J).
• So, the loss is 1 / (2·10⁻²⁴) = 50·10²²
• Free space path loss is \$\left(\frac{4\pi r f}{c}\right)^2\$, with \$r\$ being the distance between transmitter and receiver.

Solving for \$r\$:

\begin{align} \left(\frac{4\pi r f}{c}\right)^2 &= 50·10^{22}\\ \frac{4\pi r f}{c} &\approx 7·10^{11}\\ 4\pi r f &\approx c· 7·10^{11}\\ r &\approx \frac{c· 7·10^{11}}{4\pi f}\\ &= \frac{3·7·10^{19}}{4·3·3·10^{9}}\text{m}\\ &= \frac{7·10^{10}}{12}\text{m}\\ &\approx 5·10^6\,\text{km} \end{align}