How to model the input and output process flow in a liver model to simulate it?

Question

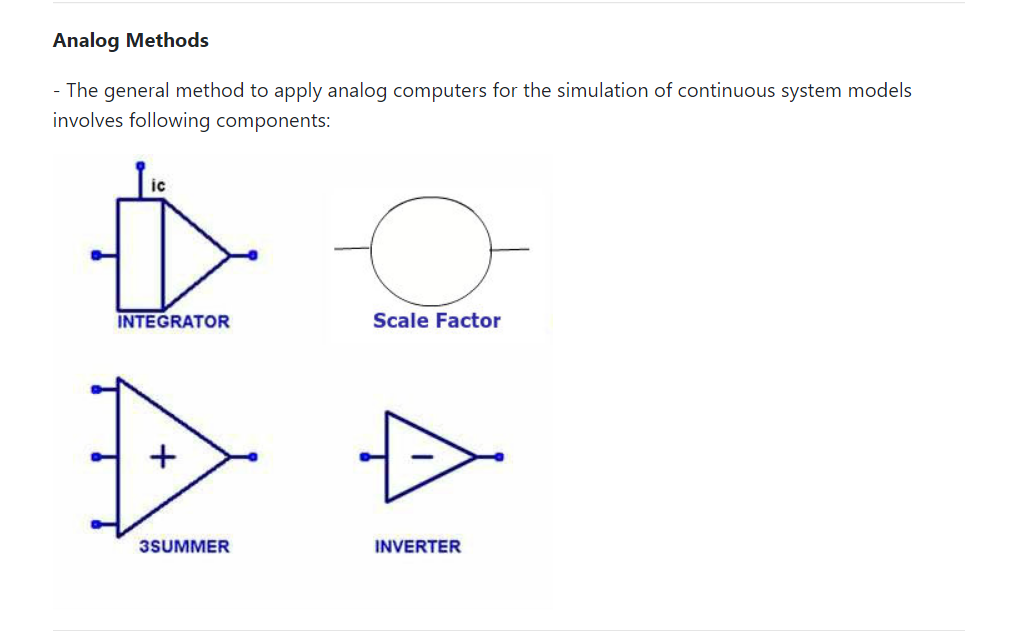
What's the confusion:

Confusion is to how to model the equations. What signs to use for incoming and outgoing components in a process flow. The confusion isn't mainly for how to translate the equations to a amplifier-figure(although I learnt a lot from the previous answer), it's mainly how to convert that first block diagram I've mentioned to equations. And it's only the second equations that I'm confused of signs positive or negative.

My thoughts about sign what they should be:

If the arrows are coming towards the block in consideration, add + sign else add - sign.

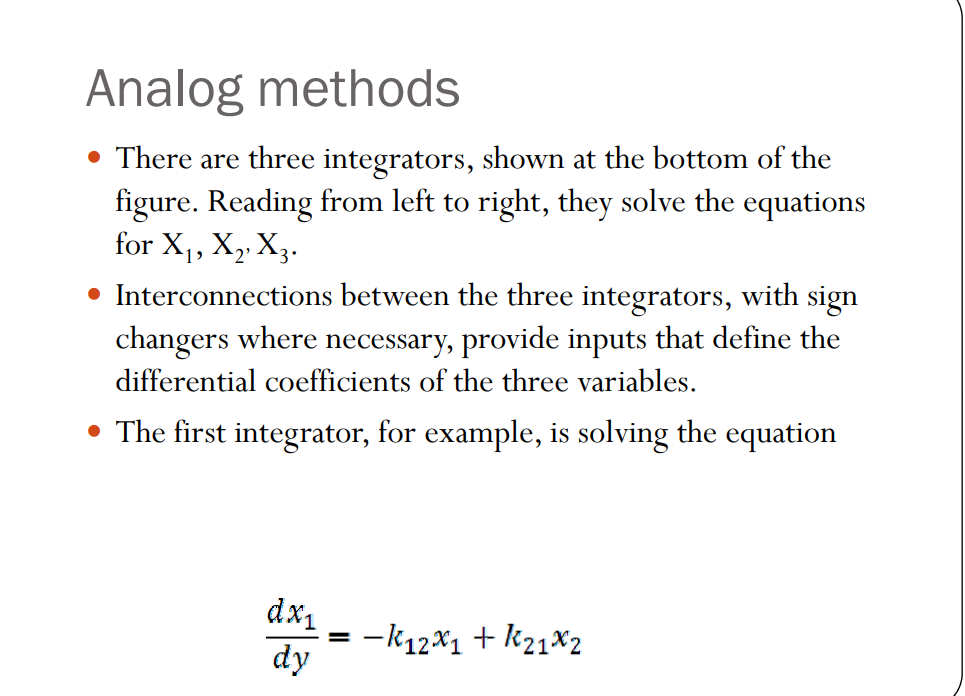
For example: consider c1 (c1 in the book is labeled x1 in the slide) block, then we find x1', for that **+ will be what comes towards it i.e $$K_{21}*x_2$$, and - will be what goes outside i.e. $$K_{12}*x_1$$.

I really don't know why, because it's probably related to medical sciences. But I don't need to understand it as far as I know.

So, $$ x_1'=K_{21}*x_2-K_{12}*x_1$$

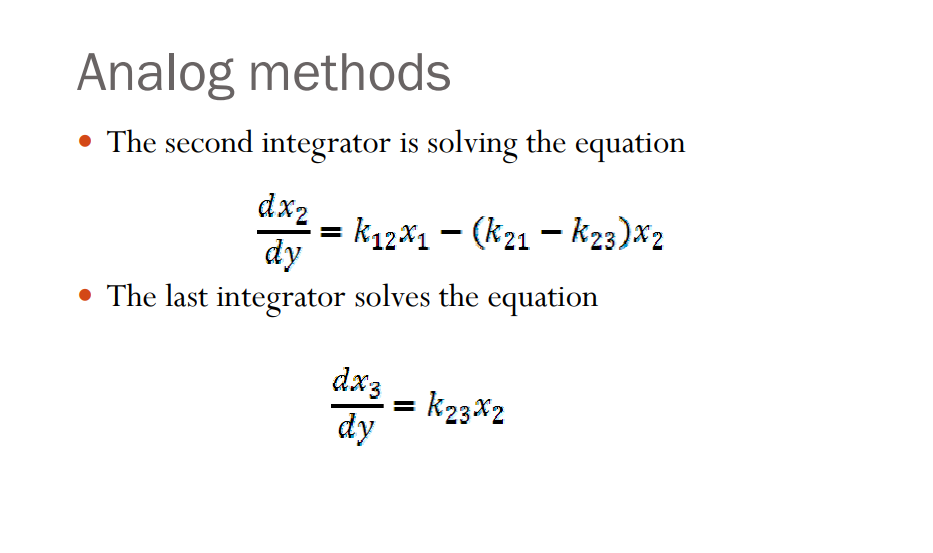
So according to this logic, for x2', this'd be the equation-:

$$x_2'=K_{12}*x_1-K_{21}*x_2-K_{23} *x_2$$

This is the notation being used.

How to translate compartmentalized liver to analog computer? I just want to learn to translate the figure to equations.

This is the question:

International source:

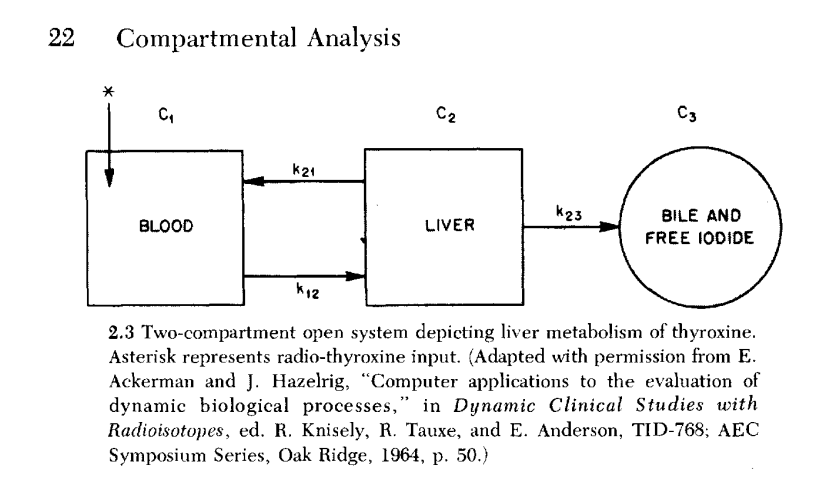
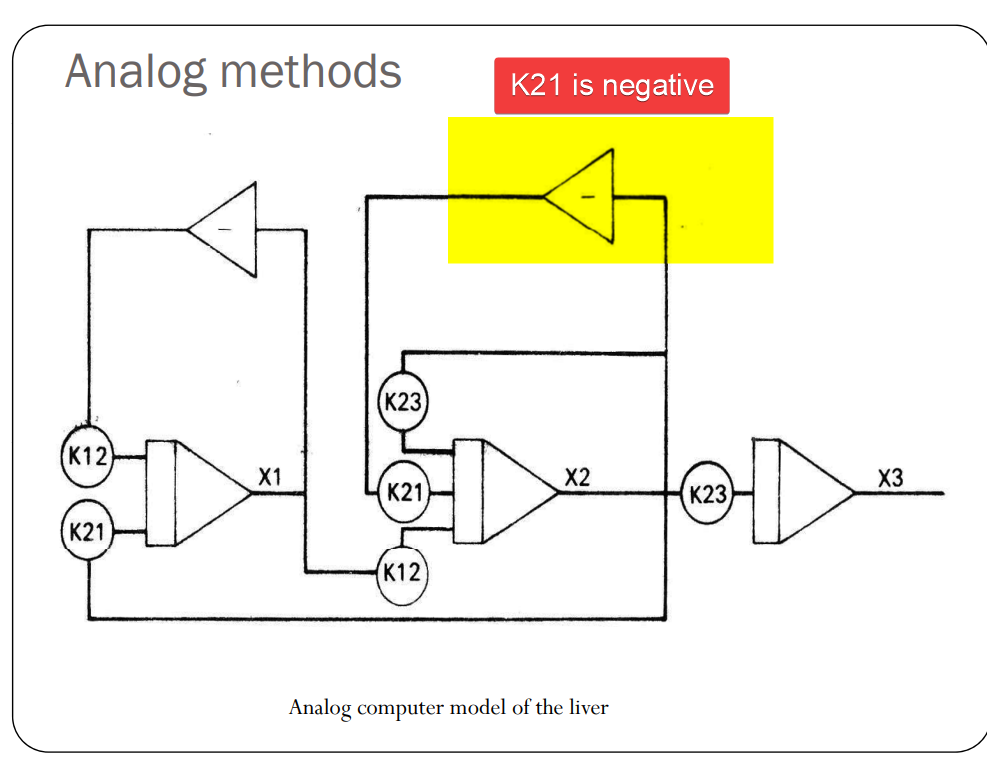
The compartmental analysis figure is the diagram that I want to translate to differential equations and eventually analog computer.

This is from mathematical models in the health sciences book by Eugene Ackerman.

I don’t know about the author, but I bet that this is extremely reliable compared to the materials locally available and written by local authors. But it doesn't tell which equation it is modeling, so I am not 100 % sure about it. But to be honest, I don't clearly understand its conventions and it's age old book which is un-updated, so relying on it would be a mistake.

It has problems as well:

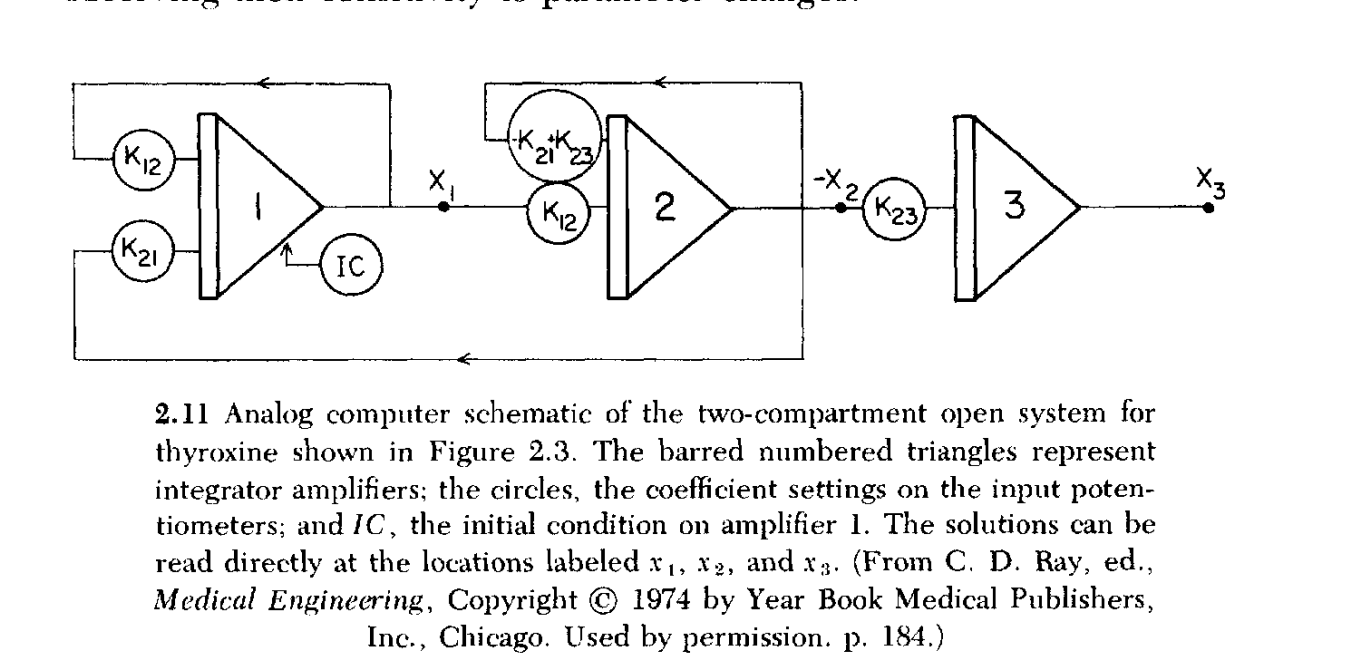
It doesn't use negative for $$K_{12}$$ And idk why is it using negative for $$K_{23}$$.

Less reputed source:

This is pretty ambiguous, their equation and figure don't even match.

If $K_{21}$ is negative then $$K_{23}$$ should also be negative(although it doesn't writes that in its equation.

I can guess that it's trying to subtract the net output instead of doing it individually, but I'm not sure why it's doing it.

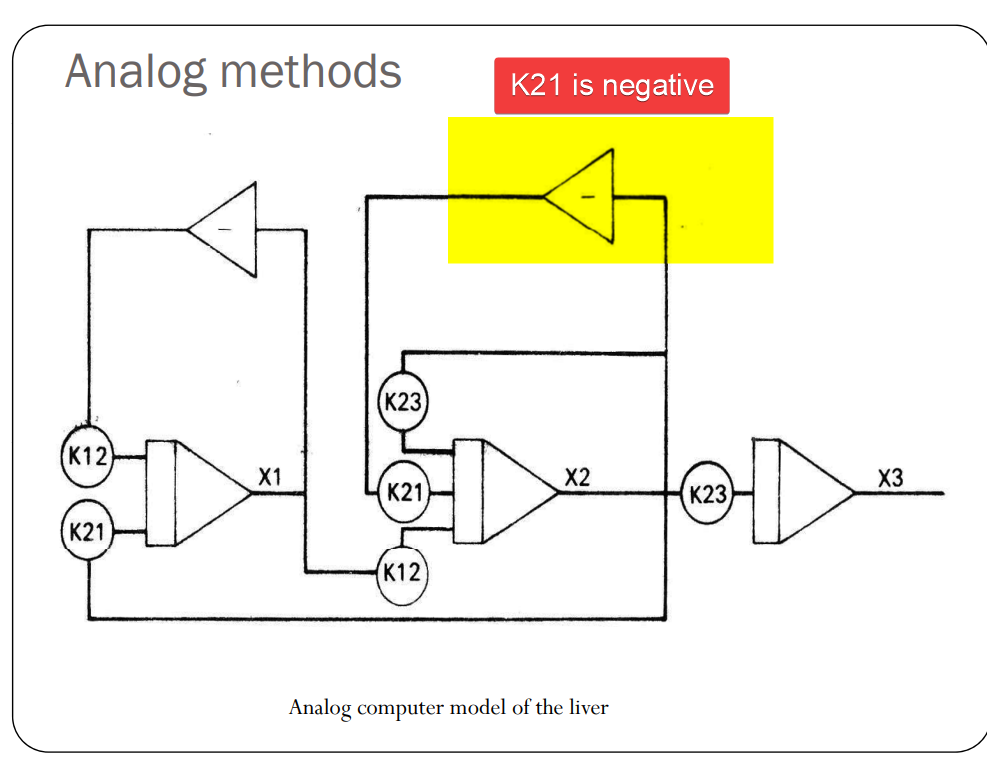
Highly reputed source (LOCAL):

If this source is correct, I don't understand how is it correct?

My thoughts about sign what they should be:

If the arrows are coming towards the block in consideration, add + sign else add - sign.

For example: consider c1 (c1 in the book is labeled x1 in the slide) block, then we find x1', for that **+ will be what comes towards it i.e $$K_{21}*x_2$$, and - will be what goes outside i.e. $$K_{12}*x_1$$.

I really don't know why, because it's probably related to medical sciences. But I don't need to understand it as far as I know.

So, $$ x_1'=K_{21}*x_2-K_{12}*x_1$$

So according to this logic, for x2', this'd be the equation:

$$x_2'=K_{12}*x_1-K_{21}*x_2-K_{23} *x_2$$

My teacher (he is not the one to check the final papers):

He says "some books have written plus in $$K_{21}$$ while some minus I am not sure what it is actually."

We don't know who will check the paper or who checked it.

Answer 1

You really want to read an analog computer introductory material from that era. There is a lot of unspoken convention in those diagrams. For example, all summers (triangles) are assumed to be inverting, and same goes for integrator inputs (triangles with a vertical bar). That's because the implementations used inverting amplifiers, to reduce the number of stages needed. E.g. an inverting integrator can be done with just one high-gain inverting stage.

To understand analog computation diagrams - which are their own technical language and are not circuit diagrams! - see e.g. A Practical Approach to Analog Computers by Strong and Hannauer. You won't go very far without trying some simple examples using e.g. SPICE or on a breadboard.

The translation between the differential equations and integrators is also a specific process tailored to the building blocks available in an analog computer.

For numerical solutions, there's no need to translate differential equations into equivalent analog circuits. I.e. if you can look up a reference on liver physiology that's tailored towards bio-modelling, you'll get the differential equations, and then you can either solve them directly using Octave, Mathematica, etc., or use a reference on analog computing to translate them into an analog computation diagram.

It doesn't use negative for K12. And idk why is it using negative for K23.

Potentiometer coefficients were usually positive-only, between 0 and 1. Inversions, if needed, were done using summer blocks. A triangle without a bar is a summer, and if the gains are not shown, they are -1 by default. Summers typically had inputs with gains of -1 and -10. The negative sign was not written out explicitly! This is the language that publications that used analog computer models would use, the language that the analog computer application notes used, the language that anyone doing anything with analog computing had to learn, since it mapped almost 1:1 to the configuration of the hardware the problem would run on. That's how you'd read that publication from the 70s. The later stuff is, sadly, too often regurgitated without the wherewithal to spend an hour or two read the free contemporaneous introductory materials.

So, a summer element with a "-" sign in the middle is a creation of someone who doesn't know what they are talking about, pretty much :(

Analog computation diagrams do not have "positive" and "inverting" summers, nor are there separate inverters. The "summer" and "inverter" symbols from the diagram you cited are bogus:

Do Not Use These Symbols Like This!

You've also shown this part of the diagram:

K21 is not negative, because K21 is a pot with a value between 0 and 1. All inputs to the integrator are inverting by convention.

The liver model, redrawn with implied conventions made explicit, is:

The integral equations that it models are:

$$ \begin{aligned} X_1(t) &= \int{-K_{12}X_1(t) - K_{21}(-X_2(t)) {\,\rm d}t} + {I\!C}_1, \\ -X_2(t) &= \int{-(K_{23}-K_{21})(-X_2(t)) -K_{12}X_1(t) {\,\rm d}t}, \\ X_3(t) &= \int{-K_{23}(-X_2(t)) {\,\rm d}t}. \\ \end{aligned} $$

Simplifying a bit:

$$ \begin{aligned} X_1(t) &= \int{-K_{12}X_1(t) + K_{21}X_2(t) {\,\rm d}t} + {I\!C}_1, \\ X_2(t) &= \int{K_{12}X_1(t) + (K_{21}-K_{23})X_2(t) {\,\rm d}t}, \\ X_3(t) &= \int{K_{23}X_2(t) {\,\rm d}t}. \\ \end{aligned} $$