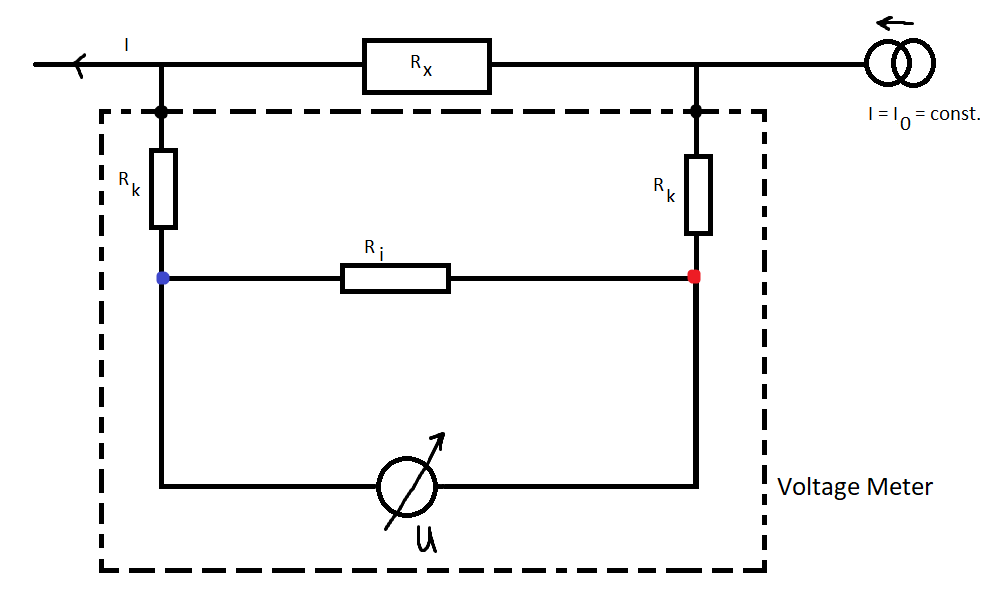
There is a question of terminology here. You say you are tasked with finding the voltage measured by the 'voltage meter'. In the drawing there is a current source, a resistance \$R_x\$ and a box labeled 'Voltage Meter' with resistors \$R_i\$ and \$R_k\$ and a meter movement labeled '\$\mu\$' or 'u'.
If we take this to mean that the voltage meter is made up of the movement and resistors in the box, the voltage that it will measure is the voltage across \$R_x\$.
This voltage will be \$I\$ times the total resistance, \$R_x\$ in parallel with the voltage meter resistance.
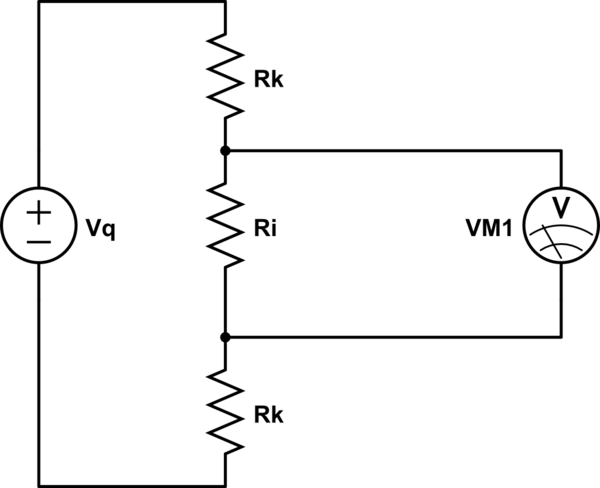
The problem is how to determine the voltage meter resistance. If we take the \$R_k\$ values as the connection resistance (and assume they are equal) and \$R_i\$ as the meter internal resistance it would be $$2R_k + R_i$$
If the meter movement has resistance that is not included in \$R_i\$ then that has to be taken into account and it becomes $$2R_k + (R_i || R_{movement})$$
Since no value is given for the meter movement's resistance you would either have to assume that it is part of \$R_i\$, that it is large enough to not matter, or that you can't answer without knowing it. I would lean towards it being part of \$R_i\$.
If on the other hand, the 'voltage meter' you are supposed to find the measurement of is not the boxed area but the meter movement \$\mu\$, then look at the other answers here.