Why is the output voltage expression wrong?

Question

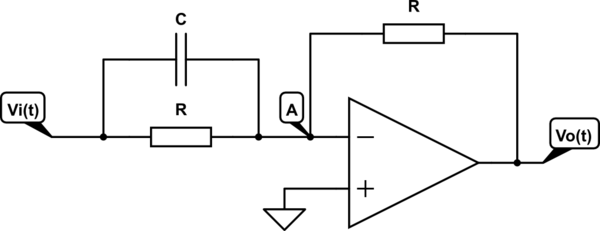
I derived the expression for the output voltage in this circuit, however, I have got the wrong answer. The problem is that I just know that it is the wrong answer because it's a multiple choice question and this was one of the wrong choices (hence I don't know why it is wrong - it seems correct to me!).

$$KCL_a:\frac{e_x-v_i}{R} + C\frac{dv_i}{dt} + \frac{e_x-v_o}{R} = 0$$ \$e_x = 0\$ because of virtual GND; so the equation becomes: $$\frac{-v_i}{R} + C\frac{dv_i}{dt} + \frac{-v_o}{R} = 0 \iff v_o(t) = -v_i + RC\frac{dv_i}{dt}$$

However it seems this is the wrong answer and I just don't understand why it is the case. Maybe it has to do with considering node a as it is? I think I can connect the wires into a single node at a because there is not element between.

^{simulate this circuit – Schematic created using CircuitLab}

Answer 1

Well, notice that no current flows into the negative terminal of the opamp. This implies that:

$$\text{I}_{\text{R}\space\text{||}\space\text{C}}=\text{I}_\text{R}\tag1$$

Using that we can see that:

$$\text{V}_\text{i}\cdot\left(\text{R}\space\text{||}\space\frac{1}{\text{sC}}\right)^{-1}=\frac{\text{V}_--\text{V}_\text{o}}{\text{R}}\tag2$$

And \$\text{V}_-=\text{V}_+=0\space\text{V}\$.

So, we get:

$$\text{V}_\text{i}\cdot\left(\frac{\text{R}\cdot\frac{1}{\text{sC}}}{\text{R}+\frac{1}{\text{sC}}}\right)^{-1}=\text{V}_\text{i}\cdot\frac{1+\text{sCR}}{\text{R}}=\frac{0-\text{V}_\text{o}}{\text{R}}\space\Longleftrightarrow\space\text{V}_\text{i}\left(1+\text{sCR}\right)=-\text{V}_\text{o}\tag3$$

Answer 2

The KCL for the A node is:

$$\frac{v_{_\text{A}}}{R}+\frac{v_{_\text{A}}}{R}+C\frac{\text{d}}{\text{d}t}v_{_\text{A}}=\frac{v_{_\text{O}}}{R}+\frac{v_{_\text{I}}}{R}+C\frac{\text{d}}{\text{d}t}v_{_\text{I}}$$

or,

$$\frac{v_{_\text{A}}-v_{_\text{O}}}{R}+\frac{v_{_\text{A}}-v_{_\text{I}}}{R}+C\frac{\text{d}}{\text{d}t}\left(v_{_\text{A}}-v_{_\text{I}}\right)=0\:\text{V}$$

or, with \$v_{_\text{A}}=0\:\text{V}\$,

$$\begin{align*} -v_{_\text{O}}-v_{_\text{I}}-R\,C\,\frac{\text{d}}{\text{d}t}v_{_\text{I}}&=0\:\text{V} \\\\ -v_{_\text{O}}&=v_{_\text{I}}+R\,C\,\frac{\text{d}}{\text{d}t}v_{_\text{I}} \end{align*}$$

You could put the above into standard form for a 1st order equation and try to solve it in the time domain. Or you could cast it into Laplace notation and solve for the transfer function:

$$\begin{align*} -V_{_\text{O}}&=V_{_\text{I}}+R\,C\,sV_{_\text{I}} \\\\ -V_{_\text{O}}&=V_{_\text{I}}\left(1+R\,C\,s\right) \\\\ \frac{V_{_\text{O}}}{V_{_\text{I}}}&=-1-R\,C\,s \end{align*}$$

Not sure if that helps. But there it is.