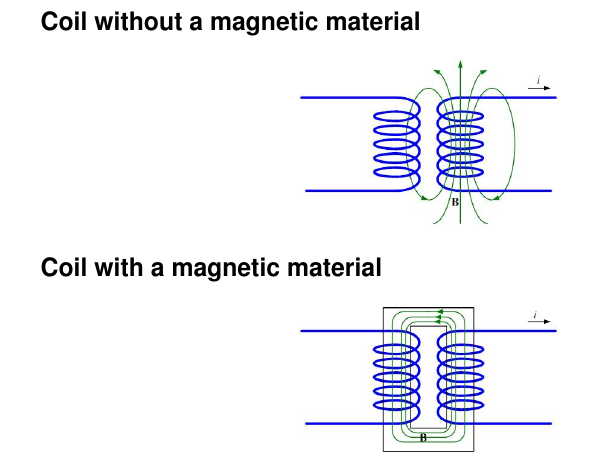
I'm slightly confused as to how the magnetic core below "confines" the magnetic field generated by the coil to flow so nicely. What stops it from generating the same pattern as in the first image?
1 Answer
It doesn't 'confine' the magnetic field as such.
Both with and without the core, the H-field created by the flow of current in the windings creates the same B-field (or magnetic flux) in the air space around them.
What the core does do is increase the B-field in the core, possibly by 1000 fold or more. This means the magnetic behaviour is dominated by the field in the core, rendering the remaining field in the air space essentially irrelevant.
-
\$\begingroup\$ Ah, I see, thanks! A follow-up question: how does the B-field so sharply "turn" the 90 degree corners of the core? With respect to magnetic domains etc.? \$\endgroup\$ Commented Oct 23, 2022 at 9:17
-
\$\begingroup\$ it really doesn't ever make a 90° turn, but to answer "why is the field shaped exactly as it is?", you'll need to learn Maxwell's equations. You're leaving the high-level view of things and turning into "how can I quantitatively describe the field", and there's no way around learning the formulas that do exactly that! (in your case with static magnetism, Ampère's circuital law and Faraday's law of induction would suffice, because there's no time-dependency involved. But with the two other equations, you can then describe everything that involves magnetism, including electromagnetic waves.) \$\endgroup\$ Commented Oct 23, 2022 at 10:06
-
\$\begingroup\$ If we look at 99.9% of the field that we pretend is 'confined' in the magnetic core, then the core is the only place it can be, so it goes round corners. If it went straight at the corner, so out of the side of the core into air, it would store a huge amount of energy in the air. Physics doesn't 'like' large energy concentrations, so it avoids it if it can, by staying in the core. Much the same as why a springy steel wire tends to be straight. To bend it requires energy, if it can unbend then it does. This is very, very hand-wavy, not Maxwell's. These things don't have wants or desires. \$\endgroup\$– Neil_UKCommented Oct 23, 2022 at 10:11
-
\$\begingroup\$ Perhaps your intuition is contaminated by, for instance, a car, which doesn't go round corners so well. That has momentum, so needs a force to turn it, to change its velocity vector. There is nothing moving along what we draw a magnetic lines, in fact there is some debate as to whether magnetic lines are a useful model at all. There is energy associated with the field, and like all physics, we can compute a force corresponding to a movement by computing dE/dx, the work per distance something is moved. \$\endgroup\$– Neil_UKCommented Oct 23, 2022 at 10:20
-
1\$\begingroup\$ @Andyaka It's a very hand-wavy answer to match the pitch of the OP's question, so I don't really care exactly what I mean. Please feel free to edit for pedantry. I thought that flux is B-field * area, so as we weren't being quantitative, didn't really find a distinction. I'm off to make and eat lunch now. I'll come back and see how you've improved it in a few hours. \$\endgroup\$– Neil_UKCommented Oct 23, 2022 at 10:58