0
\$\begingroup\$

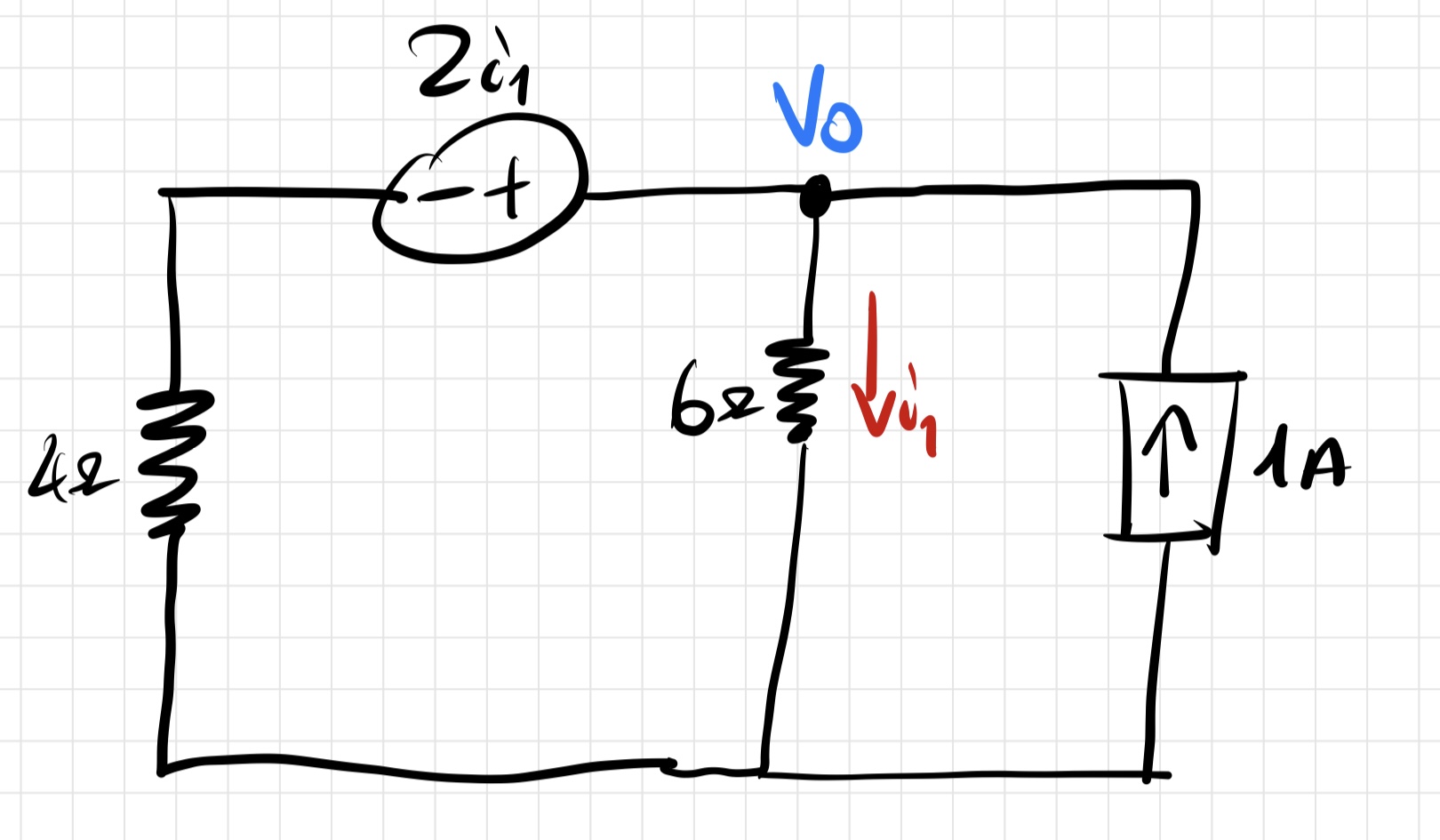
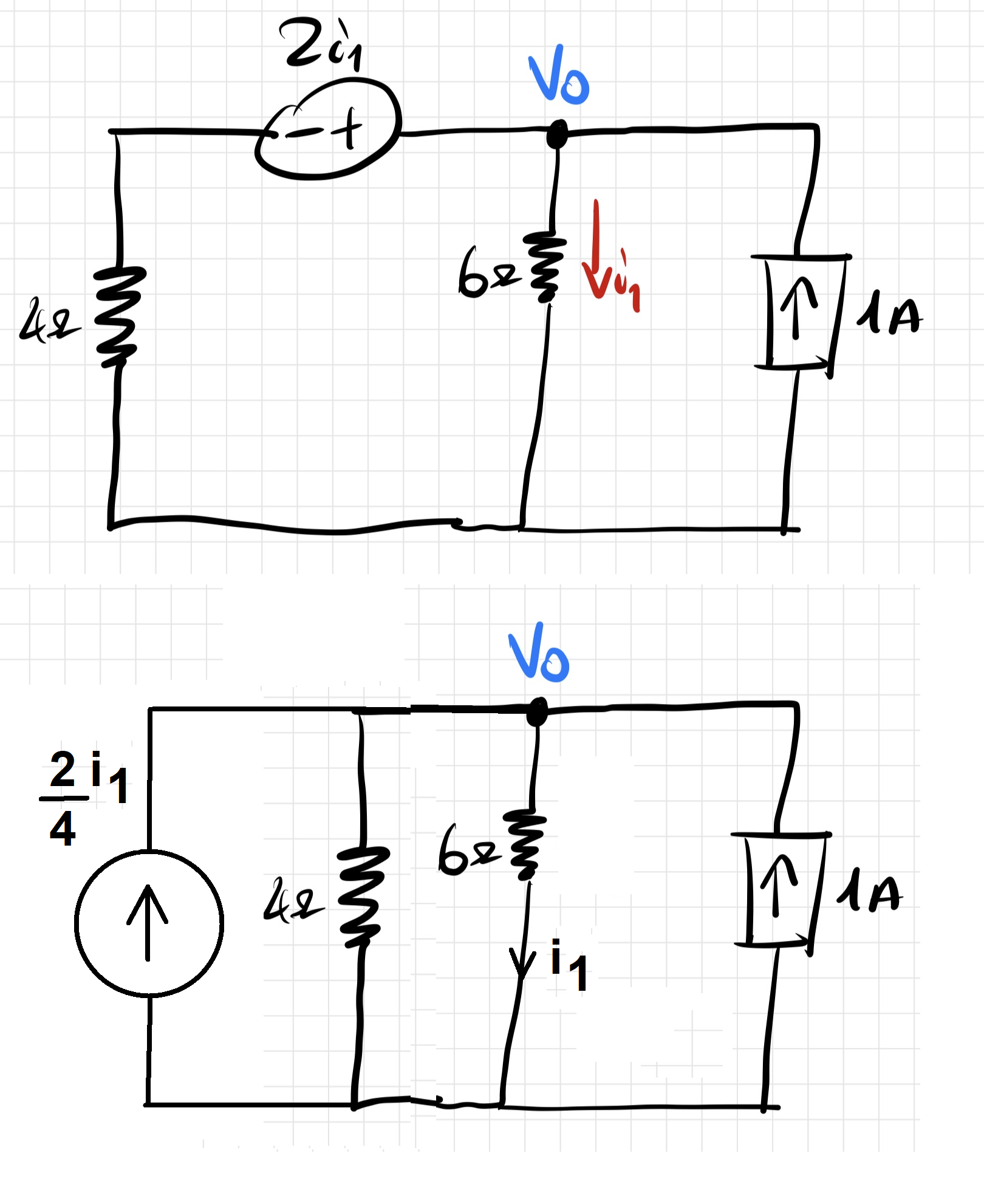
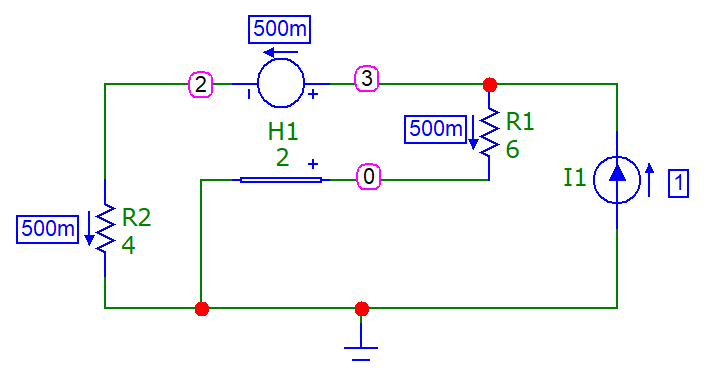
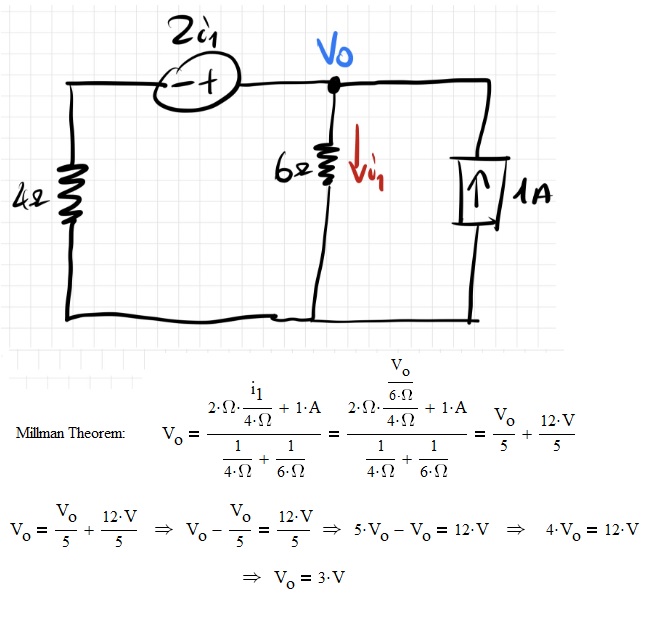
I have the following circuit as shown in the picture. My question is how can I perform Node analysis in this circuit in the node \$V_{0}\$?

I want to find \$i_{1}\$ but without KCL or KVL. Just the node analysis in the node \$V_{0}\$.

I tried : \$\frac{V_{0}}{6} +\frac{V_{0}}{4}-1 = 0 \$ ignoring the ccvs. If I take it into account the dependent ccvs how the node analysis will be?

Any help?

(Side note: I know that KCL in node \$V_{0}\$ and KVL in the left sub circuit solves it but I want to see the node analysis how will be changed if I take into account the dependent source. The current source 1A is the circuit excitation in the terminal A,B)

enter image description here

\$\endgroup\$
13
  • 1
    \$\begingroup\$ Well, if you ground the bottom node then Vo/6 is i1. So the dependent source is Vo/3. Does that make sense, so far? \$\endgroup\$
    – periblepsis
    Commented Sep 19, 2023 at 11:10
  • 1
    \$\begingroup\$ So the voltage over the 4 Ohm resistor must be Vo minus the dependent source, which is Vo/3. So that means Vo - Vo/3. But that is just 2/3*Vo. \$\endgroup\$
    – periblepsis
    Commented Sep 19, 2023 at 11:13
  • 1
    \$\begingroup\$ So the current sinking into the 4 Ohm must be [(2/3)*Vo]/4 or 1/6 Vo. And the current sinking into the 6 Ohm must be Vo/6 or i1, as already mentioned. The sum of these two is Vo/3, not so? And these two sinking currents must be equal to 1 A. If Vo/3 = 1, then what is Vo? \$\endgroup\$
    – periblepsis
    Commented Sep 19, 2023 at 11:16
  • 1
    \$\begingroup\$ Just put it all together. You've got all the pieces now. The full expression would be written: Vo/6 {the current in the 6 Ohm} + (Vo - 2*i1)/4 {the current in the 4 Ohm} = 1 A {the current sourced into the node}. Then substitute i1=Vo/6 to get: Vo/6 + (Vo - 2*Vo/6)/4 = 1 A. \$\endgroup\$
    – periblepsis
    Commented Sep 19, 2023 at 11:22
  • 1
    \$\begingroup\$ You could just as well swap positions of the dependent source and the 4 Ohm resistor, since they are both in series with each other. The equation would be written out a little bit differently. But the result would be the same. See if you can do it that way. \$\endgroup\$
    – periblepsis
    Commented Sep 19, 2023 at 11:29

2 Answers 2

Reset to default
1
\$\begingroup\$

I want to find i1 but without KCL or KVL.

I'd convert the dependent voltage source into a dependent current source like this: -

enter image description here

Then, it's clear that the current through the 4 Ω resistor is \$1 - 0.5i_1\$.

I'd multiply \$i_1\$ by 6 to get \$V_{O}\$ and, equate this to \$1 - 0.5i_1\$ multiplied by 4 (also equals \$V_O\$): -

\$6\cdot i_1 = 4(1 - 0.5i_1)\$

Do a few lines of rearranging to find that \$i_1\$ equals 0.5 amps.

Sanity check in microcap: -

enter image description here

Note that I haven't used KVL or KCL.

\$\endgroup\$
0
\$\begingroup\$

If you don't want to apply KVL or KCL, if the network is in the right configuration (as in this case) then apply Millman's theorem:

Circuit

\$\endgroup\$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.