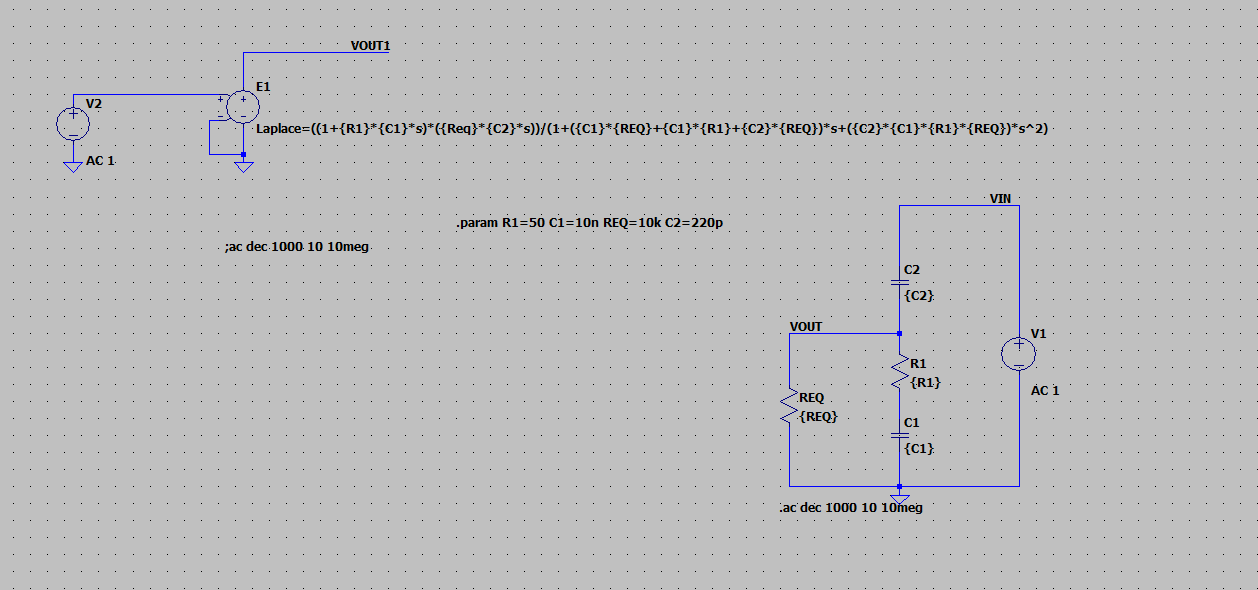
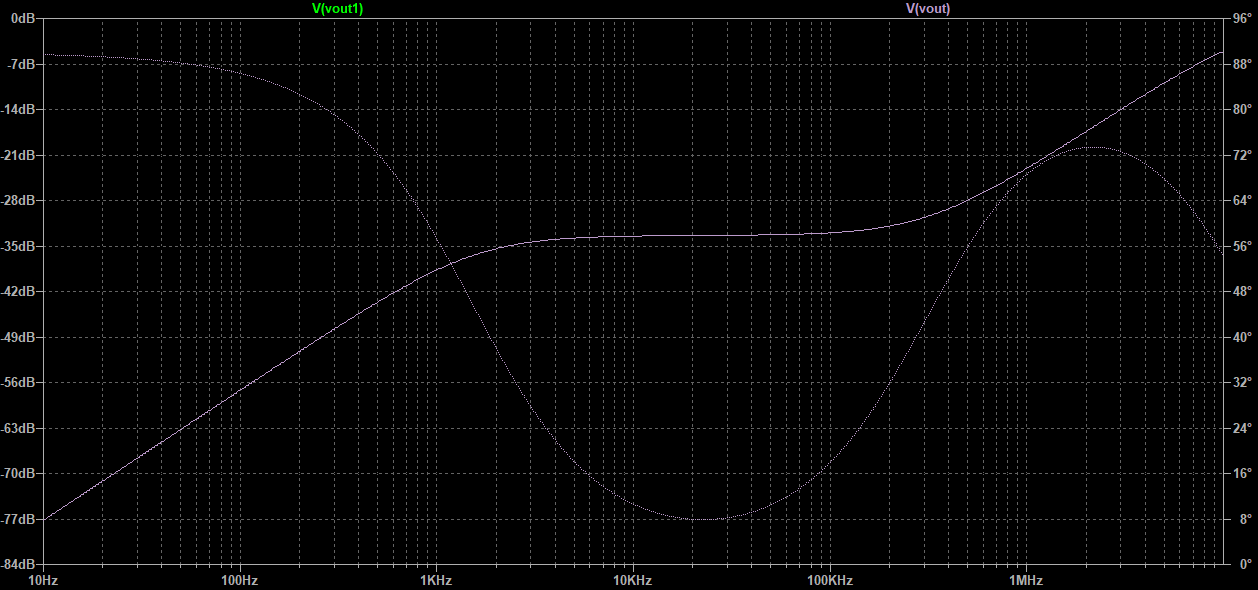
Expressing the transfer function (TF) the right way is often the key to figure out its asymptotic response. A well-organized or well-ordered expression was designated as a low-entropy result by Dr. Middlebrook in his classes on analytical techniques.
With a well-written transfer function, you should infer the position of the poles, zeroes and gains for instance, naturally figuring out the asymptotic response or the approximate Bode plot. And later on determine the components values to build the circuit. So it's truly a design-oriented analysis or D-OA, another acronym forged by Dr. Middlebrook. Your expression was probably derived using brute-force analysis and could be advantageously reorganized.
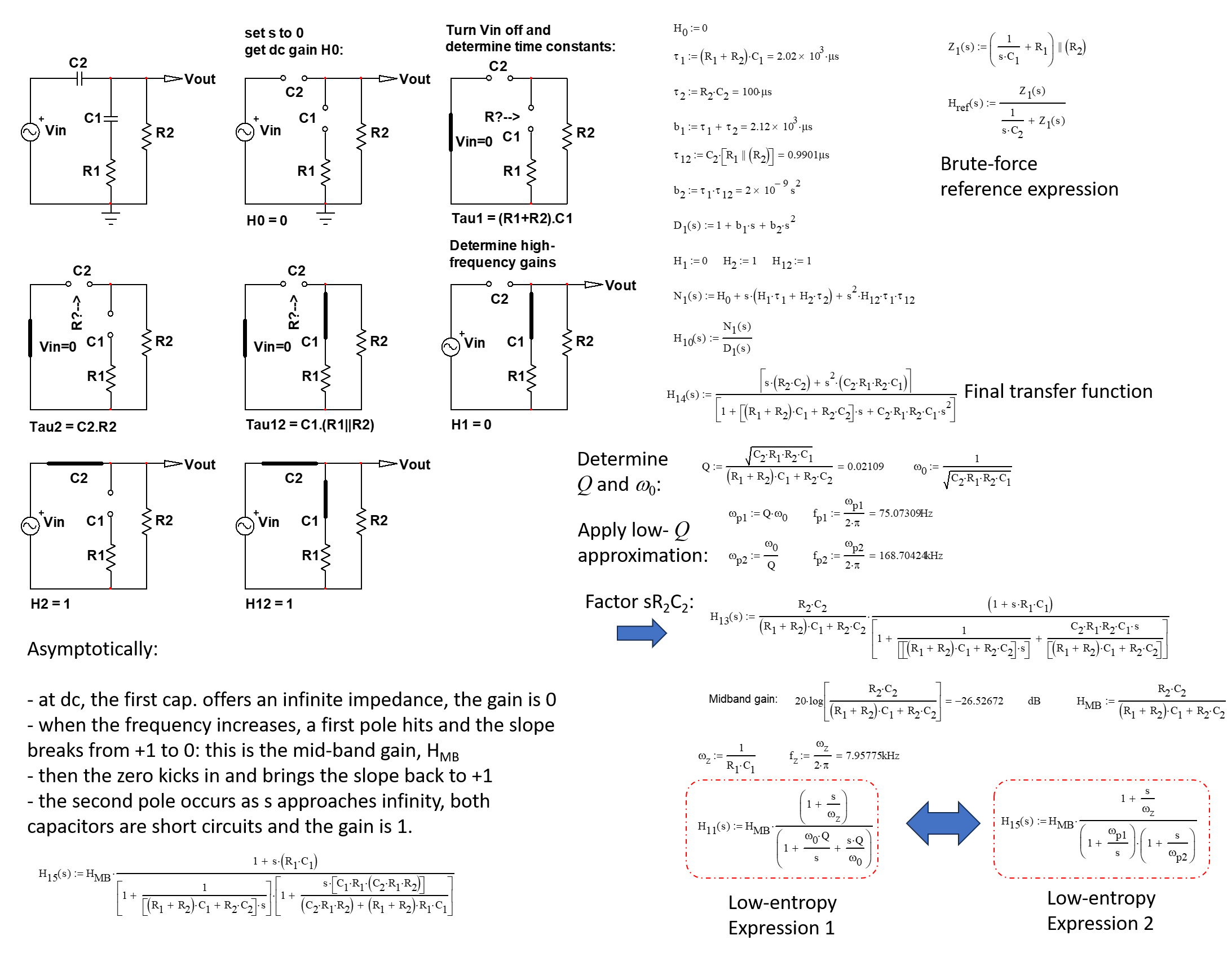
In my analysis, I have applied the fast analytical circuits techniques or FACTs that I described in the last book that I have released on this topic. The idea is simple: determine the gain \$H_0\$ of the circuit at dc for \$s=0\$ and then, turn the excitation off to determine the time constants of the circuit. You do it by temporarily disconnecting one of the energy-storing elements - you only have capacitors in this 2nd-order circuit - and you "look" through the connecting terminals to determine the resistance \$R\$ you see while the ac source \$V_{in}\$ is replaced by a short circuit. Then, you find the various \$\tau=RC\$ to form the denominator. The numerator can be obtained using a null double injection (NDI) or, in a simpler manner, by determining high-frequency gains, later combined with the time constants you already have. What is cool is that, very often, you can inspect the circuit without writing a line of algebra. See the flow below:
With an expression now featuring a mid-band gain, a zero and two poles, you can construct the asymptotic response of this transfer function. The plots in the right side confirm the approach:
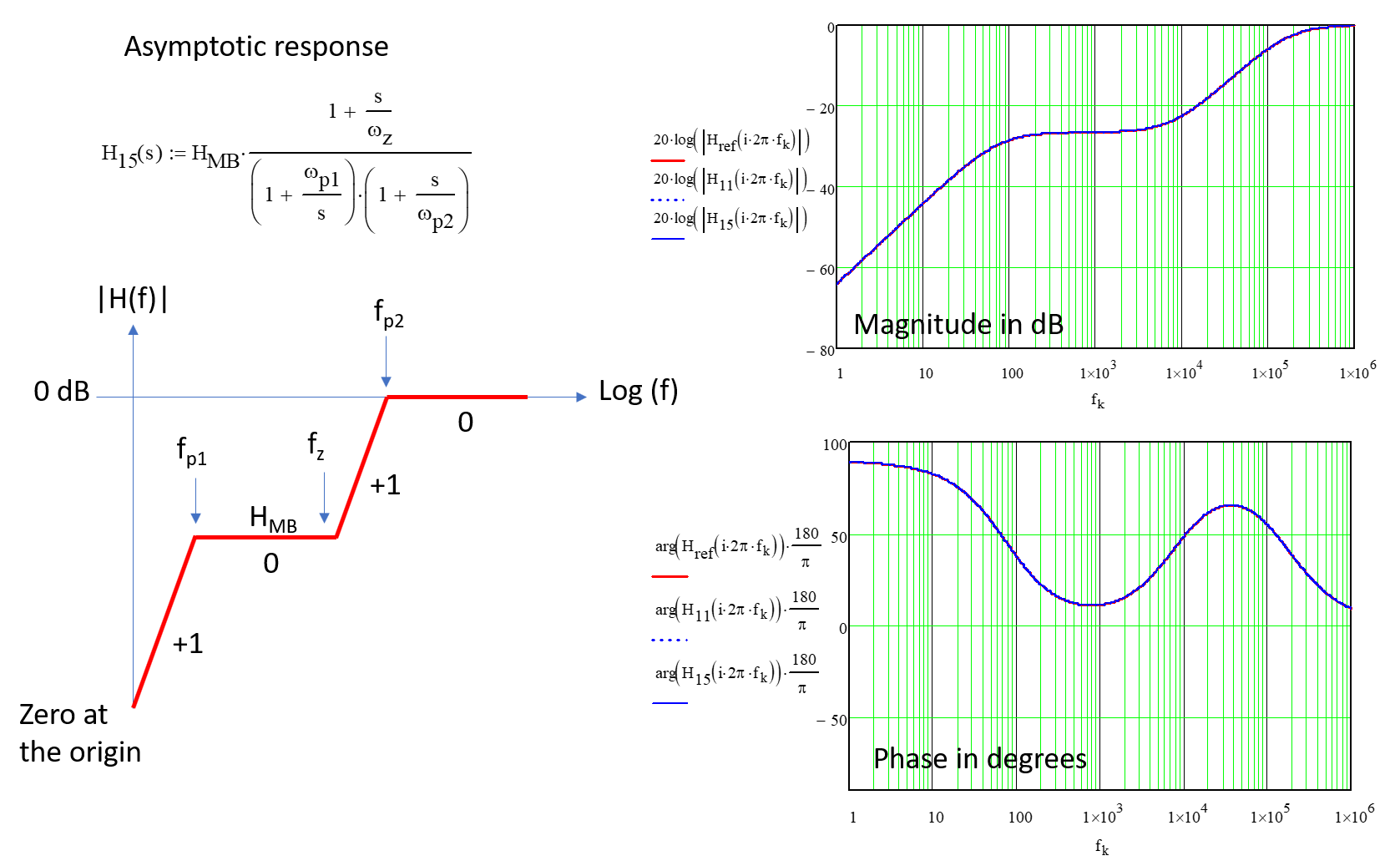
Obtaining a sound expression for the transfer function is usually not the final step when analyzing a passive or active circuit. It is important to shape the result and organize it so that it tells you what it does and how you can use the expression to calculate the components values. These are the principles hidden behind the terms low-entropy expressions and D-OA.