Excuse me, sir,
I'm currently finishing my lab experiment report but I got stuck finding Id and Is of a Diode in reverse-biased mode.
Here is the question: Vin is in the range of 0V to 18V with the step of 2V connected to a resistor with resistance measured around 145.23kΩ and a 1n4007 diode connected in reverse-biased. The simplified schematic is shown below. . 
Here is my data: 
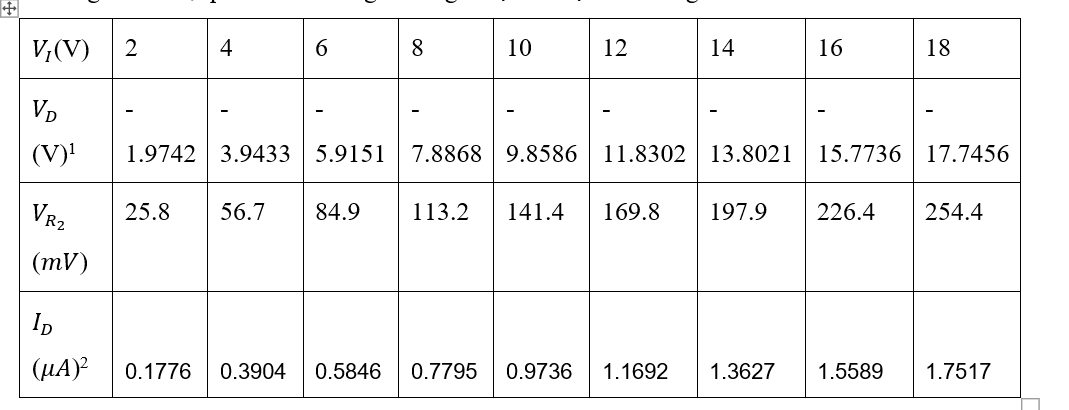Then I apply the formula: Is = Id/(Exp(Id/ηVt)-1). Suppose that η = 1, and T = 303 kelvin. I found ηVt ~ 26.1105mV. Then I had this table: 
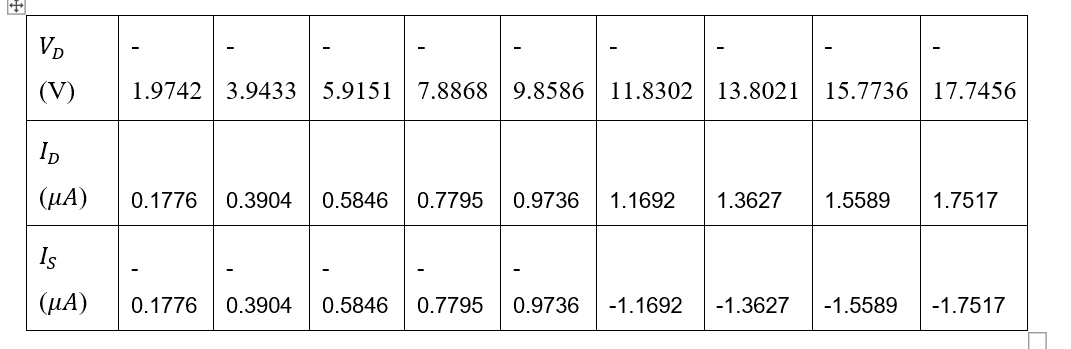It seem like that the Is = -Id. But I don't know why. Can you figure me out what am I wrong?
Thank you very much.
\$\begingroup\$
\$\endgroup\$
2
-
\$\begingroup\$ Do you mean Is = Id/(Exp(Vd/ηVt)-1) rather than Id/(Exp(Id/ηVt)-1)? Assuming you do, then Exp(Vd/ηVt) is the exponential of a sizeable negative number and will be about equal to zero, so Is = Id/(0-1) = -Id, as you have found. The Schottky diode equation predicts that the current through the diode (Id) will be -Is, and that is what you see. A real diode isn't ideal of course. \$\endgroup\$– TonyCommented Apr 8 at 2:05
-
\$\begingroup\$ Oh thank you very much, it is my mistake. \$\endgroup\$– CÔNG NGUYỄN DUYCommented Apr 8 at 16:09
Add a comment
|
1 Answer
\$\begingroup\$
\$\endgroup\$
1
You have written:
$$ I_S = \frac{I_D}{e^{\left( \frac{I_D}{\eta V_T} -1 \right)}} $$
That's incorrrect, \$I_D\$ does not appear in the exponent, and the term should be diode voltage \$V_D\$. This is the corrected equation:
$$ I_S = \frac{I_D}{e^{\left( \frac{V_D}{\eta V_T} -1 \right)}} $$
-
\$\begingroup\$ I really appreciate your answer, sir. Thank you very much. \$\endgroup\$ Commented Apr 8 at 16:10