Is RMS value of a pulse wave practically useful?

Question

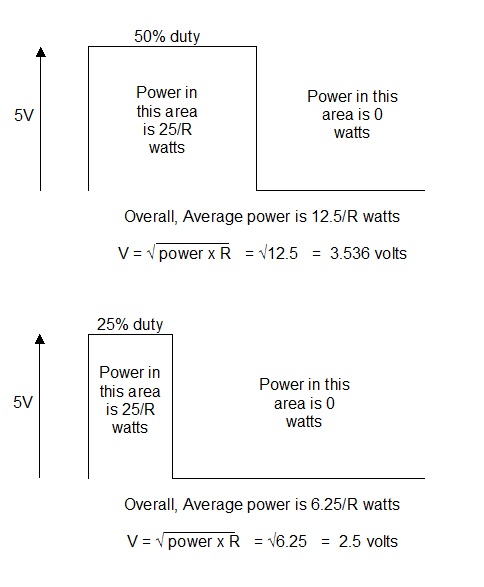
As we know the mean value of a pulse wave (depending on its duty cycle) corresponds to a constant DC.

But when it comes to alternating current through a resistor for DC power correspondance we measure the RMS value i.e. RMS of an alternating current becomes such as the current which lights a bulb as the same intensity as if we have a DC value of the same with that RMS.

I measure pulse wave voltage with Voltmeter's DC settings. I think it gives the mean value.

So my question is if we have a pulse wave does it make sense anymore to talk about its RMS value?

Answer 1

So my question is if we have a pulse wave does it make sense anymore to talk about its RMS value?

Yes, of course it does; the rms value of the pulse wave is the effective DC voltage across a resistor that gives the same average power.

Recall that the instantaneous power associated with a resistor is

$$p_R(t) = \dfrac{v^2_R(t)}{R} $$

The average power, over a period \$T\$, is then

$$p_{avg} = \dfrac{1}{T} \int_0^Tp_R(t)\,dt =\dfrac{1}{T} \int_0^T\dfrac{v^2_R(t)}{R}\,dt$$

Thus, the equivalent DC voltage that produces the same average power is

$$V_{eq} = \sqrt{p_{avg}\cdot R} = \sqrt{\dfrac{1}{T} \int_0^Tv^2_R(t)\,dt}$$

But, that last term is precisely the root of the mean of the square (rms) value of \$v_R(t)\$.

So, yes, it makes sense to talk about the rms value of a pulse waveform or any other voltage or current waveform for that matter.

Answer 2

If we have a pulse wave does it make sense anymore to talk about its rms value?

Yes it does. If the voltage is 50% duty and rises to +1V at the top and -1V at the bottom it will measure: -

• Zero volts on a multimeter measuring DC
• Theoretically 1V on a multimeter measuring AC

You can't rationalize one from the other when they are AC coupled.

And what if they aren't AC coupled - say you measured 2.5V for a 5V square wave - sure, you can predict it is 50% duty but you can't say the heating effect it has is the same as 2.5V DC.

The RMS of a 5V square wave with 50% duty is not 2.5 volts it is \$\sqrt{\dfrac{5^2}{2}}\$ = 3.536 volts: -

Answer 3

The average power going into a system is a meaningful number (it represents the amount of energy per second). If the voltage and current going into a system are always proportional (meaning voltage varies in phase with current) then the average power going into a system will be proportional to the square of voltage and also proportional to square of current. The RMS voltage is essentially the square root of the amount of power that a would be generated across a unit load by a given voltage or current waveform.

In the case of a motor driven by PWM, the voltage and current waveforms are likely to be substantially out of phase, in ways which will vary depending upon various factors including the level of mechanical loading. As such, the power being driven into the motor will be not be proportional to the RMS voltage nor to the RMS current. Within the motor and control system, energy will be shuffled around between places it's put to use (motor's mechanical load), places it's wasted mechanically (bearing friction), places it's wasted electrically (resistance in the motor windings and the control system), and places it's sometimes stored and sometimes harvested (mechanical inertia and electrical inductance). Power dissipation due to winding resistance is proportional to RMS-measured current, pretty much independent of whatever else is going on, but most other kinds of energy transfer will vary in other ways.

With regard to other kinds of loads, the usefulness of RMS voltage as a measure will depend upon the nature of the load. In some cases, simple time-averaged voltage will have a more meaningful relationship with behaviors of interest, while in other cases RMS voltage is what's important.

Answer 4

There are many ways to define "average" when measuring an AC voltage or current. RMS usually makes the most sense, because it is the only way which, for any waveform, preserves equations such as:

$$ E = IR $$

$$ P = IE $$

$$ P = I^2 R $$

$$ P = \frac{E^2}{R} $$

RMS voltage or current answers the question:

What is the equivalent DC voltage or current with the same power into a resistive load as this AC voltage or current?

RMS is the only method that does this for any periodic waveform, be it sinusoidal, square, a pulse train, symmetrical or not, or even totally irregular. You might say that the magic of it is in the square part of root mean square, because power into a resistive load is proportional to the square of current or voltage. Thus, two 50% duty cycle square waves, one from 0V to 5V, and the other from -2.5V to 2.5V, do not carry the same power. Only RMS takes this into account:

$$ \sqrt{\frac{(5V)^2 + (0V)^2}{2}} = \sqrt{\frac{25(V^2)}{2}} = \sqrt{12.5(V^2)} \approx 3.5V $$

$$ \sqrt{\frac{(-2.5V)^2 + (2.5V)^2}{2}} = \sqrt{\frac{6.25(V^2) + 6.25(V^2)}{2}} = \sqrt{6.25(V^2)} \approx 2.5V $$