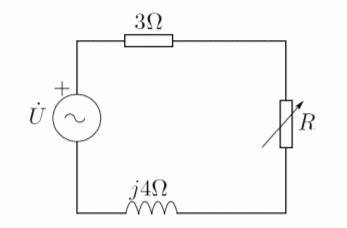
Well, first of all we can write the input impedance as follows:
$$\underline{\text{Z}}_{\space\text{in}}=3+\text{R}+4\text{j}\tag1$$
The input current is given by:
$$\underline{\text{I}}_{\space\text{in}}=\frac{\hat{\text{V}}\cdot\exp\left(\phi\cdot\text{j}\right)}{3+\text{R}+4\text{j}}\tag2$$
The real power of the load is given by:
$$\text{P}_\text{load}=\text{P}_\text{R}=\text{V}_{\text{R/rms}}\cdot\text{I}_{\text{R/rms}}\cdot\cos\left(\varphi_\text{R}\right)=\text{R}\cdot\text{I}_{\text{R/rms}}^2\cdot\cos\left(\varphi_\text{R}\right)=$$
$$\text{R}\cdot\left(\frac{1}{\sqrt{2}}\cdot\left|\frac{\hat{\text{V}}\cdot\exp\left(\phi\cdot\text{j}\right)}{3+\text{R}+4\text{j}}\right|\right)^2\cdot1=\frac{\text{R}}{2}\cdot\left(\frac{\hat{\text{V}}}{\sqrt{\left(3+\text{R}\right)^2+4^2}}\right)^2=$$
$$\frac{\text{R}}{2}\cdot\frac{\hat{\text{V}}^2}{\left(3+\text{R}\right)^2+4^2}=\frac{\hat{\text{V}}^2}{2}\cdot\frac{\text{R}}{\left(3+\text{R}\right)^2+16}\tag3$$
Now, solve:
$$\frac{\partial\text{P}_\text{load}}{\partial\text{R}}=0\space\Longrightarrow\space\text{R}=5\tag4$$
And the real power of the load is there equal to:
$$\hat{\text{P}}_\text{load}=\frac{\hat{\text{V}}^2}{2}\cdot\frac{5}{\left(3+5\right)^2+16}=\frac{\hat{\text{V}}^2}{32}\tag5$$