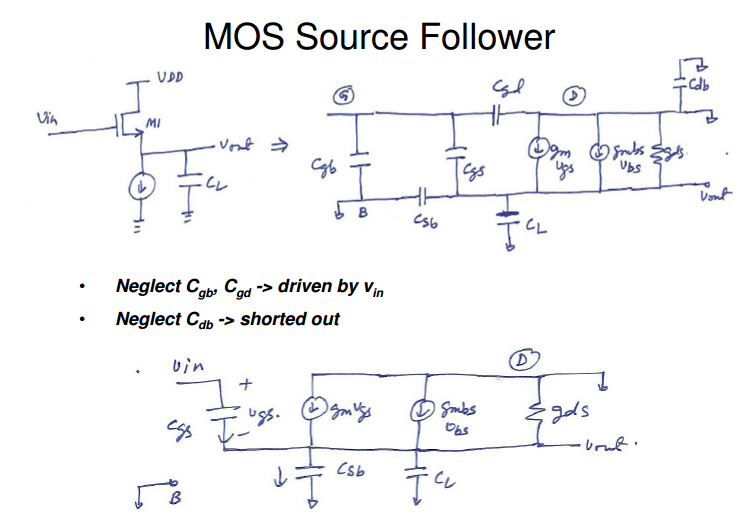
This lecture shows the frequency response of source follower.
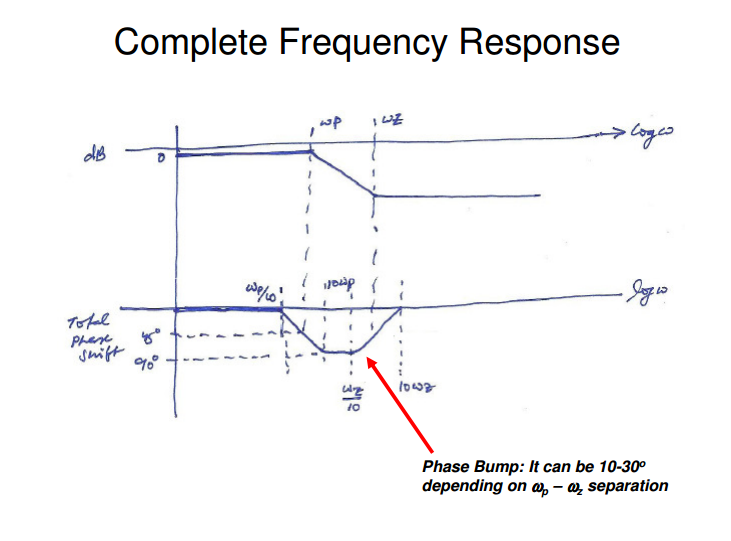
As you can see, the transfer function has one pole, one zero and they are close to each other.
So from the phase plot, we can see that there is a phase bump here. I have heard that the phase bump causes signal distortion.
However, I can't find the explanation anywhere now.
If the pole and zero are far from each other, the output signal is also shifted in phase.
Could anyone explain that? Thank you.
It really depends on what you mean by the term "distortion".
Yes, the bump in the phase response will cause the output waveform to be different from the input waveform. But the non-flat frequency response has that kind of effect, too. But both of these effects are linear and can easily be compensated for, restoring the original waveform.
A more useful definition of "distortion" is any nonlinear effect that cannot readily be compensated for, such a clipping, crossover distortion, slew rate limiting, etc. The effects you're talking about do not fall within this definition.
