90° hybrid coupler (Quarter-wave branch line hybrid)

Question

according to "Foundations for Microwave Engineering" by Robert E. Collin, Lumped Element Quadrature Hybrids" by D. Andrews and several others this is what a Quarter-wave branch line hybrid looks like:

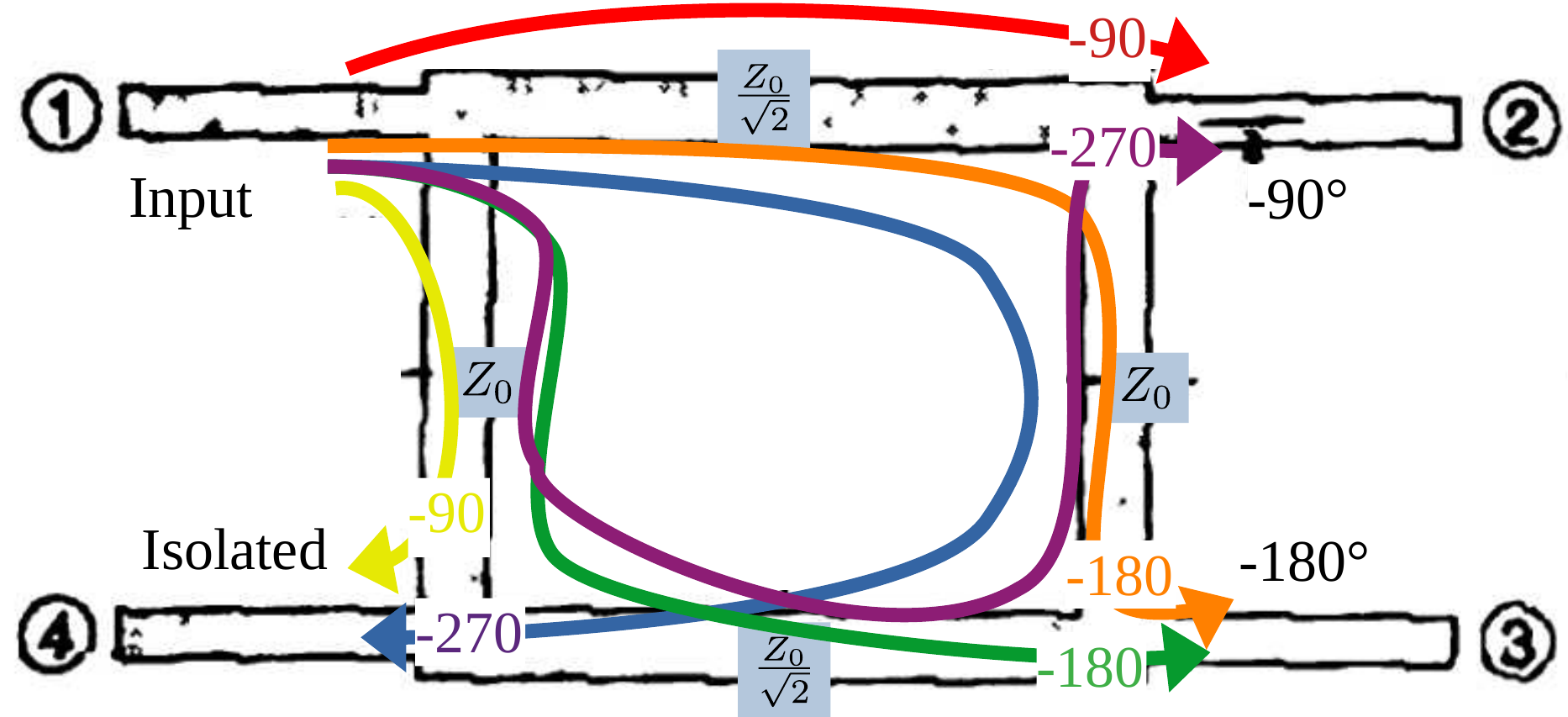
Assuming input is at port 1, port 2 is supposed to have a -90° phase shifted signal, port 3 is supposed to have a -180° phase shifted signal and port 4 is supposed to be isolated i. e. having no signal at all.

I go along with port 3: both signal paths (right-down as well as down-right) encounter the same phase delay of -180° and interfere constructively at port 3 thus creating a -180° phase shifted signal.

I also agree with port 4 being isolated: one signal path (down) shifts the signal by -90° while the other signal path (right-down-left) shifts the signal by -270°. This leads to two signals out of phase by 180° canceling each other at port 4.

My problem is with port 2: while it is supposed to provide a -90° phase shifted signal, my reasoning is that, similar to port 4, one signal is -90° phase shifted while the other signal is phase shifted by -270° and both signals cancelling each other out.

What is the deal here? What am I missing? And why are the horizontal lines of the hybrid supposed to have that specific impedance ratio of those vertical lines? Whats up with that?

Answer 1

What is the deal here? What am I missing? And why are the horizontal lines of the hybrid supposed to have that specific impedance ratio of those vertical lines? Whats up with that?

The -90 and -270 paths are equal opposite in amplitude at port 4, so they cancel. However they are not equal opposite amplitudes at port 2, so there is a signal output.

The reason for this difference is that the λ/4 lines have the impedances that they do.

Intuition only gets you as far as -90 / -180 / -270 phase shifts. Amplitudes (except in the minds of a few gifted individuals) are much harder to intuit correctly in a circuit of this complexity, especially as you have to also account for the loading at ports 3 and 4, when calculating the output at port 2.

Perhaps the most useful and 'magical' thing to bear in mind about 1/4 wave lines is that they invert the output impedance to the input. If a λ/4 line is loaded in a short, it looks like an open. If it's terminated with an open, it presents a short at its input. Generally, if it has impedance Zline, and is loaded with Zload, it presents an input impedance of \$\frac{Zline^2}{Zload}\$.

You could go round the circuit computing what load each node presents, and then inverting that impedance through the line driving it, to load the next node. Rather you than me. The cheating way, the practical way, is to put this circuit into a simulator that can handle transmission lines. Start with all the lines at Z0. You'll see the outputs at port 2 and port 4 are identical. Then vary the line impedances and see what happens. We gain intuition by experiencing a behaviour often enough that it becomes obvious.