RF Narrow bandpass filter (crystal ladder)

Question

I am currently designing a narrow band pass filter at around 8Mhz, using the crystals having a resonance frequency of 8Mhz.

Using the tutorial from :http://www.giangrandi.ch/electronics/crystalfilters/xtalladder.html we manage to get a crystal ladder filter having a passband at around 8Mhz, bandwidth of 400Hz.

However, at high frequency (anything above 70Mhz), the filter response rise up and anything above 70Mhz can pass through. (see the picture)

We do see the response of the filter looks something like the picture below around 6~10Mhz. However, after the 70Mhz, the entire response is different. Is this normal?

How can we make a filter that ONLY pass through 8Mhz and not above 70Mhz?

Answer 1

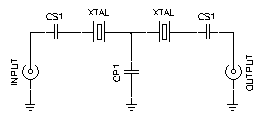
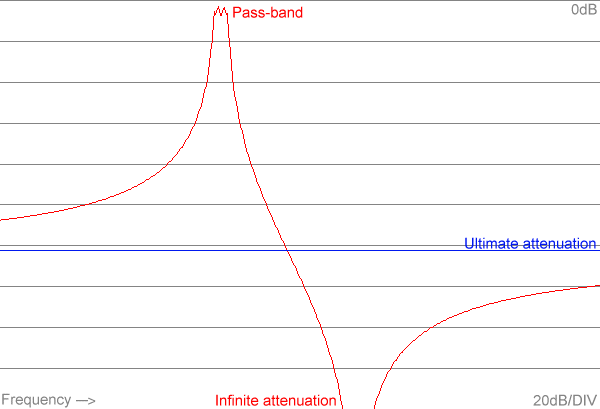
This type of crystal lattice is not meant to be the only source of selectivity in a circuit. At very high frequencies, the parasitic capacitances of the crystal holders and electrodes simply pass everything.

It would be more typical for this sort of lattice to be incorporated into an IF chain that also has ordinary LC circuits to provide the required attenuation farther away from the desired passband.

Additional Detail:

The equivalent circuit for a quartz crystal is something like this:

^{simulate this circuit – Schematic created using CircuitLab}

The components across the bottom represent the mechanical resonance of the crystal itself, while the capacitor at the top represents the capacitance of the electrodes and holder. Typical values are:

• C_ser: 10s of fF (yes, femtofarads, 10^-15F)
• L: 10s of mH
• R: 10s of ohms
• C_par: 10s of pF

The crystal has a series-resonant frequency based on just C_ser and L. It has a relatively low impedance (basically just R) at this frequency.

It also has a parallel-resonant frequency when you consider the entire loop, including C_par. Since C_ser and C_par are essentially in series, together they have a slightly lower capacitance than C_ser alone, so the parallel-resonant frequency is slightly higher. The crystal's impedance is very high at this frequency.

But at frequencies much higher than either of the resonant frequencies, you can see that the impedance of C_par alone will dominate, and this just keeps decreasing with increasing frequency.

Answer 2

Two ways are :

1. augment the wideband attenuation with a separate (probably L-C) bandpass filter as described in Dave's answer.

2. "Neutralise" (to use a 1920s term) the stray capacitance. It was normal to add a couple of extra turns on an RF transformer to generate a low amplitude signal of the opposite polarity to the wanted signal. This was then coupled to the output circuit via a low value trimmer capacitor to cancel out the stray : trimmer would be adjusted for maximum attenuation at the RHS of your analyser screen.

The second approach was patented as the "Neutrodyne" circuit and it can still be useful today.