Load lines and Esaki diodes

Question

I wanted to check something I was working on, and it's an assignment so I don't want the answer, I just want to know if I am approaching it right.

I have an esaki diode. I have a curve (the s-curve that shows Voltage in vs. current. I am trying to figure out how to get 2 stable operating points, with a simple voltage source connected in series with a 250 ohm resistor. I know to calculate a load line, but for some reason I am not getting answers that make sense (I can't seem to get a lad line that intersects the curve that gets me two stable points unless my Vin is zero).

Any pointers? I am using the relation Vin = IdR + Vd, but I am not sure if that even applies here. I just want to see how to figure out how much voltage i need to bias the diode.

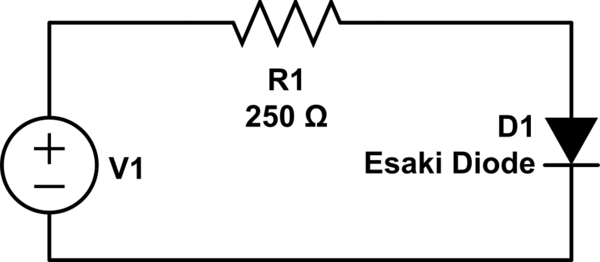
^{simulate this circuit – Schematic created using CircuitLab}

edited to add schematic

Answer 1

Generally you only get one stable operating point per load line, but in the case of an Esaki tunnel diode you can get two. Take a look at this page for some diagrams of what a load line with two operating points looks like: http://people.seas.harvard.edu/~jones/es154/lectures/lecture_2/load_line/load_line.html . Basically, you need a line that intersects the I-V curve in two different places where the I-V curve has positive slope.

The relation $V_in = I_d R + V_d$ relates the I-V curve with the supply voltage and load resistance. This is the correct formula; there should be two pairs of $I_d$ and $V_d$ that fall on the I-V curve of the diode and will satisfy this relationship for a given $V_in$ and $R$, so long as the load line intersects the I-V curve in more than one place.