Calculating Power of a Non-Continuous Signal

Question

I have to find the power of the following signal and would like to know if I'm doing this right or, if I'm doing it wrong, how to do it.

The equation for power in my textbook is \$\overline{m^2(t)} = \lim_{T\to\infty} \frac{1}{T} \int_{\frac{-T}{2}}^{\frac{T}{2}} m^2(t) dt\$

This signal periodically has the equation \$m(t) = \frac{t}{\pi/4} \$

From the picture, \$T=\pi\$

Therefore, this can be simplified to: \$\overline{m^2(t)} = \lim_{T\to\infty} \frac{1}{\pi} \int_{\frac{-\pi}{4}}^{\frac{\pi}{4}} (\frac{t}{\pi/4})^2 dt\$, right?

If the above is correct, then \$\overline{m^2(t)} = \frac{1}{6}\$

I believe this is correct, but only for the part of the signal that exists. Since half of the signal has no value or is zero, does this mean I have to divide the answer I just got by 2? So that \$\overline{m^2(t)} = \frac{1}{12}\$?

Answer 1

An integral is a sum like a + b + c... a, b,c are the values of the function in the part that you are integrating.

Where the value does not exist, it has the value 0. Adding 0 to a sum will not change that sum.

You already take that into account.

You say that the integral in the formula is \$\int_{\frac{-T}{2}}^{\frac{T}{2}}\$ and that \$T=\pi\$, which would lead to \$\int_{\frac{-\pi}{2}}^{\frac{\pi}{2}}\$, but actually the image shows that T = \$\frac{\pi}{2}\$, hence and the substitution is as you have written: \$\int_{\frac{-\pi}{4}}^{\frac{\pi}{4}}\$. This adjusts the lower and upper bound so that the values that are summed up by the integral are those different from 0, because integrating those does not add anything to the integral.

That's why it is wrong to divide by two. In general: if you have a formula for something, use that formula, don't add more calculation to it, because that changes the formula and the result is something else.

Answer 2

please clear following points (still i'll explain the concept needed to solve this question). 1) if it is a textbook question, then limit x tends of infinity, its just to confuse, as we can see in the image, max magnitude is 1. further, the signal is function of "t". please see if any info you missed out.

*************************************solution*************************************** (this solution will help to get through the main concept behind power calculation)

for power you need to obtain the RMS value of signal. when you find the RMS value, you actually square the function.

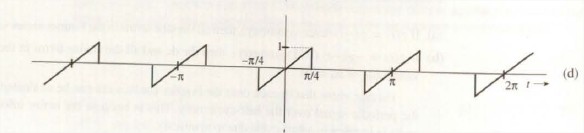
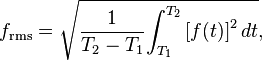fig. this is how you can find rms value for a signal, further, power of signal is found as squared value because there is under-root of rms value, whose integration will be tricky.

now,

power = (1/time period)*{ integration of modulus of rms value of signal w.r.t to time}

---

in your question you are asking if you need to divide the final value by 2, as half of the signal is zero. you notice that signal is divided into 3 sections,

m(t) = t/(pi*.25) , for 0 to pi/4 .................(eq. 1)

m(t) = 0 , for pi/4 to 3pi/4..............(eq. 2)

m(t) = -t/(pi*.25), for 3pi/4 to pi................(eq. 3)

so, period is pi radians. and when you calculate RMS value of m(t) over the period of pi radians, the rms value for (eq. 2) comes out to be ZERO. and once you are done with RMS, value, you can find the power. So during this process, you already considered the portion of the signal whose magnitude is zero. SO, THERE IS NO NEED TO DIVIDE THE FINAL ANSWER BY 2.

hopefully, this answer will be of some help.