0
\$\begingroup\$

I used this calculator to determine that my hand-turned inductor has an inductance of approx. .5 uH.

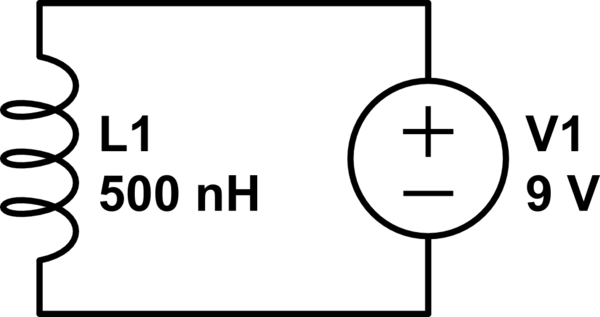
schematic

simulate this circuit – Schematic created using CircuitLab

When I measure this circuit on a multi-meter every node on the circuit is measured in mV. How is this possible? When I use Ohm's Law to get the inductor's resistance it is measured it is measured in thousandths of an ohm.

Why aren't I getting around 9v with a realistic voltage level?

\$\endgroup\$
3
  • 2
    \$\begingroup\$ If you connect a normal 9V battery (that has internal resistance) to an inductance with very low resistance (effectively a short circuit) what do you think happens to the terminal voltage of the battery? \$\endgroup\$
    – JIm Dearden
    Commented May 13, 2015 at 8:13
  • \$\begingroup\$ I'm tempted to say that I'm not pulling any current, so therefore my voltage is proportional according to Ohm's Law? But placing a capacitor there instead works. I can still measure my current. Then afterwords I can watch the voltage drop from the capacitor when I disconnect the battery. Shouldn't the same hold true for an inductor? As the magnetic field (That I'm apparently not producing.) dissipates and converts back into electrical energy? \$\endgroup\$
    – Allenph
    Commented May 13, 2015 at 8:22
  • \$\begingroup\$ Did you measure the current? \$\endgroup\$
    – Criticizing Israel not allowed
    Commented Jul 3, 2018 at 0:50

3 Answers 3

Reset to default
5
\$\begingroup\$

"I'm tempted to say that I'm not pulling any current" That is your error.

When you measure the voltage, your system is in steady state, where the inductance does not count, only the ohmic resistance of your coil, which is very low. A 9V battery has a rather high internal resistance, so you in effect have a voltage divider, and you are measuring the voltage over the leg of the divider that has a very low resistance => you emasure a very low voltage.

\$\endgroup\$
2
  • \$\begingroup\$ This was my solution...i.sstatic.net/y0e5Y.png...however, while I understand how to rectify the problem now, I'm still a little shaky on the "why." And I'm still not getting an electro-magnet...shouldn't I? \$\endgroup\$
    – Allenph
    Commented May 13, 2015 at 8:49
  • 1
    \$\begingroup\$ @Allenph: If you want a quantitative answer you must edit your post to include the length and diameter of the wire in the coil and either a link to the battery's data sheet or a table/plot showing its internal resistance as a function of load current. You are getting an electromagnet, albeit a weak one since you don't have a lot of turns and its core is air. You can check it easily with a regular compass or a magnetized needle suspended at its mid-point with a string. \$\endgroup\$
    – EM Fields
    Commented May 13, 2015 at 9:42
1
\$\begingroup\$

My apologies for the hand-drawn sketch...

This is the equivalent circuit (neglecting the small resistance in the wire and inductor) for the steady-state circuit. The green box is the battery with its internal resistance. As has already been pointed out, the inductor "disappears" in the steady state, so I've drawn it in ghostly grey :).

Now you can see why you see no (or very small) voltage gradients along the wire/inductor.

I'm guessing that your 9V battery is getting very warm as a relatively large current will be flowing through your circuit.

Sketch of steady-state equivalent circuit

\$\endgroup\$
0
\$\begingroup\$

The equation of an (ideal) inductor is.

$$V=L\frac{dI}{dt}$$

So lets say at t=0 we connect an ideal 500nH inductor to an ideal 9V source.

$$9=500*10^{-9}\frac{dI}{dt}$$

$$9=0.5*10^{-6}\frac{dI}{dt}$$

$$\frac{dI}{dt}=18*10^6$$

$$I = \int18*10^6dt = 18*10^6t+c$$

At \$t = 0\$, \$c=0\$ therefore

$$I = \int18*10^6dt = 18*10^6t$$

So one second after connecting the inductor to the battery you should have a current of 18MA. (no that capital M is not a typo)

Back in the real world you don't have an ideal 9V source and you don't have an ideal inductor and for that matter you don't have ideal wires to connect the inductor to the voltage source.

In reality very soon after you connect the inductor to the battery the behaviour will be dominated not by the inductance but by the resistance of the coil and the battery. The coil likely has a much lower resistance than the battery leading to very small voltages being measured.

\$\endgroup\$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.