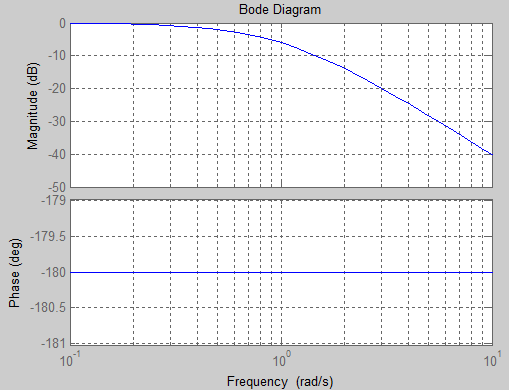
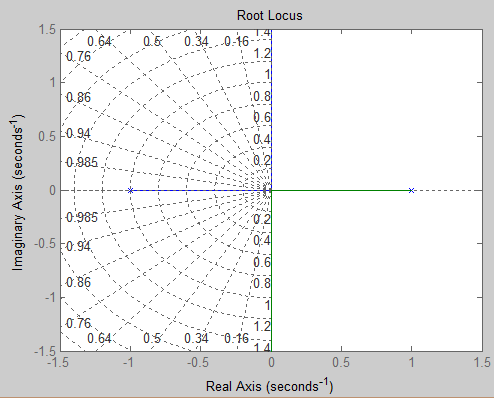
Why is system of the type
$$T = 1/(s^2 - 1)$$ Difficult to control using standard control methods?
When I look at frequency plots, it doesn't seem to give me any important information as to why this system would be difficult to control using classical methods
Can someone who knows control theory (especially the part about compensators or other classical control techniques) inform me as to why this system would be difficult to be controlled using classical frequency based methods?
\$\dfrac{1}{(s^2-1)}\$ factorises to \$\dfrac{1}{(s+1)(s-1)}\$, which has a stable pole at \$s=-1\$ and an unstable pole at \$s=1\$.
If this is the open-loop TF (you don't say if you wish to close the loop around this TF), then it can be stabilised by placing a zero at \$s=-a\$, giving an OLTF:\$\dfrac{s+a}{(s^2-1)}\$, and a CLTF: \$\dfrac{s+a}{s^2-1+s+a}=\dfrac{s+a}{s^2+s+(a-1)}\$, which is stable for \$a>1\$.
This system can't be controlled with a proportional controller or a proportional integral. Other than that general controllers should not have a problem with it such as lead, lag, PD, PID. The only real question is how do you want the system controlled. Do you want no overshoot, do you want zero steady state error, do you want a fast response...?
