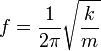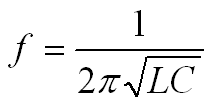The step transient response of a parallel RLC circuit

Question

Let's say an inductor is connected in parallel to a resistor and in parallel to a capacitor. At time t=0 the circuit is connected to a DC current source.The initial stored energy is zero. I have the solution in the text book under the section "The Step Response of a Parallel Circuit".

The current through the inductor is equal to the current of the current source plus a negative exponential multiplied to a sinusoidal function.

I understand that an inductor acts as a short for DC. But for the transient response the current through it will be zero and the current goes through the capacitor and resistor.

My question is where does the sinusoidal term comes from? Are there any physics behind it, or we just get it from analyzing the circuit?

What I am looking for is an answer like this:

When the DC current source is connected to a parallel RLC circuit, the capacitor acts as an open circuit, the inductor acts as short so all the current goes thorough the inductor but this the steady state response. For the instance that we close the switch(transient response) .....???...I am not sure what happens. It seems that the inductor becomes an open circuit(not sure why)and the capacitor and resistor current is negative exponential times sinusoidal (I don't understand this part either)

Answer 1

The step response of your circuit will normally be composed a constant term and exponentials, and the exponentials can take various forms, depending on the relative values of the components.

For example, for relatively large values of resistance the exponentials will be of the form: \$ e^{-\sigma t}\$; \$ e^{-\beta t}\$, which decay to zero over time.

A sine wave, if it exists, arises from Euler's Formula: \$\small e^{jx}=cos(x)+jsin(x)\$.

Thus, for smaller resistance values the exponentials may have complex powers: \$ \small e^{-(\sigma \pm j\omega )t}\$ and these cases can be expressed as: $$ e^{-(\sigma \pm j\omega )t}=e^{-\sigma t} \: e^{\pm j\omega t} = e^{-\sigma t}[cos(\omega t) \pm j\:sin(\omega t)]$$ which is an exponentially decaying sinusoid.

It's interesting to note that if the resistance is zero, \$\small \sigma =0\$, and the response is a pure sine wave.

Answer 2

The mechanical equivalent of an LC resonant circuit can be regarded as a physical mass suspended by a spring: -

_{(source: umt.edu)}

This is well known to anybody from an early age but the oscillation type being sinusoidal doesn't become apparent until you study engineering.

The picture above does not contain any damping (equivalent to a resistor) so the oscillations continue forever. If damping is applied this happens (shown from a different angle: -

The "spring" is the green thing that ironically looks like a resistor. The red square is the "mass" running on a notionally friction-less bed and the blue rectangle is the dampener.

Formulaically the spring-mass system has a resonant frequency that is: -

k is spring stiffness and is equivalent (depending on how you regard force as an electrical analogue) to the inverse of inductance. M is equivalent to capacitance.

And for an LC circuit the formula is: -

Answer 3

Physical explanation for this is oscillation of energy during the transient. Voltage-current relation for inductor and capacitor is opposite (current through inductor lags voltage by 90 and through capacitor leads voltage by 90). During transient time, in frequency domain there is "infinite" frequency spectrum of harmonics (current and voltage), so we have some currents go through inductor some through capacitor and some through resistor, and because of phase difference current and voltage are oscillating, until transient is finished and we get steady state. Negative exponential multiplied with sinusoidal component is because we lose high frequencies when transient is over during time. I hope this is simple physical explanation.