Why is CMRR infinite for coupled differential amplifiers when Re increases ?? I cant seem to understand what happens on making Re infinite.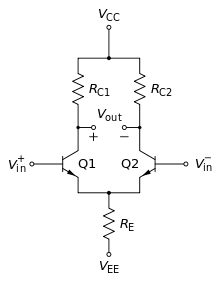
-
\$\begingroup\$ This circuit is confusing regarding Re as here the biasing current through Re also changes with Re's value. It is easier to separate the two, replace Re with a current source and then consider two cases: 1) an ideal current source so Re is infinite 2) a less then ideal current source where Re has a certain value (Re is in parallel with the current source). Think about the CMRR for both cases. You might need to draw a small signal equivalent circuit. \$\endgroup\$– BimpelrekkieCommented Aug 16, 2016 at 11:59
-
\$\begingroup\$ i still dont understand .. \$\endgroup\$– JeffyCommented Aug 16, 2016 at 12:22
-
\$\begingroup\$ Since you don't even know what gm is, no wonder. \$\endgroup\$– BimpelrekkieCommented Aug 16, 2016 at 14:34
-
\$\begingroup\$ yes i didnt know what gm is because im 'studying basic'.. and this topic was something new for me . Im a student so most of my concepts are weak .. but thanks for that help \$\endgroup\$– JeffyCommented Aug 18, 2016 at 12:00
3 Answers
For a single common-emitter transistor amplifier, voltage gain boils down to collector resistor divided by emitter resistor. The bigger the emitter resistor the smaller the gain.
When applied to a differential amplifier (aka long-tailed pair) the common mode gain is in fact the gain of the single transistor so, if the emitter resistor is very high compared to Rc then common mode gain is very small.
Given that the common mode rejection for a perfect long-tailed pair with the output taken differentially across the collectors is theoretically 100% for any size emitter resistor (within reason), any slight imbalance in the transistors doesn't contribute a massive common-mode signal at the output but, as explained above, whatever imbalance there is will be eradicated by a high value of Re.
In fact, most op-amps (if not all?) will use a constant current source for Re and, if you did some research, you'd find that a perfect constant current source has infinite impedance.
According to the definition of the CMRR (CMRR=Adiff/Acm) , the common-mode gain Acm must be zero for CMRR approaching infinite. The common-mode gain Acm is defined for two equal input signals (common-mode signal) at both input nodes.
Now - see what happens when there is a common-mode input signal only: The increasing base-emitter voltage causes an corresponding and equal increase in both emitter currents. This will cause a corresponding emitter voltage increase (effect of emitter degeneration - as known from the classical common emitter stage). This effect can also be explained as signal feedback, which causes a gain reduction. However, due to two emitter currents through a common emitter resistor Re the emitter resistor enters the gain formula (common-mode gain Acm) with a factor of two:
Acm=-gmRc/(1+2gmRe)
This expression clearly shows and explains why the common-mode gain goes down for larger Re values (and CMRR goes up correspondingly).
If the ohmic resistor is replaced by a transistor-based current source the value of Re is to be replaced by the dynamic source resistance rout of the current source which can be made much larger than a suitable ohmic resistor Re. For an ideal current source (infinite rout - not realizable in practice) the gain Acm would go down to zero and the CMRR goes to infinite.
-
\$\begingroup\$ Ok and in that common -mode gain formula Acm= -gmRc /(1+ 2gmRe) ... what is gm?... is that beta gain @LvW \$\endgroup\$– JeffyCommented Aug 16, 2016 at 13:33
-
\$\begingroup\$ Sorry - I have automatically assumed that you are familiar with the commonly used symbol for the transconductance gm=d(Ic)/d(Vbe) which is nothing else than the slope of the exponential relation (Shockleys equation) Ic=f(Vbe). The parameter gm is the key parameter for converting any input voltage change into a corresponding output current change. \$\endgroup\$– LvWCommented Aug 16, 2016 at 14:00
-
\$\begingroup\$ what is gm? Geez, and you're asking about CMRR ?? I suggest you go back to the small signal model of a BJT and get studying. CMRR in diffpairs is for people that do understand gm. \$\endgroup\$ Commented Aug 16, 2016 at 14:33
Look at it like this: For any change in common mode voltage(Vcm) you ideally do not want the tail current to change. Why? because if the the tail current increases for changing Vcm, that will obviously change the collector currents of both the transistors. Remember that the collector currents flow through the collector resistors so that in turn changes the output voltage. This can be thought of as the common mode gain(bad).
This is where the output impedance of the tail current source (a resistor in your case) is important. When you apply a dVcm, a voltage of approximately dVcm-0.7v appears across the emitter resistor (note how we are now analyzing each branch as a emitter follower); So the current through the emitter tail resistor is dItail = dVcm-0.7/Re! Now note how dItail is function of dVcm, and as explained in paragraph above, a change in dItail will change the output voltage. So simply put, Vout is function of Vcm.
Now take Re to infinity, which is the approximation we take as the output impedance of an ideal large signal current source. In this ideal case, a change in Vcm does not change the tail current and thus the output voltages. So Avcm is 0. CMRR = Adiff/Avcm, as Avcm tends to 0, CMRR tends to infinity.
-
\$\begingroup\$ yes I understand now, and replacing Re with a 'constant current bias' will maintain the tail current constant cuz of its high impedance. Can you tell me one more thing .. ''voltage follower acts like a buffer and its output impedance is low ... but what will be the benefit of using it as an "input to an inverting amplifier"??" \$\endgroup\$– JeffyCommented Aug 18, 2016 at 12:13
-
\$\begingroup\$ Indeed. Infact, Re is usually very large as we try to imitate the ideal infinite output impedance of a large signal current source! Yes i can tell you one more thing..? \$\endgroup\$– MAMCommented Aug 18, 2016 at 12:20
-
\$\begingroup\$ ''voltage follower acts like a buffer and its output impedance is low ... but what will be the benefit of using it as an "input to an inverting amplifier"??" \$\endgroup\$– JeffyCommented Aug 18, 2016 at 12:23
-
\$\begingroup\$ This question is not related to this one. You should really research around this topic more and ask another question if necessary. However, the answer is this: I assume you are aware that a voltmeter must have high input resistance so it does not load the source its trying to measure? Same goes for an amplifier, you do not want it to load and thus change the signal you are trying to amplify, so you need high input impedance! Remember, generally a buffer has HIGH input impedance and LOW output impedance. \$\endgroup\$– MAMCommented Aug 18, 2016 at 12:27
