RHP zero in boost converter

Question

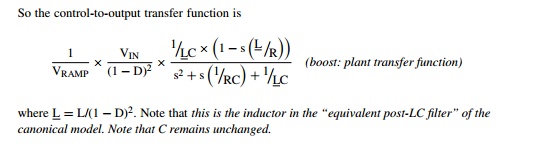
Below is the control to output transfer function of a boost converter. (source: Switching Power Supplies A - Z by Sanjaya Maniktala here at page 286)

And this is one part talking about the RHP zero the book. I don't understand the last part from "Eventually...".

When output voltage dips, the controller adjusts to increase duty cycle. However, by increasing duty cycle the time to transfer energy to load decreases.
If everything goes on like this, finally the duty cycle will go to 1 and no time for transferring energy to load.
But the note below says that eventually the inductor current ramps up to the right level and the strange behavior gets corrected.
Why duty cycle not go to 1?

Note: Intuitively, the RHP zero is often explained as follows — if we suddenly increase the load, the output dips slightly. This causes the converter to increase its duty cycle in an effort to restore the output. Unfortunately, for both the boost and the buck-boost, energy is delivered to the load only during the switch off-time. So, an increase in the duty cycle decreases the off-time, and there is now, unfortunately, a smaller interval available for the stored inductor energy to get transferred to the output. Therefore, the output voltage, instead of increasing as we were hoping, dips even further for a few cycles. This is the RHP zero in action. Eventually, the current in the inductor does manage to ramp up over several successive switching cycles to the new level consistent with the increased energy demand, and so this strange situation gets corrected — provided full instability has not already occurred!

Answer 1

The continuous mode boost and buck-boost converters exhibit control-to-output transfer functions \$G_{vd}(s)=\hat{v}(s)/\hat{d}(s)\$ containing two poles and one RHS (right half-plane) zero, called zero of nonminimum phase.

Your original transfer funtion is:

$$G_{vd}(s)=\frac{1}{V_{RAMP}}\times \frac{V_{IN}}{\left ( 1-D \right )^2}\times \frac{1/\underline{L}C\times\left ( 1-s\left ( \underline{L}/R \right ) \right )}{s^2+s\left ( 1/RC \right )+1/\underline{L}C}$$

Starting from a simpler function (without the RHP zero), named \$ G_{vd}'\$:

$$G_{vd}'=\frac{1}{V_{RAMP}}\times \frac{V_{IN}}{\left ( 1-D \right )^2}\times \frac{1/\underline{L}C}{s^2+s\left ( 1/RC \right )+1/\underline{L}C}$$

Or, placed as a standard second order t.f:

$$G_{vd}'=K_{DC}\times \frac{1/\underline{L}C}{s^2+s\left ( 1/RC \right )+1/\underline{L}C}$$

where the DC gain is \$K_{DC} =\frac{1}{V_{RAMP}}\times \frac{V_{IN}}{\left ( 1-D \right )^2}\$.

The equation can be rewritten as:

$$G_{vd}'=K_{DC}\times \frac{1}{\left ( \frac{s}{\omega_0} \right )^2 + \frac{s}{\omega_0Q}+1 }$$

With \$\omega_0=1/\sqrt{\underline{L} C}\$ and \$\omega_0Q=R/\underline{L}\$

Similarly, the original transfer function can be expressed as (RHP zero included):

$$G_{vd}=K_{DC}\times \frac{\left (1-\frac{s}{\omega_{RHP}} \right )}{\left ( \frac{s}{\omega_0} \right )^2 + \frac{s}{\omega_0Q}+1 }$$

The response will be:

$$\hat{v}(s)= \frac{K_{DC}}{\left ( \frac{s}{\omega_0} \right )^2 + \frac{s}{\omega_0Q}+1}\times\hat{d}(s) - \frac{K_{DC}/\omega_{RHP}}{\left ( \frac{s}{\omega_0} \right )^2 + \frac{s}{\omega_0Q}+1 }\times\hat{d}(s)\times s$$

The response of the original system is the sum of two components: The first is equivalent to the modified system response (without the zero) and the second is the derivative (scaled) of that one. For the case of a stable system with a step input in \$t = 0\$, this last component will have substantial influence at the beginning and then will vanishes when \$t\rightarrow\infty \$. Note the negative sign leads to a momentary opposite effect in output (nonminimum phase).

UPDATE:

The presence of zero RHP in the model is explained as follows: For the output voltage to increase, the duty cycle must be increased in such a way that the inductor will be disconnected from the load for a long time, causing the output voltage to drop (i.e. in the opposite direction to the one desired). The controller must be designed to meet the project requirements and avoid oscillations while maintaining duty cycle below an undesirable 100% - being limited by the PWM integrated circuit itself.

Answer 2

Perhaps an intuitive example, rather than numeric can help?

Assume continuous mode operation (i.e. inductor current does not drop to zero during the 'off' time), and consider a step increase in duty cycle with a constant current load.

The inductor current will rise with each switching cycle (as it is now subject to more volt-seconds while the switch is on than when it is off), after a few cycles the quantity of charge Q (I*t) delivered to the output capacitor each cycle exceeds Q used by the load, so the output voltage starts rising. Once the boost voltage (less resistive losses) exceeds Vin/(1-D), the inductor current will fall until equal to the load current.

If the control loop has too much gain at high frequency, it could reduce the duty cycle faster than the inductor current can rise, creating an unstable condition.