JFET - transconductance, gain (μ), and internal resistance at the drain

Question

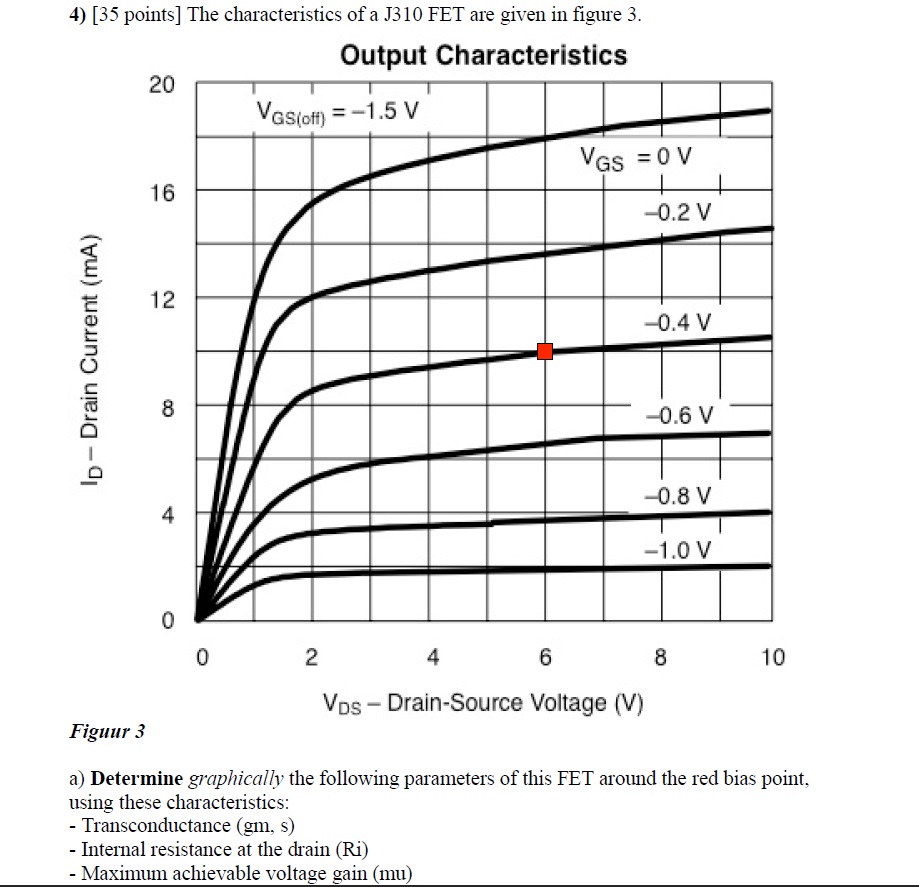
Here is an assignment: to determine gm, internal resistance at the drain, and maximum gain (μ).

Can transconductance gm be found from the formulas and graph?
How to determine graphically the internal resistance at the drain? As I understand it, it is: r'ds = delta V(DS)/delta I(D). How to determine the delta V(DS) from the graph?
In the end it is: A(v) = gm*r'ds. But how to determine μ with the given data?

Resistance should be voltage divided by current. So it can't be \$\Delta V_{ds}/\Delta V_{gs}\$. — The Photon, Commented Jun 13, 2017 at 21:15

analogsystemsrf · Accepted Answer · 2017-06-14 03:19:22Z

1

MU at 6 volts drain is just [Id(Vgs = 0.6v) - Id(Vgs = 0.2v)] / (0.6v - 0.2v), or

(13.5ma - 6.5ma) / 0.4v = 7ma/0.4 = 7ma*2.5 = 17.5mA/volt

answered Jun 14, 2017 at 3:19

analogsystemsrf

35k2 gold badges20 silver badges48 bronze badges

\$\begingroup\$ but this is the calculation of gm (transconductance), not mu (gain) \$\endgroup\$
– Tomas
Commented Jun 14, 2017 at 11:38
\$\begingroup\$ gm and rd are Norton's equivalent. Mu is what you get if you turn it into Thevenin's one i.e. mu=gm*rd \$\endgroup\$
– carloc
Commented Jun 14, 2017 at 12:01
\$\begingroup\$ yes, mu = gm*rd. But "[Id(Vgs = 0.6v) - Id(Vgs = 0.2v)] / (0.6v - 0.2v), or (13.5ma - 6.5ma) / 0.4v = 7ma/0.4 = 7ma*2.5 = 17.5mA/volt" - is the clear calculation of gm. delta Id / delta Vgs \$\endgroup\$
– Tomas
Commented Jun 14, 2017 at 13:51

Add a comment |

1 Answer 1