voltage booster current draw

Question

My input source is 3x1.5V alkaline batteries.

The input power supply is then connected directly to a 12V voltage boost regulator. I used my multimeter to measure the current draw of my load and it reads 200mAH. (The multimeter is connected series after the regulator and before the load)

Question: How long will my batteries last assuming they have a total capacity of 900mAH? And will the current draw be the same if I used a 12V power supply non boosted? (Assuming the voltage booster has an efficiency of 100%)

My thinking is that since the voltage is boosted, in order to maintain the same power rating, the current draw will increase, which makes the actual current draw 533mAH in this case. Please advise!!

Answer 1

My input source is 3 x 1.5 V alkaline batteries.

OK. 4.5 V nominal supply.

I used my multimeter to measure the current draw of my load and it reads 200 mAH.

Check your multimeter. It won't have a mAH range. You meant 200 mA.

How long will my batteries last assuming they have a total capacity of 900 mAH?

The current drawn from the batteries will be scaled up by the step-up ratio divided by the efficiency. Let's assume 80% efficiency: \$ I_{batt} = \frac {12}{4.5}0.2 \frac {1}{0.8} = 0.67 \ \text A \$.

The batteries should last \$ \frac {990 \ \text {(mAh)}}{670 \ \text {(mA)}} = 1.5 \ \text {h}\$

And will the current draw be the same if I used a 12 V power supply non boosted? (Assuming the voltage booster has an efficiency of 100%).

The current drawn at 12 V would be the same - but you won't find a 100% efficient booster.

My thinking is that since the voltage is boosted, in order to maintain the same power rating, the current draw will increase, which makes the actual current draw 533 mAH in this case.

Your calculation is correct (for 100% efficiency) but your units are wrong. 533 mA.

Answer 2

Your assumption is correct except for battery resistance and converter efficiency which increases your primary current and varies with cutoff voltage. This results in 50 to 60% error.

If you look up the Watt-hour rating vs time of discharge for Alkaline cells and examine the cutoff chosen for 3 cells matches or exceeds the lowest input range of your step-up converter.

You now have your Energy source =Wh x N cells

Then compute your load Power in Watts. since Energy is Power * time , the result is your energy draw time until cutoff voltage is reached. Step$up Voltage energy transfer efficiency might vary somewhat near cutoff since the battery resistance rises sharply.

Wh/h=h then times efficiency yields your estimated discharge time .

Normally battery ratings are done for 20h discharge periods, so reducing to near 1h and less leads to a loss of capacity due to the greater batteries ESR*I^2 losses which are heat and not recoverable. Thus is why Wh capacity may reduce to higher load currents.

Details

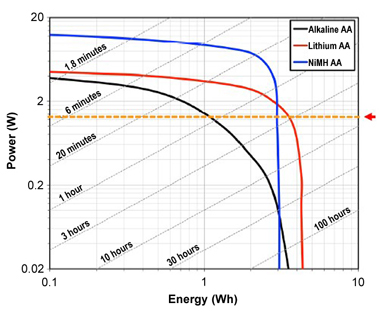
If we assume the average voltage for a constant current you cells may have a 20 h energy capacity of 1.25VxAh=Wh (from 1.6 to 0.9V). we don’t know why you said “total” capacity because cells in series have an Ah capacity of the weakest cell, and NOT the total of all three. In any case your 900mAh seems low as AA alkaline may have 2.4Ah @25’C for 20h load which is reduced to less than half in 1h loads. this is due to the much higher ESR of Alkaline compared to Lithium and NiMH.

So if you see the 20h Energy capacity vs 1h capacity, you may have 3x energy capacity from voltages adding but current limited by the weakest cell.

Assuming your value is for a std 20h discharge rate compare 900mAh*2.25V=2.0Wh to 2.4Wh per cell on this chart and then later x3 for 3 cells.

ref

Notice the 20h interpolation for the black curve of Alkaline AA intersects with 2.2Wh per cell and at 1h it is only 1.1Wh or 1.1W for 1h per cell. or 50% of rated capacity, which is coincidental to orange arrow.

It is not a simple solution, but it is more accurate.