Load resistance in voltage regulator

Question

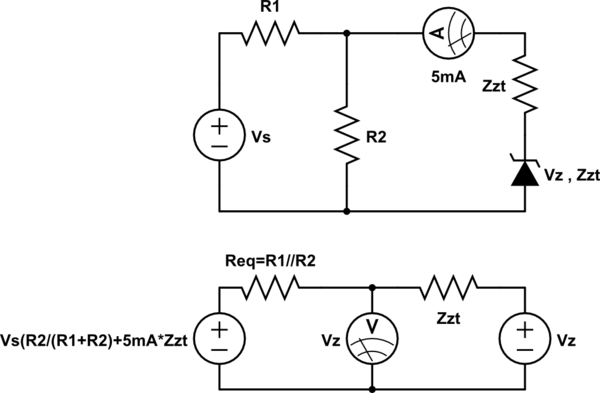
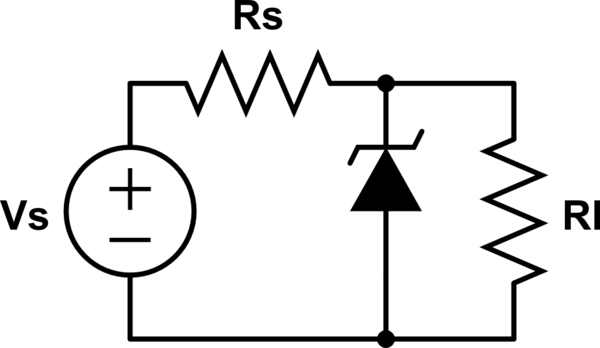
I want to find the minimum resistance \$R_L\$ so as to maintain \$V_z\$(voltage of the zener corresponding to the minimum current \$I_{z_0}\$) across the same resistor \$R_L\$.

^{simulate this circuit – Schematic created using CircuitLab}

I have two ways of looking at this, one of them is wrong and I need your help to figure it out!

First method:

I take off the diode as the load and find thevenin voltage : $$V_{th}=\frac{R_L}{R_l+R_s}V_s$$

Since

\$V_{th}=V_{R_L}\$

I'll equate it with \$V_z\$ which gives :

\$\frac{R_L}{R_L+R_S}V_S=V_z\$

thus

\$R_L=\frac{R_S.V_z}{V_S-V_z}\$

Second method:

If \$R_L\$ is minimal then \$I_L\$ passing through it would be maximal and \$I_z\$ minimal (\$= I_{z_0}\$) thus we'd have :

$$I_S = I_L+I_{z_0} \Leftrightarrow \frac{V_S-V_z}{R_S}=\frac{V_z}{R_L}+I_{z_0}\Leftrightarrow R_L=\frac{R_S.V_Z}{V_S-V_Z-R_S.I_{z_0}}$$

(I'm skeptical concerning the implication \$I_L\$ maximal \$\Rightarrow\$ \$I_z\$ minimal, or in other words \$I_z = I_{z_0}\$)

Answer 1

A typical 300mW Zener uses a test current Izt=5mA with an incremental resistance Zzt and a threshold current, at 1/10 Izt or It @ 0.5mA which results in a knee resistance of Zzk approx. 10x Zzt.

The same is true for LEDs.

Make a Thevenin equivalent circuit for 5mA at a low power.
This gives the low rated resistance, Zzt and is important to include in the equation to solve for R1.

^{simulate this circuit – Schematic created using CircuitLab}

Now you can easily solve for R1

Answer 2

\$ V_S = V_{R_S} + V_Z\$

\$ V_S = I_S\cdot R_S + V_Z\$

\$ I_S = I_Z + I_L\$

\$ V_S = (I_Z + I_L)\cdot R_S + V_Z\$

\$ I_L = \dfrac{V_Z}{R_L}\$

\$ V_S = (I_Z + \dfrac{V_Z}{R_L})\cdot R_S + V_Z\$

\$ R_S =\dfrac{V_S - V_Z}{I_Z + \dfrac{V_Z}{R_L}} \$

\$ R_S =\dfrac{V_S - V_Z}{I_{Z_0}+ \dfrac{V_Z}{R_{L_{min}}}} \$

EDIT: It's not what your asking for...wait a minute...

\$R_{L_{min}}=\dfrac{R_S\cdot V_Z}{V_S-V_Z-R_S\cdot I_{Z_0}}\$

I guess you got the same.