Simulating matching op-amp input impedance with offsets

Question

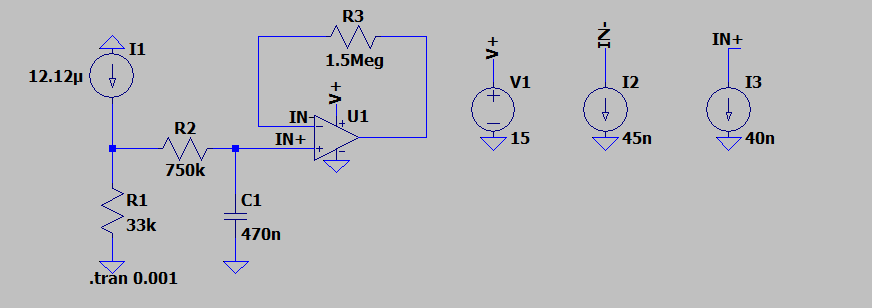
I have a current source with RC filter at the input of an LM324 op-amp configured as a buffer. The Input Biasing Current is 45nA and the Offset Current is 5nA for the LM324.The input resistor is 750k and normally a 750k resistor in the feedback loop would balance the inputs. With the output connected directly to IN- there is about a 30mV difference between the input and the output, where the input is about 400mV. However to get the circuit to provide a gain of 1 I doubled the feedback resistor to 1.5Meg. The result is much better and the output error is much smaller. Why is it that double the resistor was needed here and not 750K?

Also I am trying to simulate this behavior in LTSpice using the circuit below and have included the offset input currents. However a direct connection from output to IN- gives a gain of 1 and does not indicate real life results. is there a better way to model this?

Thanks.

Edit 1:

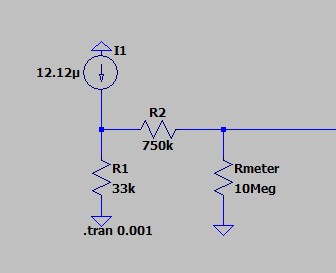
Meter at IN+ creating a voltage divider with R2 and creating a voltage drop. When the same meter is used to measure the output the voltage divider disappears. Tried with 2 meters simultaneously and input and output were closer. Still has the voltage drop, but they are the same.

Answer 1

With the output connected directly to IN- there is about a 30mV difference between the input and the output, where the input is about 400mV.

Measuring a high impedance node with a voltmeter can result in a significant error. The input node impedance is around 783 kohm and your meter might have an input impedance of around 10 Mohms so, when you put your meter at the input your new effective impedance will be: -

$$\dfrac{1}{\frac{1}{783k} + \frac{1}{10M}}$$

This equals 726 kohm and would naturally reduce 400 mV to 371 mV. Here's your 30 mV difference.

However to get the circuit to provide a gain of 1 I doubled the feedback resistor to 1.5Meg. The result is much better and the output error is much smaller. Why is it that double the resistor was needed here and not 750K?

Fix the basic problem of not using your meter in parallel with a large impedance. I would also choose a much better op-amp than the LM324 because it has an input offset voltage of 3 mV and if you require accuracy, 3 mV may be too much of an extra uncertainty. Also, if you require good AC performance you might find the LM324 somewhat unable to deliver the goods. Also there is no mention in the LM324's data sheet what resistive input impedance it has over and above the leakage currents from either input pin.

is there a better way to model this?

Model the effective input resistance of your meter at the op-amp +INPUT.