2
\$\begingroup\$

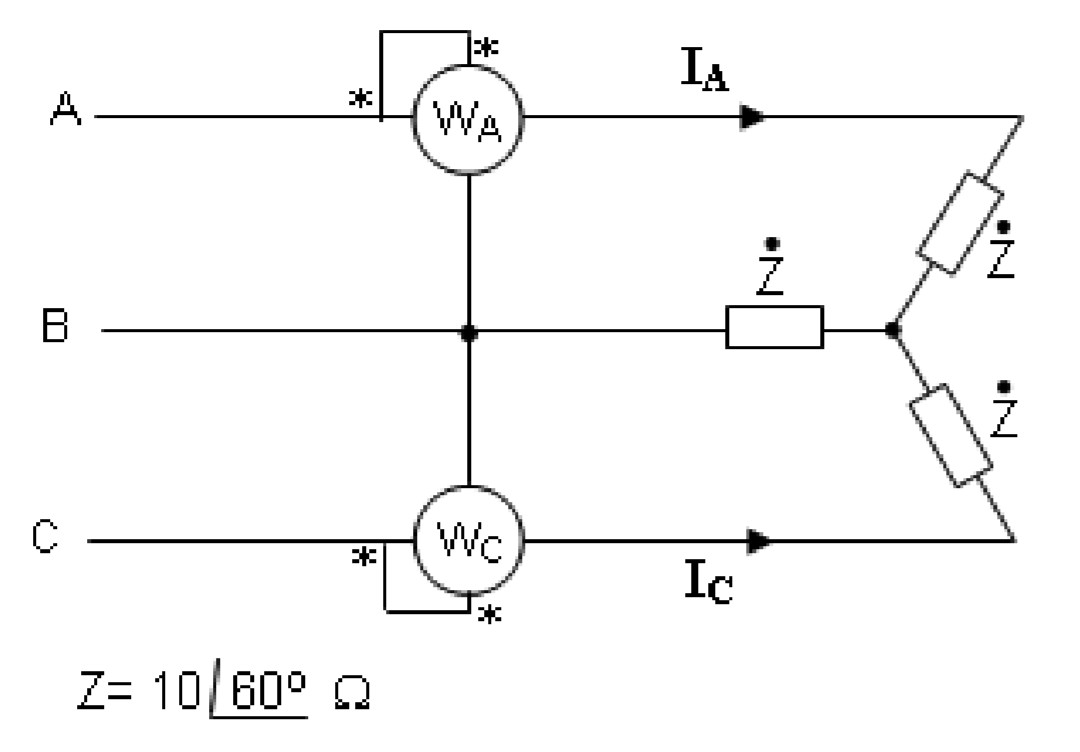
Diagram

On the above picture, VAB is 380 V, balanced loads.

The right answer says power on Wattmeter A is 0 and power on wattmeter C is 7240 W.

This question came from a previous admission test from a power company

I need help understanding why the zero.

Also, why do I have to ignore the phase of the source ?

The following was edited. See edit history if necessary

I tried:

VAB²/(2*Z)

380<0² / 2*10<60

Which results in 7220 (not 7240, but I believe it's an approach error).

Also, kindly answer another question I made, which I believe is related:

Relation between phase angle and current direction

The answers there don't seem, to my eyes, related to the question. And they came before I enhanced the question.

\$\endgroup\$
7
  • \$\begingroup\$ Unless I'm really missing something, the power reading on W_A and W_C must be equal as it's a balanced supply and load. Are the W things wattmeters? \$\endgroup\$
    – Transistor
    Commented Dec 3, 2019 at 22:24
  • \$\begingroup\$ Correct: wattmeters, balanced loads. I suppose that two currents are entering the load and another one is leaving. That's why I linked the other thread I made. This question came from a power company admission test \$\endgroup\$
    – Lucas BS
    Commented Dec 3, 2019 at 22:26
  • \$\begingroup\$ The "right answer" looks wrong. Apart from that, the power calculation must be done on the phase-neutral voltage if you are using Z as the load because the load on each phase is between phase and neutral so your VAB²/Z + VBC²/Z + VCA²/Z line doesn't look right but your next one does. \$\endgroup\$
    – Transistor
    Commented Dec 3, 2019 at 22:34
  • \$\begingroup\$ Corrected the attempted calculations (left intact the part you quoted so your comment makes sense). The "right answer" can be trusted. This came from a test applied STATEWIDE three years ago. The company DOES nullify questions when their alternatives/answers are messed. \$\endgroup\$
    – Lucas BS
    Commented Dec 3, 2019 at 22:46
  • 1
    \$\begingroup\$ I see your edit now. You can't do VAB²/2*Z because you're omitting the effect of \$I_C\$ on the Z on line B. Use $$ \frac {\sqrt 3 V_{AB}^2}{Z} $$ (I think!). \$\endgroup\$
    – Transistor
    Commented Dec 3, 2019 at 23:03

1 Answer 1

Reset to default
2
\$\begingroup\$

This answer was edited. If needed, see history

It is known that the sum of the two wattmeters is the total power of the load, but it doesn't mean that both of them will present the same value, since the sources are out of phase. As noted by Transistor, the wattmeters read the lines in sequence.

Found a document with an exercise. Refer to example 20.1 or 20.3

https://nptel.ac.in/content/storage2/courses/108105053/pdf/L-20(NKD)(ET)%20((EE)NPTEL).pdf

Also, from the same document:

PF=1 → W1=W2
PF=0.5 (cos60) → W1=Total power
0.5<PF<1 → W1>W2

For the proposed problem:

Total power (all three loads):

P=|VL|²/R*cos(<Z)
OR
P=3*|Vp|*|Ip|*cos(<Z)

Line power:

W1=|VL|*|IL|*cos(<V+(|<I|))
W2=|VL|*|IL|*cos(<V-(|<I|))

With <V being the angle between the Line voltage and the Reference (usually, the angle is 30°) , and |<I| being the positive angle between the Line current and the Reference

In my case:

W1= 380*220/10*cos(30+60)= 0
W2= 380*220/10*cos(30-60)= 7240

I wish I could find a shorter formula that takes the angles into account (0, -120, 120) instead

This answer is free to be improved

\$\endgroup\$
3
  • 1
    \$\begingroup\$ I haven't checked your calculations but I have realised that while the supply and load are balanced the metering isn't. If \$ W_A \$ and \$ W_B \$ were referenced to the next phase in the sequence (rather than both referenced to B) then they would read the same. Or if Z is purely resistive both would read the same. +1 for the question and the answer. I've deleted my answer. \$\endgroup\$
    – Transistor
    Commented Dec 4, 2019 at 13:59
  • \$\begingroup\$ Along with the deleted answer, my thank you went away. But that one deserved to stay. So, thank you again for the hours of support \$\endgroup\$
    – Lucas BS
    Commented Dec 4, 2019 at 15:52
  • \$\begingroup\$ No problem. Don't forget you can accept your own answer to indicate the problem is solved. \$\endgroup\$
    – Transistor
    Commented Dec 4, 2019 at 16:47

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.