Inrush and steady state current in an Inductor with AC voltage

Question

I have two statements here from two great men.

One says

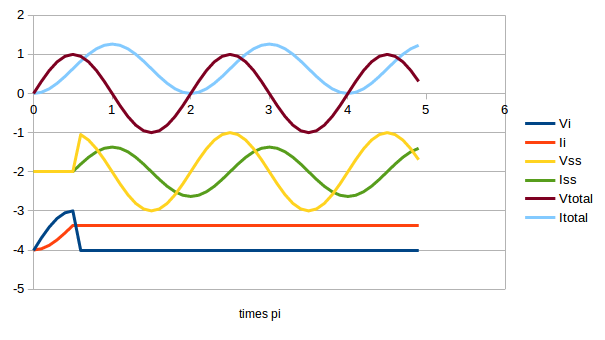
"If you apply AC voltage to the solenoid at the peak of the waveform then there is little or no inrush current whereas if the solenoid is connected to an AC source as it passes through zero volts then the current waveform in the solenoid can reach an excessive value that causes magnetic saturation of the solenoid core and make the problem of inrush even worst"

other one says

"The transient depends upon the contact timing referred to the AC voltage applied, if the contact happens at any AC voltage peak, there is no transient at all (the current becomes a 90 degrees delayed wave straight away) while the transient will be maximized when the contact is achieved at any AC voltage zero crossing, the superimposed current will looks like an asymmetrical Gauss bell (very steeply at rising side)."

My question is why there is transient current or inrush current when a ac source is connected to an inductor at ac voltage zero crossing and why is there is no transient at ac peak voltage ?

Answer 1

If we plot the current that flows in an inductor, as a result of applying a voltage, we get the following.

This was prepared in response to somebody asking about inductor current, driven from a sinuosoidal source. The graph has been divided into several sections. The top traces plot the current from the zero crossing of the sinewave. The second traces plot the current from the peak of the sinewave. The difference between the two plots is shown below.

It's best to concentrate on the yellow/green traces first. Yellow is the sine voltage, but being applied at peak volts. The green current trace grows quickly while the voltage is high, flattens off as the voltage passes through zero, drops while the voltage is negative, and passes back through zero again as the voltage gets to its peak. This is the 'steady state' response. If the circuit had some loss in it, then any starting condition would eventually settle down to this current waveform, symmetrical about zero, and having zero average.

If we switch on at the voltage zero crossing, then we get the dark blue and orange curves. During the first quarter cycle of voltage, the current builds up. It will continue to build during the second quarter cycle.

We can sum these two parts together to get the overall voltage and current waveforms if we switch on at zero crossing, the purple and light blue ones. The current builds to a peak, and returns eventually to zero. The current never goes negative.

In a low permeability solenoid, so air-cored or with big air-gaps, this would not matter, except for the extra heating the higher current produced. However, if the solenoid is cored, and designed for a maximum current just above the peak of the steady state (green) current, then we can see that the peak light blue current exceeds this by a factor of two. Doubling the current in a saturable inductor collapses the inductance, maybe by two or three orders of magnitude, so the current now rises two or three orders of magnitude faster. This is the inrush.