0
\$\begingroup\$

enter image description here

My textbook has the following example of hypothetical linear motor.
A current carrying conductor is placed in a magnetic field.
The conductor is attached to a variable load(pulley+weight).

How it works:
The force of variable load(pulley+weight) is toward right.
The magnetic force (q\$v \times B\$) is toward left.

When there is no load, the conductor accelerates toward left; the back emf grows; when the back emf reaches the supply voltage, current becomes 0, acceleration becomes 0; then the conductor moves at this maximum constant speed.

When the load is increased, the conductor decelerates, back emf decreases, current rises, the magnetic force increases. When this magnetic force balances the load, the deceleration stops; then the conductor moves at this reduced constant speed.

Good so far.

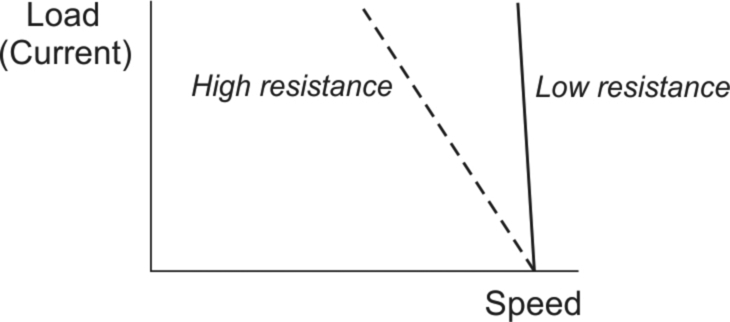
Question: My textbook says that when the resistance of conductor is 0, the speed remains constant at maximum speed no matter what the load is. I don't get this. How can speed not change with load? When we increase the load, surely the back emf decreases. This means the flux linkage is less; this means the speed of the conductor is decreased. I don't see how resistance of conductor plays a role here. Help?

enter image description here

\$\endgroup\$

1 Answer 1

Reset to default
2
\$\begingroup\$

How can speed not change with load?

If Coil R = 0 and mechanical load increases, so does electrical current in order to generate the Torque to maintain the same speed. Motors have a constant V/RPM ratio for no load. (or kV/RPM)

Yet conductors have losses so speed loss depends on the current loop resistance. (unless cryogenically cooled)

\$\endgroup\$
3
  • \$\begingroup\$ Any followup questions? \$\endgroup\$
    – D.A.S.
    Commented Dec 30, 2021 at 3:10
  • 1
    \$\begingroup\$ Ahh I get it now thanks to you :) \$I = \dfrac{V-E}{R}\$. If \$R\$ is small enough, a large change in load/current can be balanced by a small change in \$V-E\$ \$\endgroup\$
    – across
    Commented Dec 30, 2021 at 3:12
  • \$\begingroup\$ By small change in \$V-E\$, I mean when \$V-E\$ is very small, a multiple of it: \$a*(V-E)\$ is also very small. Thanks again I totally get it XD \$\endgroup\$
    – across
    Commented Dec 30, 2021 at 3:26

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.