How to measure AC resistance of an inductor?

Question

Say I found an toroid inductor lying around, what is the method of estimating its AC resistance? DC resistance is easy to know, so is its inductance. Is it essential that I have information about the wire?

Answer 1

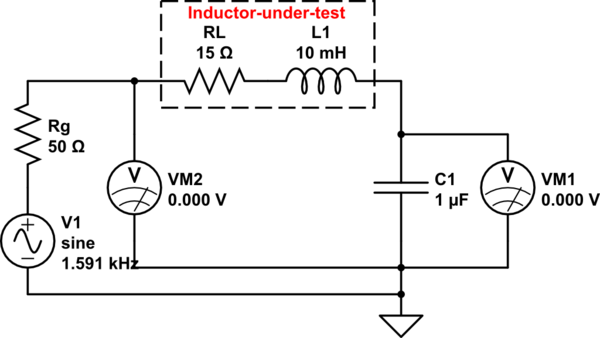
AC resistance measurement might be done with the aid of a good-quality capacitor in series with the inductor. Choose a capacitor value so that resonance with the inductor-under-test occurs at a frequency close to the frequency where you intend to apply the inductor. Since capacitors generally have higher quality (Q) than inductors, the effect of the capacitor's internal resistance might be ignored. But do try to avoid using polarized capacitors.

The measurement proceeds by exciting the series circuit with a function generator at the series-resonant frequency. One must know the generator's internal resistance (often 50 ohms).
One might also use a variac transformer to excite the series circuit, so long as the capacitor (or some series-parallel capacitor combination) has been chosen to resonate with the inductor-under-test at the line frequency (50/60 Hz).

One can monitor the circuit two ways:

• a current monitor connected in series
• a voltage monitor across the inductor OR capacitor. Voltmeter should have high internal resistance.

Current maximizes at resonance. Total series resistance is \$R_G\$ (generator's internal resistance) plus \$R_L\$ (internal resistance of inductor) plus \$R_M\$ (current monitor series resistance).

Voltage across the inductor or voltage across the capacitor maximizes at resonance, and can reach dangerously-high voltages if capacitor + inductor + function generator have low internal resistance.
If one uses a function generator, you might want to monitor two voltages

• function generator output voltage (VM2)
• voltage across the capacitor (VM1)

^{simulate this circuit – Schematic created using CircuitLab}

\$ R_L = R_G \times {{VM2}\over{V1-VM2}}\$ at resonance. \$V1\$ is open-circuit generator voltage

\$ R_L = {{VM2}\over{VM1}} \times {{1}\over{2\pi{f_R}C}}\$ where \$f_R\$ is series-resonant frequency
In the example above, resonance occurs near 1591 Hz. With \$V_G\$ of 1V, VM1=1.542V and VM2=0.23094V
The two-voltmeter method doesn't require knowing the exciting-source internal resistance \$R_G\$.
This method should be limited to amplitudes where the inductor operates linearly. If an oscilloscope is used to measure voltages, rather high test-frequencies can be used, but wire-dressing to the generator should be short.

Answer 2

You need to inject a sinusoidal voltage into your toroidal inductor an get plots (in tabulated data form) of the resulting voltage and current curves across and through the inductor.

From there you use the tabulated data of those curves to calculate the real and reactive power. You can then use that to find the impedance which will be a complex number. The real component of that complex number is the DC+AC resistance lumped together that is dissipating real power inside your inductor.

Then you take the DC resistance that you measured separately and subtract it from the real component of the impedance. That gives you the frequency dependent resistance.

That means you need a signal source, an oscilloscope, and a current probe.

Answer 3

'AC resistance' if you mean the parameter that causes losses that is not solely the DC resistance is quite difficult to measure.

In addition, if parts of it are caused by eddy currents (in the inductor itself, or in nearby conductive paths), it is very frequency dependent.

If it has a ferrite core, the AC resistance depends on the amplitude of the applied signal as this affects inductor saturation and B-H hysteresis.

A practical way is to drive the inductor with a square wave from a DCDC power stage or similar; measure the input current; square wave amplitude, output voltage and current, calculate the inductor losses and assign the 'AC resistance' to the inductor losses.

This requires care and accurate measurements because the losses are relatively small (compared to the lossless power flowing in the inductor), so small errors in the measurements lead to large errors in the result.

Answer 4

Inductors are typically by far the most imperfect of the L-C-R trinity.

The inductance will usually change (sometimes drastically) with the current. If it's air core it will not saturate, but the inductance may change significantly based on what is near it. The DC resistance will change with temperature (and it's sometimes quite significant).

In addition there is distributed capacitance (often quite significant at high frequencies as you approach the self-resonant frequency), there are core losses (which, of course, vary with frequency and temperature), and skin effect.

For some idea of how these parameters can be measured you can refer to this Keysight document, including the section on applying a DC bias to inductors. Ideally you'll have a spare few tens of thousands of dollars to buy the professional equipment. If not, it's possible to rig up tests for specific operating conditions, assuming you have some idea of what those might be.

Answer 5

ac resistance \$R_{ac}\$ can not be measured directly. It depends upon:

• Eddy current losses.
• Hysteresis loss in iron core.
• Radiation losses.
• Skin effect.

These are frequency dependent and increase with frequency.

You can get \$R_{DC}\$ with a meter.

Random inductor so inductance may be unknown. But many meters have Capacitance measuring ability. Use resonance and a measurable capacitor to determine inductance.

Connect a small resistor in series with the inductor, a capacitor and a funcion generator. Measure \$V_R\$ vs \$V_S\$ with a scope. \$V_R\$ will be in phase with ac current, so scope is indirectly measuring I vs \$V_S\$.

Same thing can be done by trying to get the capacitance voltage equal to inductance voltage but effective resistance throws off inductance voltage.

Apply a voltage and vary the frequency until \$V_R\$ is in phase with \$V_S\$. This is resonant frequency. Measure C and use resonant frequency to determine L.

$$f_R = \frac {1}{2 \pi \sqrt {LC}}$$

I'd select another scale capacitor and repeat measurements to verify L calculation.

\$R_{ac}\$ is frequency dependent.

Circuit is function generator and inductor. Adjust function generator to target frequency. Apply a voltage and measure current. Calculate impedance.

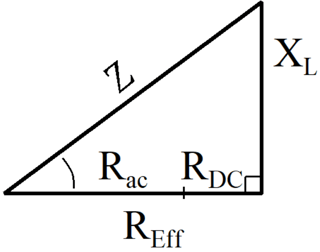
Calculate Inductive Reactance \$X_L\$. Use Pythagoreaus to determine the effective resistance \$R_{Eff}\$ of the inductor.

$$Z = \sqrt {R_{Eff}^2 + X_L^2}$$

Subtract \$R_{DC}\$ and you get \$R_{ac}\$.

I'd also repeat at a lower and higher frequency to understand impact frequency has on ac resistance.

Answer 6

Resistance is a DC characteristic of an inductor (ESR or Equivalent Series Resistance). Inductance is the AC equivalent of resistance).

ESR can be estimated using the length of wire and wire gauge.

Inductance is dependent on lots of things (coil diameter, turns count, turns spacing, core reluctance, core saturation, etc) and weakly dependent on wire diameter.