0
\$\begingroup\$

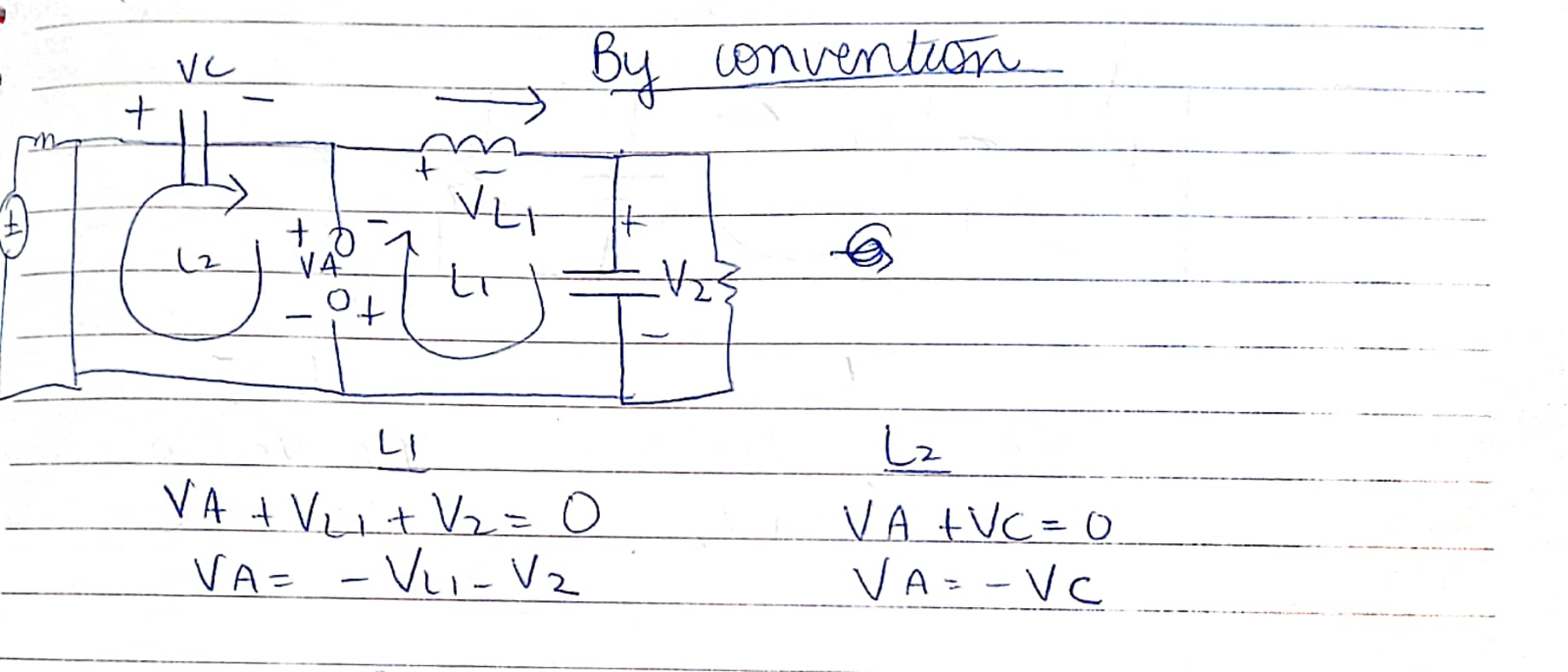
I'm a bit confused about using KVL on circuits. For example, suppose I have this circuit, and we are given the direction of inductors current and capacitors current, and we have to find VA. Using the convention, I got the following:

enter image description here

The solution is that VA is negative, so this is right.

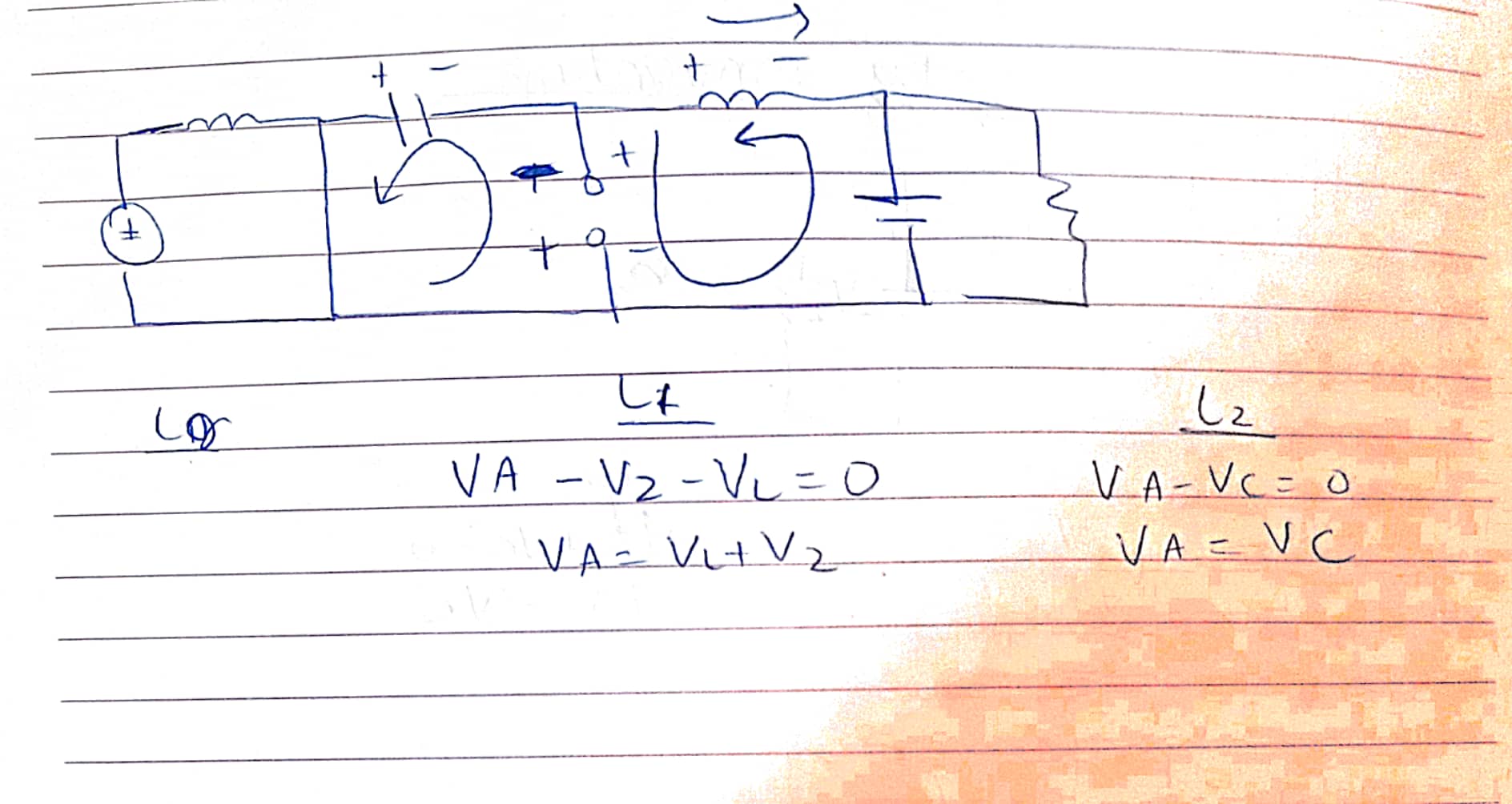
But if I change the polarity of VA and solve according, I get:

enter image description here

VA isn't positive as indicated in the solution, but I'm getting positive.
Am I doing something wrong?

\$\endgroup\$
1
  • \$\begingroup\$ You don't get to change the sign of \$V_A\$. You need to pick a setting for it and then consistently apply that choice for both loop equations. Otherwise you are changing its meaning in the middle of the analysis. And that's not good to do. You did keep the signs for everything else. So why did you change this one? \$\endgroup\$
    – periblepsis
    Commented Sep 27, 2023 at 9:24

1 Answer 1

Reset to default
1
\$\begingroup\$

You've proven the same thing in two ways. That's all.

In your first analysis you proved that the most positive terminal of \$V_A\$ was connected to the lowest node on your circuit. In your 2nd analysis you proved this again. I see no problem here.

\$\endgroup\$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.