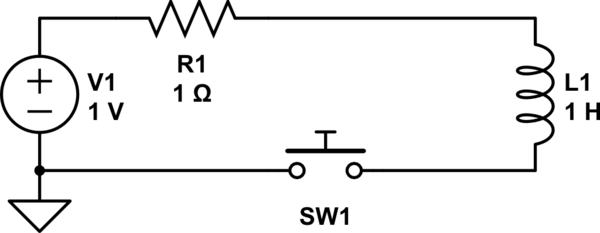
When the series current is zero, the voltage across L1 must equal V1 (by KVL and Ohm's Law).
But, for an (ideal) inductor, we have:
\$v_L = L \dfrac{di_L}{dt}\$
Thus, by KVL and the definition of an ideal inductor, at the moment SW1 closes, the time rate of change of current is:
\$\dfrac{di_L}{dt} = \dfrac{1V}{1H} = 1 \dfrac{A}{sec} \$
So, the crucial insight here is this: there is no current at the moment the switch closes but, at that very moment, the current begins to change.
How do I calculate the rate of change slightly after the first
after-SW1-closed rate if R1 is to be taken into consideration?
By solving the differential equation that describes the circuit. By KVL and Ohm's Law, we have:
\$v_L = L \dfrac{di_L}{dt} = v_1 - i_L R \rightarrow \dfrac{di_L}{dt} + \dfrac{R}{L}i_L = v_1\$
This is an easy 1st order ordinary differential equation for the series current \$i_L\$.
The solution, for zero initial condition, is:
\$i_L(t) = \dfrac{v_1}{R}(1 - e^{-\frac{t}{\tau}}) \$
Where
\$\tau = \dfrac{L}{R} \$
When t is "small enough", i.e., right after the switch closes, we have:
\$i_L(t) \approx \dfrac{v_1}{L}t \$
So, in the early moments, the resistance has negligible effect and the current is approximately a ramp. The current begins to significantly deviate from a ramp only after the current becomes large enough such that the voltage drop across the resistor is significant compared to the voltage source.